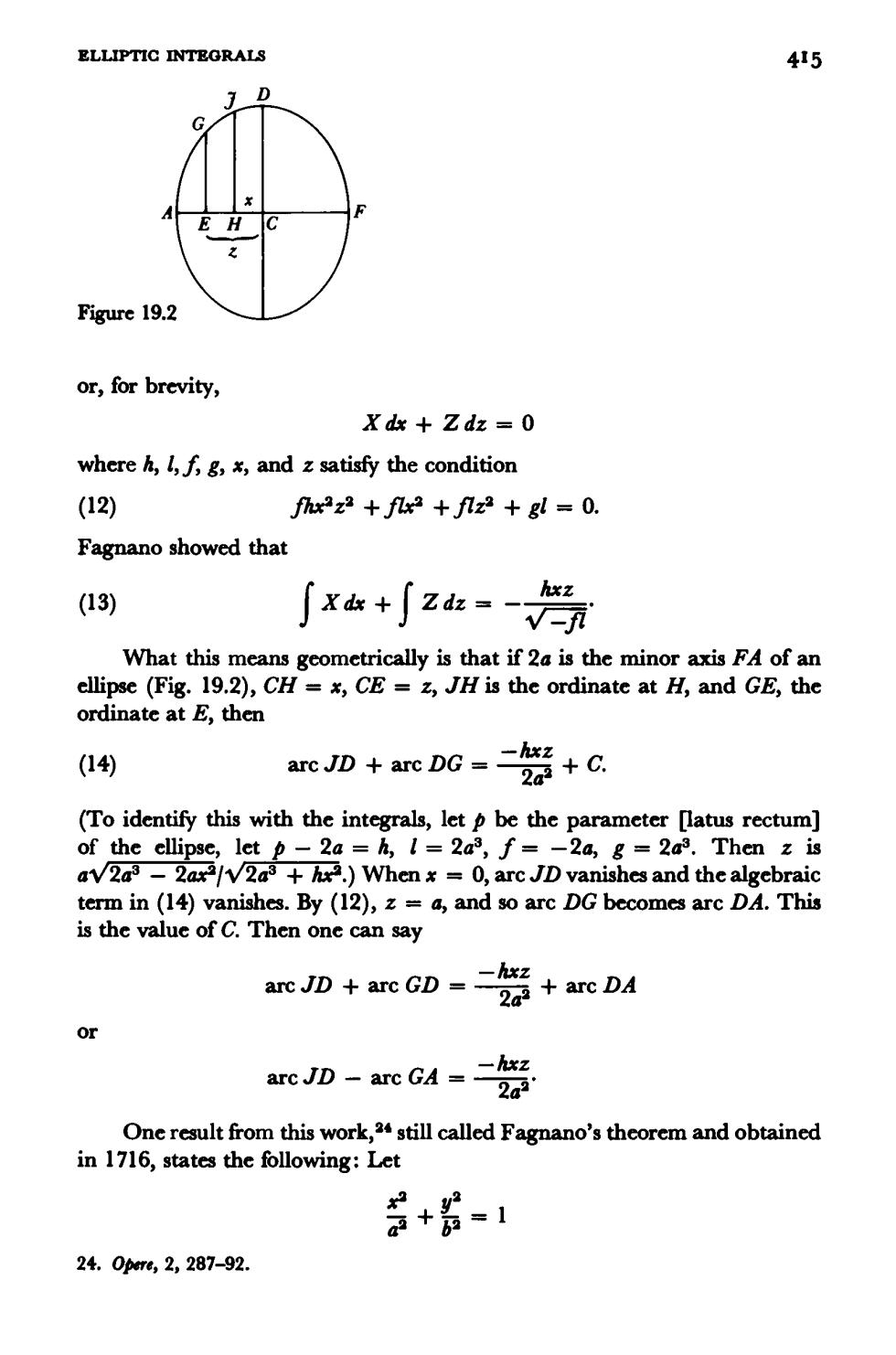
ELLIPTIC INTEGRALS 415 D 无 Figure 19.2 or,for brevity, Xdx+Zdz=0 where h,l,f,g,*and z satisfy the condition (12) fhx2z2+flx2+flz2+gl =0. Fagnano showed that (13) x出+∫z红=券 What this means geometrically is that if is the minor axis FA of an ellipse(Fig.l9.2),CH=¥,CE=名,JH is the ordinate at H,and GE,the ordinate at E,then (14) arcJD+arc DG=一2+C (To identify this with the integrals,let be the parameter [atus rectum] of the cllipsc,let p-2a=h,I=2a3,f=-2a,g 2a3.Then z is av2a3 -2ax/V2a3+hx.)When x =0,arc JD vanishes and the algebraic term in (14)vanishes.By (12),z=a,and so arc DG becomes arc DA.This is the valuc of C.Then one can say eD+acG0-祭+aeDA areJD-a GA=二 One result from this work,still called Fagnano's theorem and obtained in 1716,states the following:Let 茶+5-1 24.0pr%,2,287-92
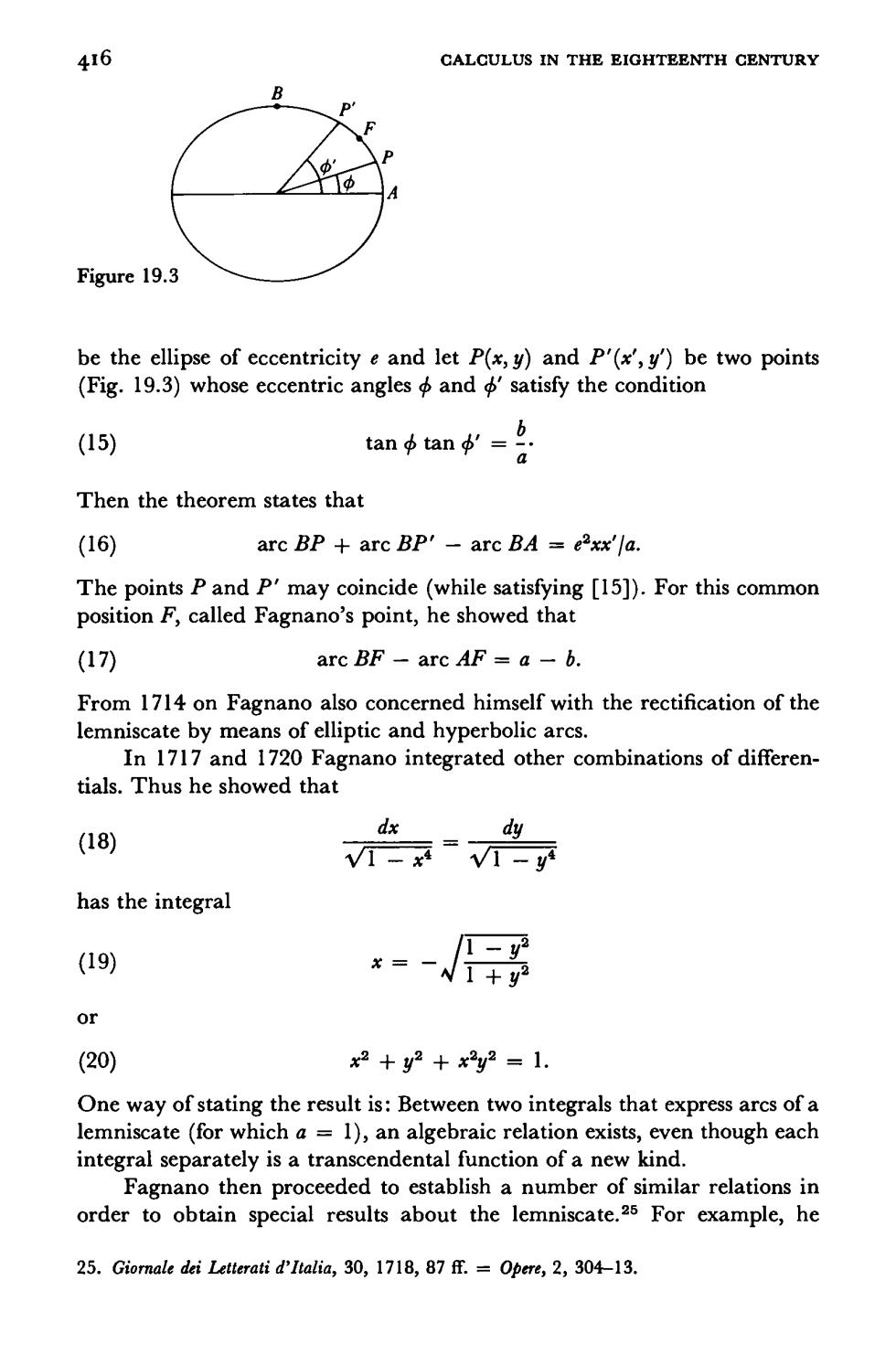
416 CALCULUS IN THE EIGHTEENTH CENTURY Figure 19.3 be the ellipse of eccentricity e and let P(x,y)and P'(x',y')be two points (Fig.19.3)whose eccentric angles$andsatisfy the condition (15) an中an$-合 Then the theorem states that (16) arc BP arc BP'-arc BA =e2xx'la. The points P and P'may coincide (while satisfying [15]).For this common position F,called Fagnano's point,he showed that (17) arc BF-arc AF a-b. From 1714 on Fagnano also concerned himself with the rectification of the lemniscate by means of elliptic and hyperbolic arcs In 1717 and 1720 Fagnano integrated other combinations of differen- tials.Thus he showed that dx (18) dy -京=可 has the integral (19) or (20) x2+2+x2y2=1. One way of stating the result is:Between two integrals that express arcs of a lemniscate (for which a =1),an algebraic relation exists,even though each integral separately is a transcendental function of a new kind. Fagnano then proceeded to establish a number of similar relations in order to obtain special results about the lemniscate.For example,he 25.Giormale dei Letterati d'ltalia,30,1718,87ff.=Opere,2,304-13

ELLIPTIC INTEGRALS 4r7 Figure 19.4 showed that if (21) dx then (22) 后= or by solving for x, (23) -1+2y2+y 1+2y-4 From a variety of such results Fagnano showed how to find the points on the lemniscate(the values ofrinr2=acos 20)that divide the quadrant,that is, the arc CQA in Figure 19.4,into n equal parts for certain values of n.He also showed how,given an arc CS,one can find the point I on this arc that divides CS into two equal parts.Further,he found the points on arc CQA which,when joined to C,divide the area under Cod and the horizontal axis into two,three,and five parts;and given the chords that divide this area into n equal parts,he found the chords bisecting each of these parts. Thus Fagnano had done more than answer Bernoulli's question;he had shown that the same remarkable algebraic property that characterized the integrals representing logarithmic and inverse trigonometric functions heldfor at least certain classesof elliptic integrals. About 1750 Euler noted Fagnano's work on the ellipse,hyperbola,and lemniscate and began a series of investigations of his own. de Comparatione Arcuum Curvarum Irrectificabiliu Euler,after repeating some of Fagnano's work,showed how to divide the area of a quadrant of the lemniscate into n+1 parts if it has already been divided into n parts.He then points out that his and Fagnano's work has furnished some useful results on integration,for the cquation (18)has, besides the obvious integral x=y,the additional particular integral x=-V(1-y211+3. 26.Novi Comm.4Acad.S.Peop,6,1756/7,58-84,pub.1761=0pera,(1),20,80-107

418 CALCULUS IN THE EIGHTEENTH CENTURY In his paper"De Integratione Equationis Differentialis m dx/VI -ndy/vI,"7 Euler takes Fagnano's results as his point of departure The integrals Fagnano had obtained for most of the diferential cquations he had considered were particular integrals.These were algebraic;but the complete integral might well be transcendental.Euler decided to look for complete integrals in algebraic form.He started with (18),but hoped to obtain the complete integral of (24) m dx ndy Here m/n is rational and the equation expresses the problem of finding two arcs of a lemniscate that have this ratio to each other.Euler says that he has been led by trial to believe that(24)has a complete integral that can be exprcssed algebraically when m/n is rational From the investigations of Fagnano it followed that equation (18)is satisfied by the particular integral(19)or(20).The integral of each side of (18)is an arc of a lemniscate with half-axis 1 and abscissa *and the integra- tion of the ordinary differential equation (18)amounts to finding two arcs that are ofcqual length.Euler had pointed out that*=yis another particular integral of(18).The complete integral should be such that it reduces to each of these particular integrals for special values of the arbitrary constant. Guided by these facts,Euler found that the complete integral of(18)is (25) y+y=c+2x1-c (26) x=-6±W- 1+cy2 where c is an arbitrary constant.Of course,given(25)we can readily verify that it is the complete integral of(18). Implicit in the result(25)is what is often called Euler's addition theorem for these simple elliptic integrals.It is clear by straight differentiation that (27) f*dx where c is a constant,is also a complete integral of(18).Hence x,y,and c must be related by (25).Thus the addition theorem says that if(27)holds for the elliptic integrals therein,then the upper limit is an algebraic symmetric function,namely (26),of the arbitrarily chosen upper limits y and c of the other two integrals.The addition theorem applics to more general integrals,as we shall see. 27.Novi Comm.Acad.Si.Peop.,6,1756/7,37-57,pub.1761=0pra,(1),20,58-79

ELLIPTIC INTEGRALS 419 By utilizing these results(25)and (27),it is rather straightforward to show that if (28) wv theny is an algebraic function of x.This result is called Euler's multiplication theorem for the clliptic integraldx/vI-x.From this result the com- plete integral of equation(28)follows;the important point is that it is an algebraic equation in *y,and an arbitrary constant c.Euler shows how the complete integral might be obtained but docs not give it explicitly. In the same paper of 1756-57 and in Volume 7 of the same journal, Euler tackled more general elliptic integrals.He gives the following result, which he says he obtained by trial and error.If one differentiates (29)+2(x+)+y(x2+y2)+2g+2y(x+)+y2=0, then the differential cquation can be put in the form (30) 爱+=0 whereand Yare polynomials of the fourth degree of which four cocients (the same inand y)can be expressed in terms of the five in (29)with the help of an arbitrary constant.Then (29)is the complete integral of(30), and when (30)is specialized to (18),then (29)becomes (25).Euler remarks that it is wonderful that,even though an integral of dx/vX cannot be obtained in terms of circular or logarithmic functions,the equation(30)is satisfied by an algebraic relation.He then generalizes the results to (31) m/n rational, whcre and Y are fourth degree polynomials with the same coefficients The result is also in Euler's Institutiones Calculi Integralis,20 where he shows what the results mean geometrically in terms of ellipse,hyperbola,and lemniscate. From these results Euler was able to proceed with what is now called the addition theorem for elliptic integrals of the first kind.Consider the elliptic integral (32) VR() 28.N60 iComm.4cad.S.Pep,7,1758/9,348,pub.1761=0pra,(1),20,153-200. 29.Vol.1,Scc.2,Chap.6=0pr4,(1),11,391-423