
2.2 Gaussian Matrices We can degenerate it into i.i.d case that each entry of xecTR is independent random variable with zero-mean and unit variance whose covariance matrix Xx"]=I and X"X]=I its joint PDF can be expressed as 人-点e and then we can degenerate it into a Gaussian vector xeC,its PDF: 人-e 6
6 2.2 Gaussian Matrices We can degenerate it into i.i.d case that each entry of is independent random variable with zero-mean and unit variance whose covariance matrix and , its joint PDF can be expressed as H XX I H X X I H 1 ( ) ( ) tr mn f e Z Z X Z m n X and then we can degenerate it into a Gaussian vector , its PDF: m x 1 H ( ) m f e z z x z
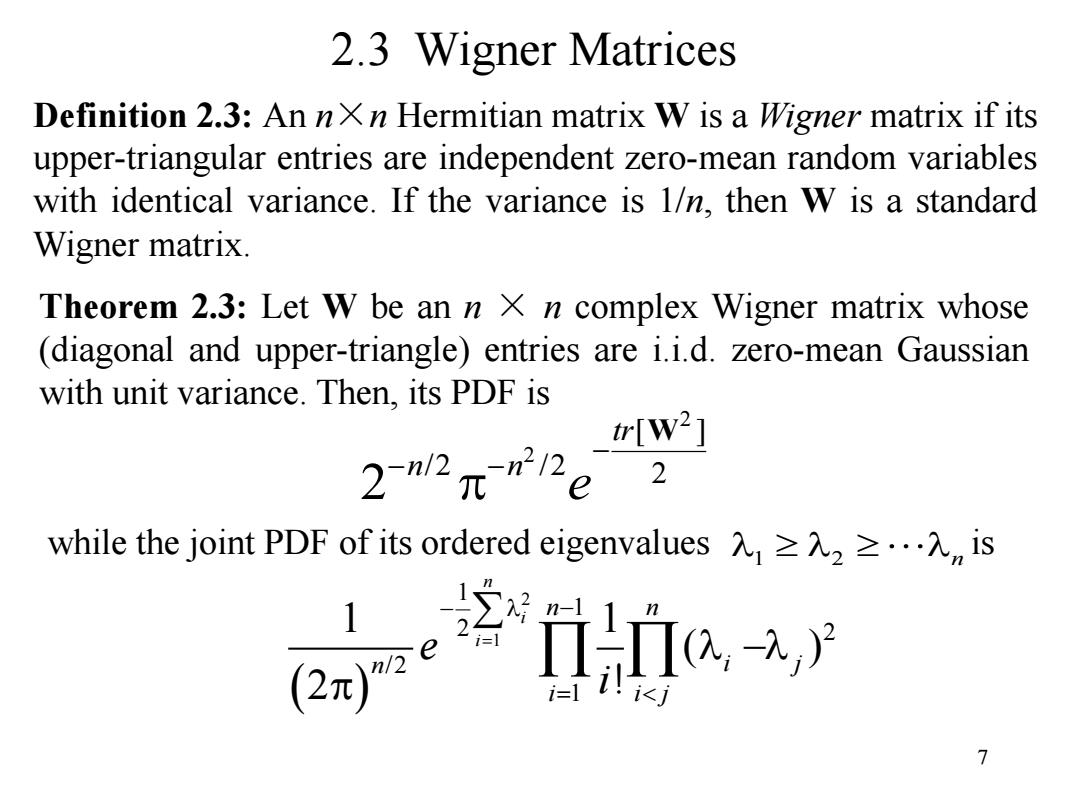
2.3 Wigner Matrices Definition 2.3:An nXn Hermitian matrix W is a Wigner matrix if its upper-triangular entries are independent zero-mean random variables with identical variance.If the variance is 1/n,then w is a standard Wigner matrix. Theorem 2.3:Let W be an n x n complex Wigner matrix whose (diagonal and upper-triangle)entries are i.i.d.zero-mean Gaussian with unit variance.Then,its PDF is rIw2] 2m2元n2e 2 while the joint PDF of its ordered eigenvalues,≥入2≥…入nis 2 i<i 7
7 2.3 Wigner Matrices Definition 2.3: An n×n Hermitian matrix W is a Wigner matrix if its upper-triangular entries are independent zero-mean random variables with identical variance. If the variance is 1/n, then W is a standard Wigner matrix. Theorem 2.3: Let W be an n × n complex Wigner matrix whose (diagonal and upper-triangle) entries are i.i.d. zero-mean Gaussian with unit variance. Then, its PDF is 2 2 [ ] /2 /2 2 2 tr n n e W while the joint PDF of its ordered eigenvalues is 1 2 n 2 1 1 1 2 2 / 2 1 1 1 ( ) 2 ! n i i n n n i j i i j e i

2.4 Wishart Matrices Definition 2.4:If the m x n Gaussian matrix H whose expectation matrix is M and covariance matrix is >then the m X m random matrix A= H,m”is a Wishart matrix,ie.A~Wmn,M,)orn≥m. H'H,m≥n Remark:For a Wishart matrix A=HH',if M=0,we call A is a central Wishart matrix. The PDF of the central complex Wishart matrix A~W,(n,0,>)for n=m iS 元-mm-l)/2 X)= e-txl det xn-m detΣ"Π,(n-i)! 8
8 2.4 Wishart Matrices Definition 2.4: If the m × n Gaussian matrix H whose expectation matrix is M and covariance matrix is Σ, then the m × m random matrix is a Wishart matrix, i.e. A∼Wm (n,M,Σ) for . 1 1)/2 tr[ ] 1 ( ) det det ( )! m(m n-m n m i f e n - i Σ X A X X Σ Remark: For a Wishart matrix A=HH† , if M=0, we call A is a central Wishart matrix. † † < = m n m n HH A H H , , The PDF of the central complex Wishart matrix A∼Wm (n,0,Σ) for n ≥ m is n m