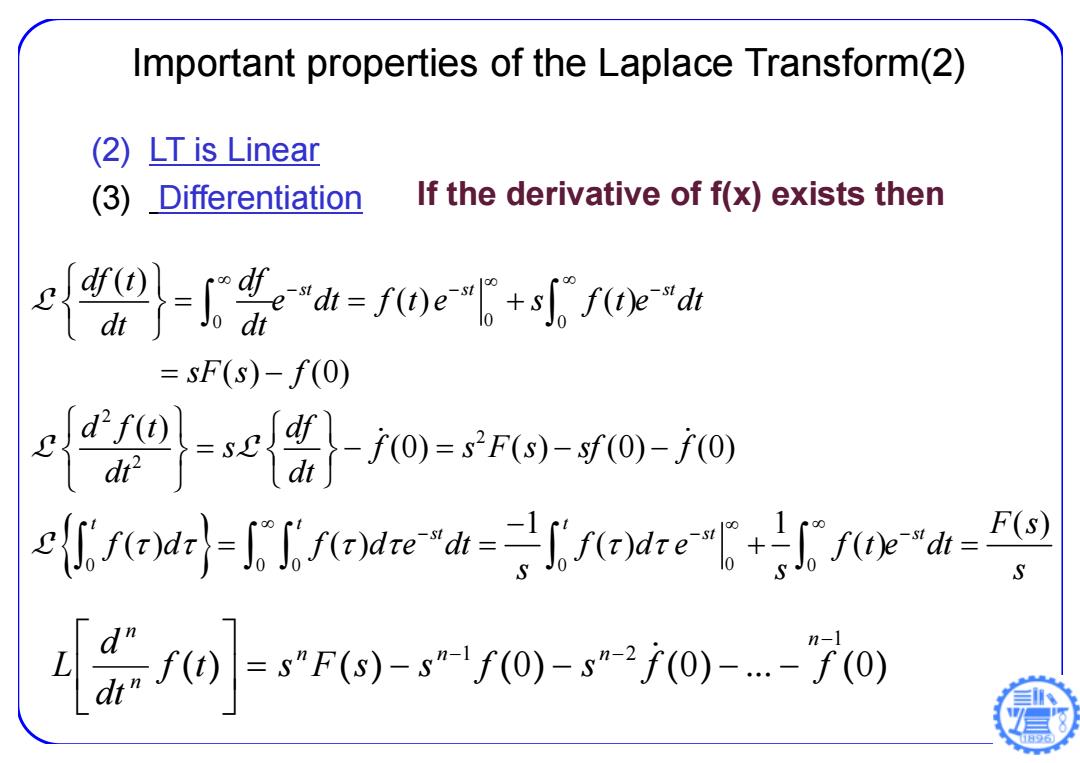
Important properties of the Laplace Transform(2) (2) LT is Linear (3) Differentiation If the derivative of f(x)exists then \离=foef+eh =sF(S)-f(0) 9到=心离0=0-0 (ddread 小蛋0o-0r7-70
Important properties of the Laplace Transform(2) (2) LT is Linear (3) Differentiation ( ) ( ) ( 0 ) ( 0 ) ... ( 0 ) 1 1 2 n n n n n n f t s F s s f s f f dt d L If the derivative of f(x) exists then 0 0 0 2 2 2 0 00 0 0 0 ( ) () () ( ) (0) ( ) (0) ( ) (0) (0) 1 1 ( ) ( ) ( ) () st st st tt t st st st df t df e dt f t e s f t e dt dt dt sF s f d f t df s f s F s sf f dt dt F f d f d e dt f d e f t e dt s s L L L L ( )s s
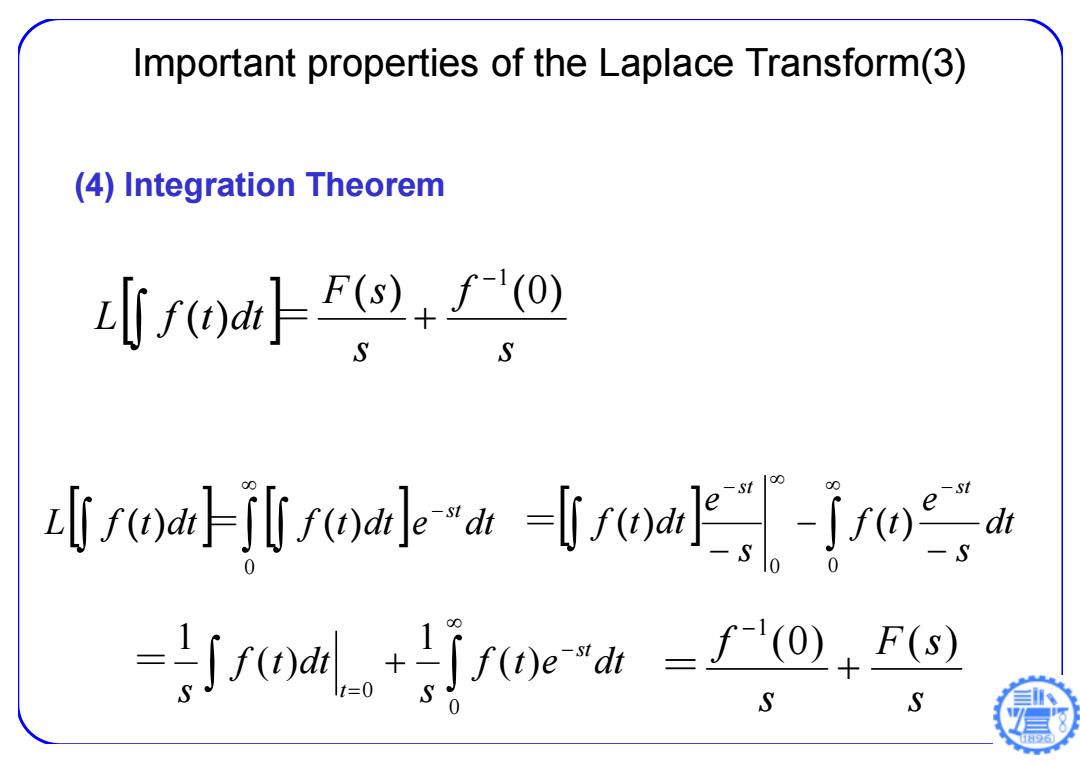
Important properties of the Laplace Transform(3) (4)Integration Theorem ff0a/'o S row-jinolo -S dt -dfe() S S
Important properties of the Laplace Transform(3) (4) Integration Theorem s f s F s L f t dt ( ) ( ) ( ) 0 1 = dt s e f t s e f t dt st st 0 0 L f t dt f t dt e dt = ( ) ( ) st 0 ( ) = ( ) 0 0 ( ) 1 ( ) 1 f t e dt s f t dt s st t = s F s s f ( 0 ) ( ) 1 =
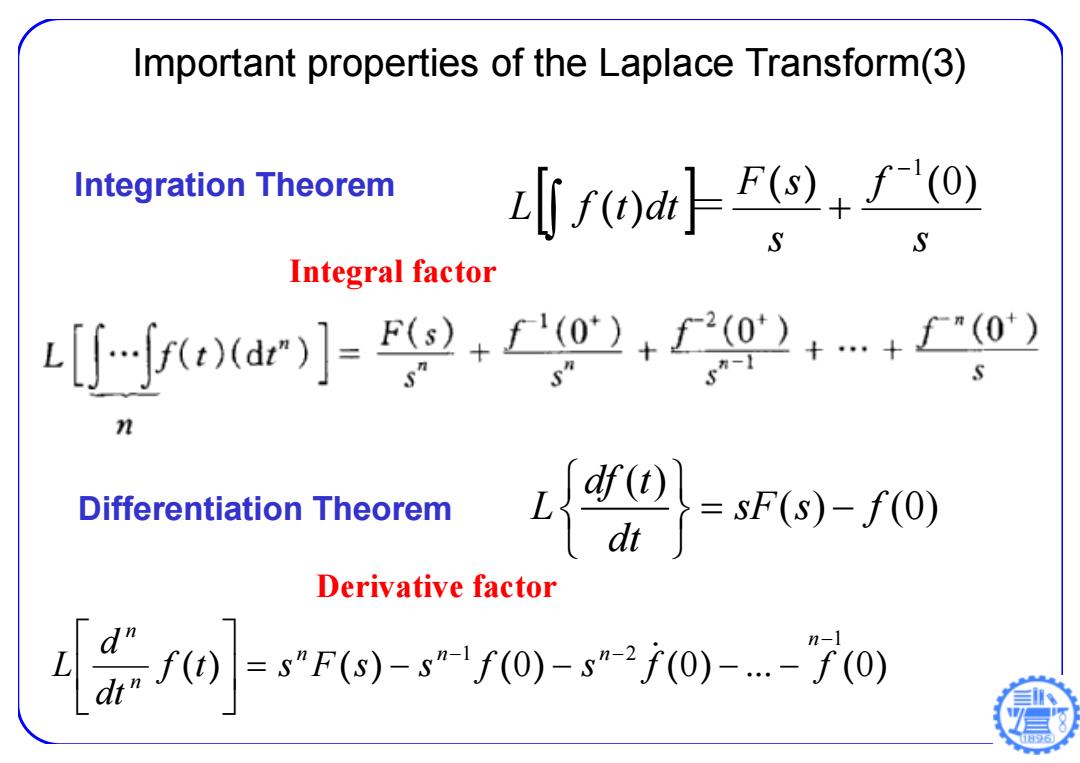
Important properties of the Laplace Transform(3) Integration Theorem rmalF+'@ Integral factor -d]小-+f八o+0+o) Differentiation Theorem t0=a-0, Derivative factor ]=7s70-f0-70
Important properties of the Laplace Transform(3) Integration Theorem s f s F s L f t dt ( ) ( ) ( ) 0 1 = ( ) ( ) (0) df t L sF s f dt Differentiation Theorem Integral factor Derivative factor ( ) ( ) ( 0 ) ( 0 ) ... ( 0 ) 1 1 2 n n n n n n f t s F s s f s f f dt d L
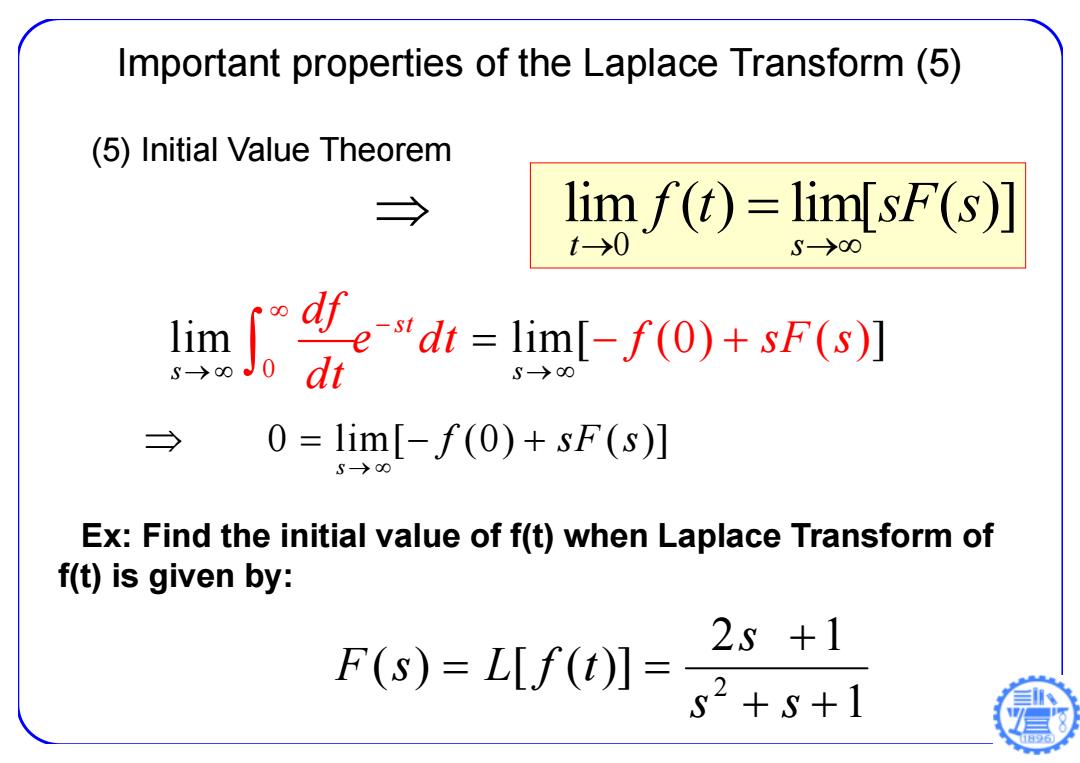
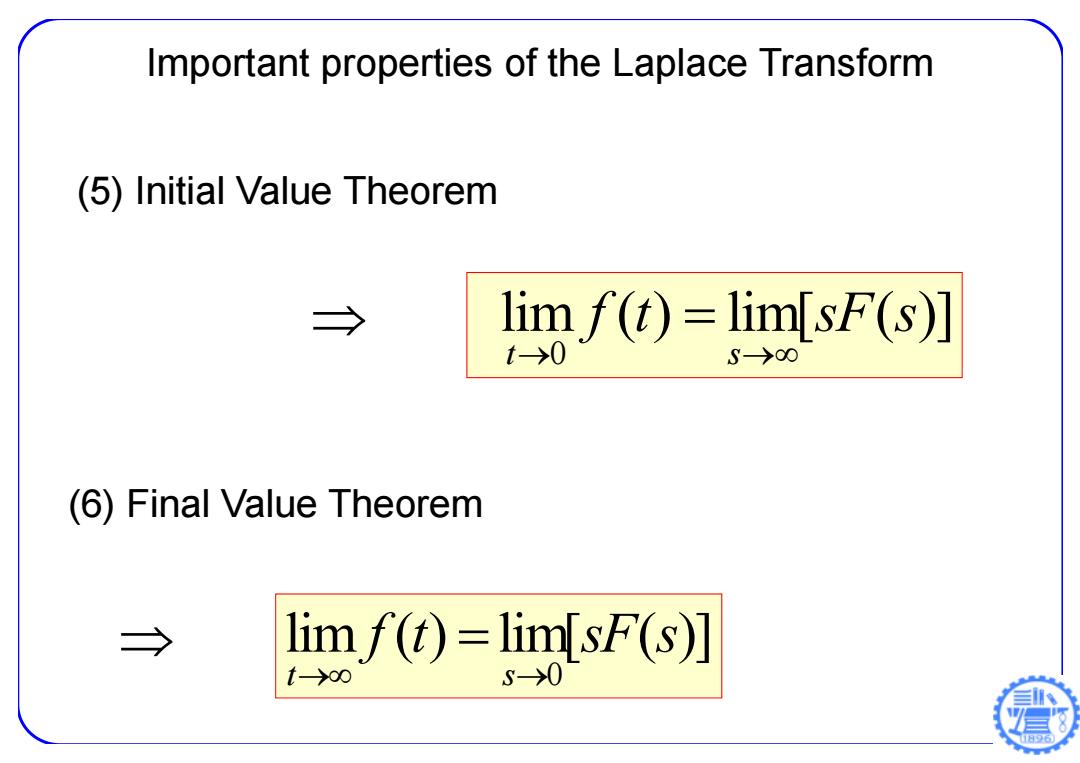
Important properties of the Laplace Transform(5) (5)Initial Value Theorem → lim f(t)=lim[sF(s)] t→0 S→00 lime-"dt=lim[-f(0)+sF(s)] dt S-> → 0=lim[-f(0)+sF(s)] S→00 Ex:Find the initial value of f(t)when Laplace Transform of f(t)is given by: 25+1 F(S)=L[f(t)]= s2+s+1
Important properties of the Laplace Transform (5) (5) Initial Value Theorem 0 lim lim[ () () 0 ] s s df st e dt f sF s dt 0 lim[ (0) ( )] s f sF s 0 lim ( ) lim[ ( )] t s f t sF s Ex: Find the initial value of f(t) when Laplace Transform of f(t) is given by: 1 2 1 ( ) [ ( )] 2 s s s F s L f t
Important properties of the Laplace Transform (5)Initial Value Theorem → lim f(t)=lim[sF(s)] t->0 (6)Final Value Theorem → limf(t)=limsF(s)] s->0
Important properties of the Laplace Transform (6) Final Value Theorem 0 lim ( ) lim[ ( )] t s f t sF s (5) Initial Value Theorem 0 lim ( ) lim[ ( )] t s f t sF s