
Tutorial 9:Further Topics on Random variables 2 Weiwen LIU wwliu@cuhk.edu.hk March 27,2017 1
Tutorial 9: Further Topics on Random Variables 2 Weiwen LIU wwliu@cuhk.edu.hk March 27, 2017 1

Covariance and Correlation Covariance and correlation describe the degree to which two random variables or sets of random variables tend to deviate from their expected values in similar ways. Independent random variables are uncorrelated,but NOT vice versa. 2
Covariance and Correlation • Covariance and correlation describe the degree to which two random variables or sets of random variables tend to deviate from their expected values in similar ways. • Independent random variables are uncorrelated, but NOT vice versa. 2
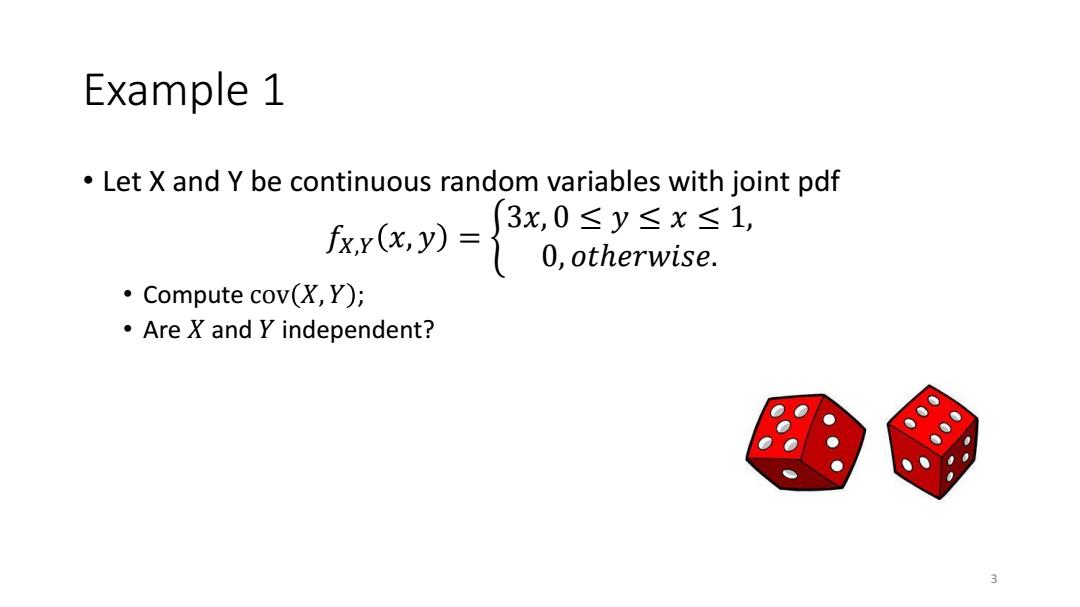
Example 1 Let X and Y be continuous random variables with joint pdf fxr(x,y) 3x,0≤y≤x≤1, 0,otherwise. ·Compute cov(X,Y); Are X and Y independent? 3
Example 1 • Let X and Y be continuous random variables with joint pdf 𝑓𝑋,𝑌 𝑥, 𝑦 = ቊ 3𝑥, 0 ≤ 𝑦 ≤ 𝑥 ≤ 1, 0, 𝑜𝑡ℎ𝑒𝑟𝑤𝑖𝑠𝑒. • Compute cov 𝑋, 𝑌 ; • Are 𝑋 and 𝑌 independent? 3
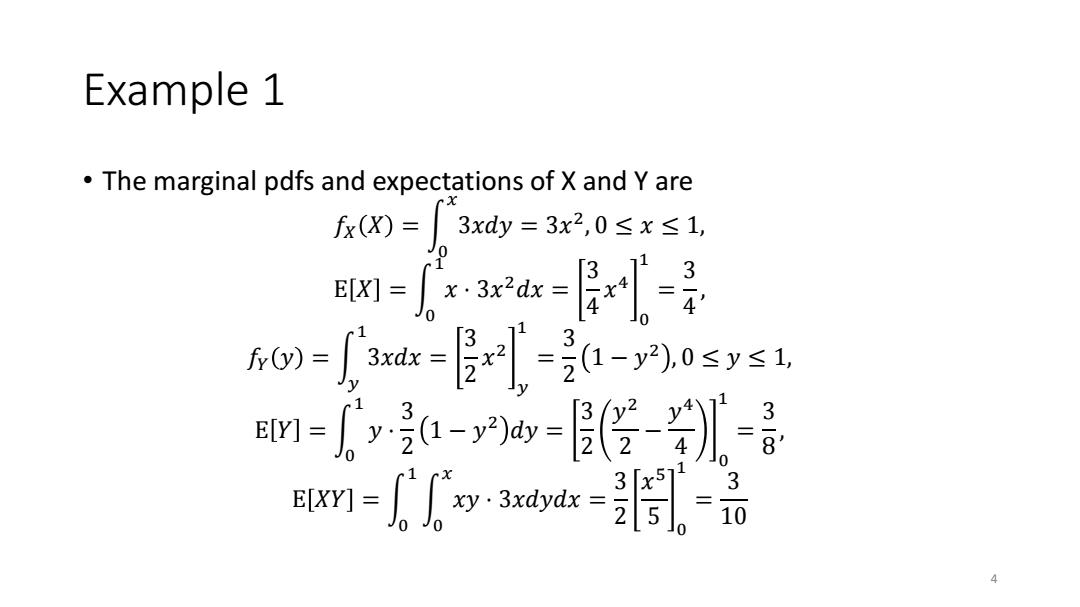
Example 1 The marginal pdfs and expectations of X and Y are fx(X)=3xdy=3x2,0≤x≤1, W=3a-屋=子 1 o)=3a=层x-0-y9.0≤ys1 =-西告 3 m-w3d--品 3 0 4
Example 1 • The marginal pdfs and expectations of X and Y are 𝑓𝑋 𝑋 = න0𝑥 3𝑥𝑑𝑦 = 3 𝑥 2 , 0 ≤ 𝑥 ≤ 1 , E 𝑋 = න0 1 𝑥 ⋅ 3 𝑥 2𝑑𝑥 = 34 𝑥 4 01 = 34 , 𝑓𝑌 𝑦 = න𝑦1 3𝑥𝑑𝑥 = 32 𝑥 2 𝑦1 = 32 1 − 𝑦 2 , 0 ≤ 𝑦 ≤ 1 , E 𝑌 = න0 1 𝑦 ⋅ 32 1 − 𝑦 2 𝑑𝑦 = 32 𝑦 22 − 𝑦 44 01 = 38 , E 𝑋𝑌 = න0 1 න0 𝑥 𝑥𝑦 ⋅ 3𝑥𝑑𝑦𝑑𝑥 = 32 𝑥 55 01 = 3 10 4

Example 1 Then the covariance is 333 3 COv(X,Y)=E[XY]-E[XJE[Y]= 10-4×8= 160 .X and Y are not independent as it is not true that fx.r(x,y)=fx(x)fy(y). 5
Example 1 • Then the covariance is cov X, Y = E XY − E X E Y = 3 10 − 3 4 × 3 8 = 3 160 . • 𝑋 and 𝑌 are not independent as it is not true that 𝑓𝑋,𝑌 𝑥, 𝑦 = 𝑓𝑋 𝑥 𝑓𝑌 𝑦 . 5