
Tutorial 6:General Random Variables 2 Yitong Meng March 6,2016 1
Tutorial 6: General Random Variables 2 Yitong Meng March 6, 2016 1

Outline Extend the notion of PDF to the case of multiple random variables Jointly continuous PDF ·Marginal PDF ·Conditional PDF ·Exercises 2
Outline Extend the notion of PDF to the case of multiple random variables • Jointly continuous PDF • Marginal PDF • Conditional PDF • Exercises 2

Continuous pde Recall that X is continuous if there is a function f(x)(the density) such that PX≤)=fk(x)d We generalize this to two random variables. 3
Continuous PDF • Recall that 𝑋 is continuous if there is a function 𝑓(𝑥) (the density) such that 𝑃(𝑋 ≤ 𝑡) = න −∞ 𝑡 𝑓𝑋 𝑥 𝑑𝑥 We generalize this to two random variables. 3

Jointly continuous PDF ·Definition Two random variables X and Y are jointly continuous if there is a function fx.y(x,y)on R-,called the joint probability density function,such that PX≤sY≤)= fxr(x,y)dxdy x≤S,y≤t
Jointly continuous PDF • Definition Two random variables 𝑋 and 𝑌 are jointly continuous if there is a function 𝑓𝑋,𝑌 𝑥, 𝑦 on 𝑅 2 , called the joint probability density function, such that 𝑃 𝑋 ≤ 𝑠, 𝑌 ≤ 𝑡 = ඵ 𝑥≤𝑠,𝑦≤𝑡 𝑓𝑋,𝑌 𝑥, 𝑦 𝑑𝑥𝑑𝑦 4
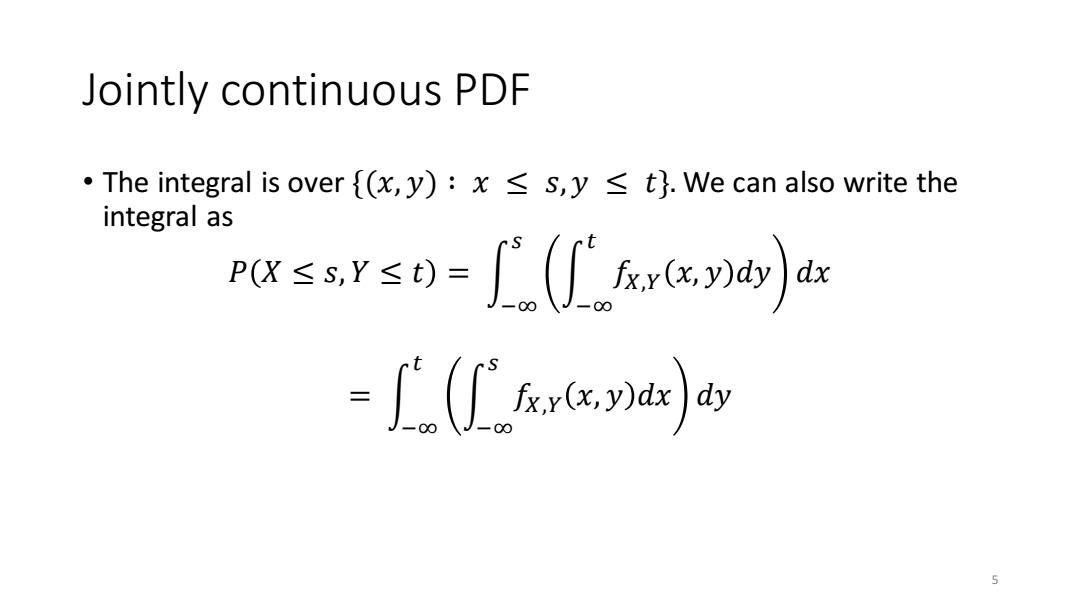
Jointly continuous PDF ·The integral is over{(x,y):x≤s,y≤t}.We can also write the integral as Px≤s,Y≤=(xr(.ydy) =(v.ys)西
Jointly continuous PDF • The integral is over {(𝑥, 𝑦) ∶ 𝑥 ≤ 𝑠, 𝑦 ≤ 𝑡}. We can also write the integral as 𝑃 𝑋 ≤ 𝑠, 𝑌 ≤ 𝑡 = න −∞ 𝑠 න −∞ 𝑡 𝑓𝑋,𝑌 𝑥, 𝑦 𝑑𝑦 𝑑𝑥 = න −∞ 𝑡 න −∞ 𝑠 𝑓𝑋,𝑌 𝑥, 𝑦 𝑑𝑥 𝑑𝑦 5