
Tutorial 7:General Random Variables 3 Yitong Meng March 13,2017 1
Tutorial 7: General Random Variables 3 Yitong Meng March 13, 2017 1
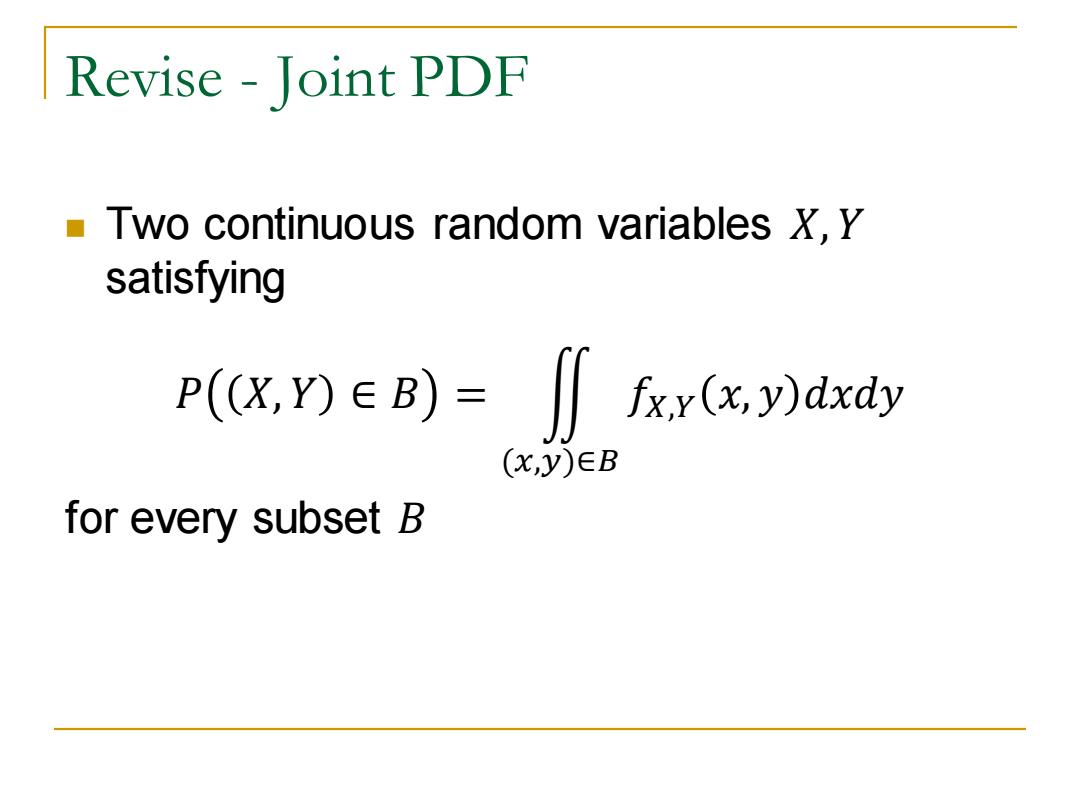
Revise-Joint PDF Two continuous random variables X,Y satisfying P((x.Y)EB)=fr(x.)dxdy (X,y)∈B for every subset B
Revise - Joint PDF ◼ Two continuous random variables 𝑋, 𝑌 satisfying 𝑃 𝑋, 𝑌 ∈ 𝐵 = ඵ (𝑥,𝑦)∈𝐵 𝑓𝑋,𝑌 𝑥, 𝑦 𝑑𝑥𝑑𝑦 for every subset 𝐵
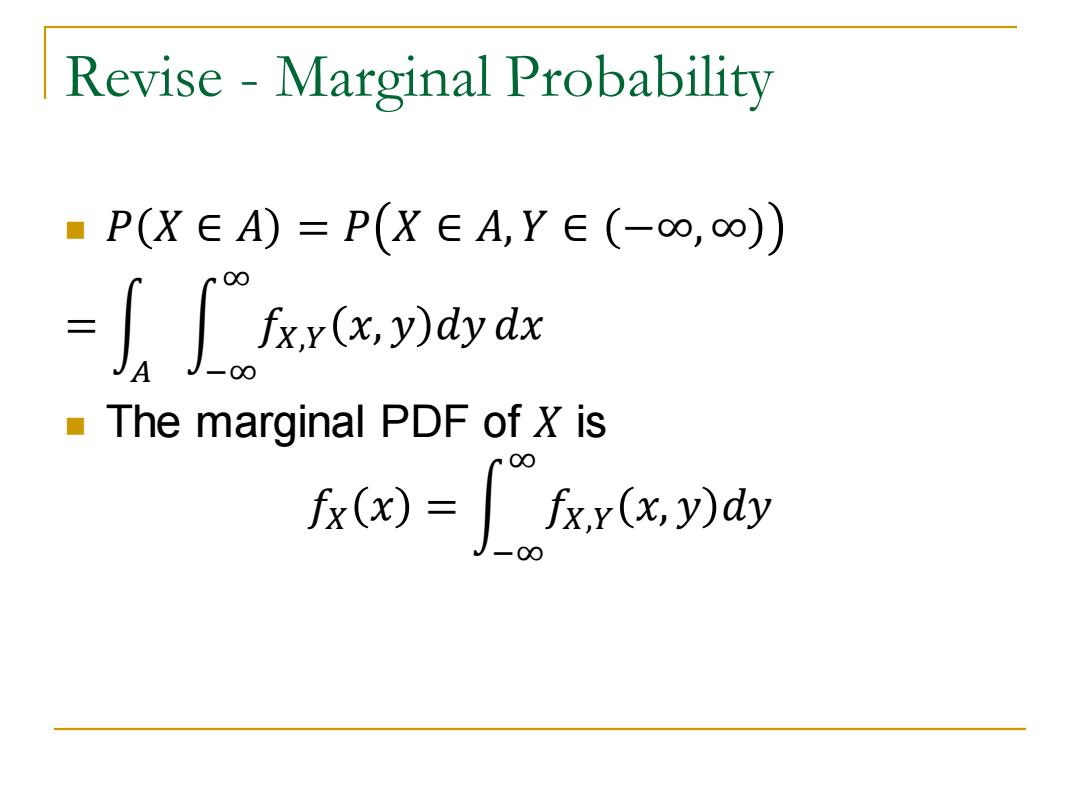
Revise-Marginal Probability ·P(X∈A)=P(X∈A,Y∈(-∞,∞) =∫rx妙r 0∞ The marginal PDF of X is 00 fx(x)=fx.v(x.y)dy
Revise - Marginal Probability ◼ 𝑃 𝑋 ∈ 𝐴 = 𝑃 𝑋 ∈ 𝐴, 𝑌 ∈ −∞, ∞ = න 𝐴 න −∞ ∞ 𝑓𝑋,𝑌 𝑥, 𝑦 𝑑𝑦 𝑑𝑥 ◼ The marginal PDF of 𝑋 is 𝑓𝑋 𝑥 = න −∞ ∞ 𝑓𝑋,𝑌 𝑥, 𝑦 𝑑𝑦
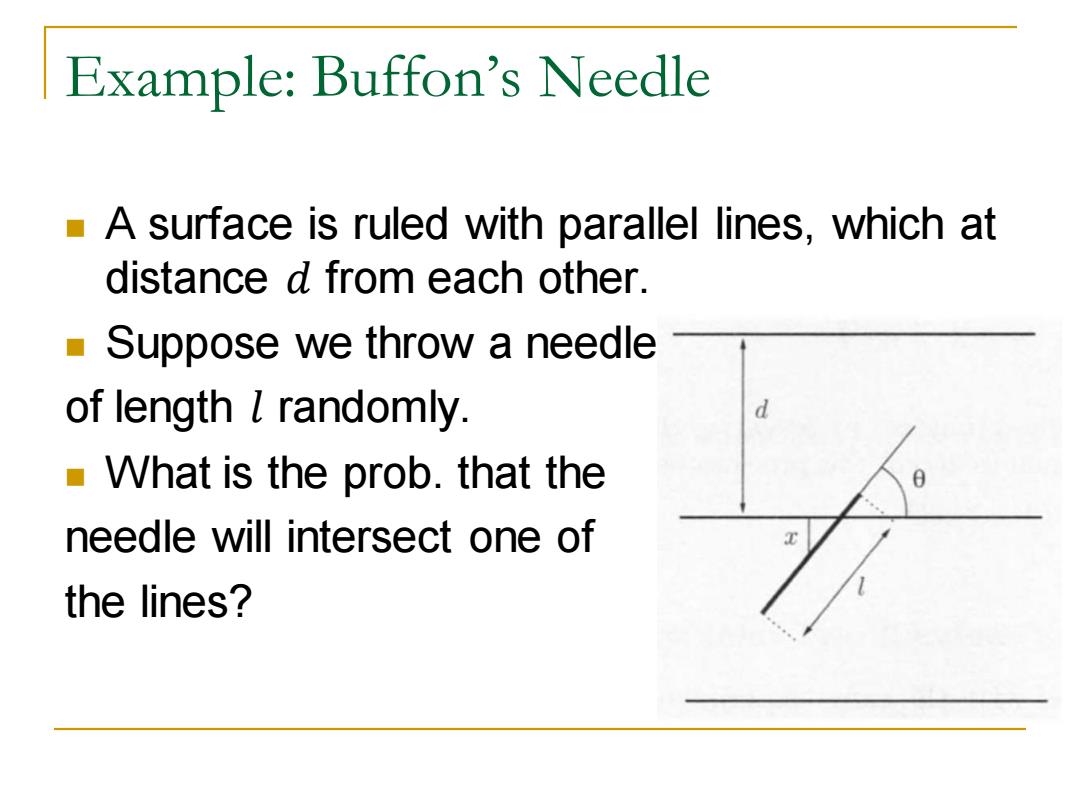
Example:Buffon's Needle A surface is ruled with parallel lines,which at distance d from each other. Suppose we throw a needle of length l randomly. What is the prob.that the needle will intersect one of the lines?
Example: Buffon’s Needle ◼ A surface is ruled with parallel lines, which at distance 𝑑 from each other. ◼ Suppose we throw a needle of length 𝑙 randomly. ◼ What is the prob. that the needle will intersect one of the lines?

Example:Buffon's Needle -Assume l<d so that the needle cannot intersect two lines simultaneously. X,the distance from the middle point of the needle and the nearest of the parallel lines 9,the acute angle formed by the needle and the lines
Example: Buffon’s Needle ◼ Assume 𝑙 < 𝑑 so that the needle cannot intersect two lines simultaneously. ◼ 𝑋, the distance from the middle point of the needle and the nearest of the parallel lines ◼ 𝜗, the acute angle formed by the needle and the lines