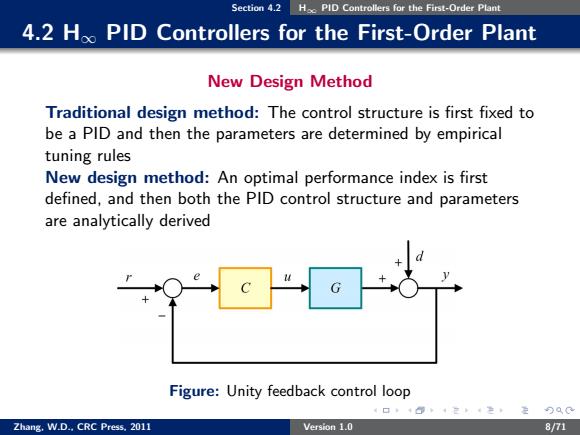
Section 4.2 Hc PID Controllers for the First-Order Plant 4.2 Ho PID Controllers for the First-Order Plant New Design Method Traditional design method:The control structure is first fixed to be a PlD and then the parameters are determined by empirical tuning rules New design method:An optimal performance index is first defined,and then both the PlD control structure and parameters are analytically derived Figure:Unity feedback control loop 4口+@4定4定0C Zhang.W.D..CRC Press.2011 Version 1.0 8/71
Section 4.2 H∞ PID Controllers for the First-Order Plant 4.2 H∞ PID Controllers for the First-Order Plant New Design Method Traditional design method: The control structure is first fixed to be a PID and then the parameters are determined by empirical tuning rules New design method: An optimal performance index is first defined, and then both the PID control structure and parameters are analytically derived Figure: Unity feedback control loop Zhang, W.D., CRC Press, 2011 Version 1.0 8/71
Section 4.2 Hc PID Controllers for the First-Order Plant Consider the unity feedback control system.According to the Youla parameterization,all stabilizing controllers can be expressed as Q(s) C(S)=1-G(5)Q(S) where Q(s)is a stable transfer function.If the model is exact,the transfer function from d(s)to y(s)is given by S(s)=1-G(s)Q(s) Take the performance index as min llW(s)s(s)llo where W(s)is a weighting function.It should be chosen so that the 2-norm boundary of the system input is normalized by unity Zhang.W.D..CRC Press.2011 Version 1.0 9/71
Section 4.2 H∞ PID Controllers for the First-Order Plant Consider the unity feedback control system. According to the Youla parameterization, all stabilizing controllers can be expressed as C(s) = Q(s) 1 − G(s)Q(s) where Q(s) is a stable transfer function. If the model is exact, the transfer function from d(s) to y(s) is given by S(s) = 1 − G(s)Q(s) Take the performance index as min kW (s)S(s)k∞ where W (s) is a weighting function. It should be chosen so that the 2-norm boundary of the system input is normalized by unity Zhang, W.D., CRC Press, 2011 Version 1.0 9/71
Section 4.2 Hoc PID Controllers for the First-Order Plant It is impossible to design a controller for any inputs.Assume that the input is a step,i.e.d(s)=1/s.One can take W(s)=1/s. Consider the first-order plant with time delay: Ke-0s G(s)= T5+1 Many plants can be described by the model.With the 1/1 Pade approximant es≈ 1-0s/2 1+0s/2 the approximate plant is 1-0s/2 G(S)≈K (rs+1)(1+0s/2) Basic idea:Design the controller for the approximate plant and then use it for the original plant 2月aC Zhang.W.D..CRC Press.2011 Version 1.0 10/71
Section 4.2 H∞ PID Controllers for the First-Order Plant It is impossible to design a controller for any inputs. Assume that the input is a step, i.e. d(s) = 1/s. One can take W (s) = 1/s. Consider the first-order plant with time delay: G(s) = Ke−θs τ s + 1 Many plants can be described by the model. With the 1/1 Pade approximant e −θs ≈ 1 − θs/2 1 + θs/2 the approximate plant is G(s) ≈ K 1 − θs/2 (τ s + 1)(1 + θs/2) Basic idea: Design the controller for the approximate plant and then use it for the original plant Zhang, W.D., CRC Press, 2011 Version 1.0 10/71

Section 4.2 H PID Controllers for the First-Order Plant Theorem (Maximum Modulus Theorem) Assume that n is a nonempty region in the complex plane and F(s)is a function that does not have poles in 9.If F(s)is not a constant,then F(s)does not attain its maximum value at an interior point of Assume that n equals the open RHP.W(s)S(s)should not have poles in n.By Theorem we have W(s)s(s)=W(s)1-G(s)Q(s) sup W(s)1-G(s)Q(s) Res>0 G(s)has a zero at s=2/0 in the open RHP.Accordingly supW(s)1-G(s)Q(s川≥w(s1-G(sQ(s=2/al=0/2 Res>0 4口+@4定4生定9QC Zhang.W.D..CRC Press.2011 Version 1.0 11/71
Section 4.2 H∞ PID Controllers for the First-Order Plant Theorem (Maximum Modulus Theorem) Assume that Ω is a nonempty region in the complex plane and F(s) is a function that does not have poles in Ω. If F(s) is not a constant, then |F(s)| does not attain its maximum value at an interior point of Ω. Assume that Ω equals the open RHP. W (s)S(s) should not have poles in Ω. By Theorem we have kW (s)S(s)k∞ = kW (s)[1 − G(s)Q(s)]k∞ = sup Res>0 |W (s)[1 − G(s)Q(s)]| G(s) has a zero at s = 2/θ in the open RHP. Accordingly sup Res>0 |W (s)[1 − G(s)Q(s)]| ≥ |W (s)[1 − G(s)Q(s)]|s=2/θ| = θ/2 Zhang, W.D., CRC Press, 2011 Version 1.0 11/71
Section 4.2 H PID Controllers for the First-Order Plant Theorem (Maximum Modulus Theorem) Assume that n is a nonempty region in the complex plane and F(s)is a function that does not have poles in 9.If F(s)is not a constant,then F(s)does not attain its maximum value at an interior point of Assume that equals the open RHP.W(s)S(s)should not have poles in By Theorem we have llW(s)s(s)llo=lIW(s)[1-G(s)Q(s)]ll o =sup IW(s)[1-G(s)Q(s)]I Res>0 G(s)has a zero at s=2/0 in the open RHP.Accordingly sup W(s)[1-G(s)Q(s)]I W(s)[1-G(s)Q(s)]ls=2/1=0/2 Res>0 定QC Zhang.W.D..CRC Press.2011 Version 1.0 11/71
Section 4.2 H∞ PID Controllers for the First-Order Plant Theorem (Maximum Modulus Theorem) Assume that Ω is a nonempty region in the complex plane and F(s) is a function that does not have poles in Ω. If F(s) is not a constant, then |F(s)| does not attain its maximum value at an interior point of Ω. Assume that Ω equals the open RHP. W (s)S(s) should not have poles in Ω. By Theorem we have kW (s)S(s)k∞ = kW (s)[1 − G(s)Q(s)]k∞ = sup Res>0 |W (s)[1 − G(s)Q(s)]| G(s) has a zero at s = 2/θ in the open RHP. Accordingly sup Res>0 |W (s)[1 − G(s)Q(s)]| ≥ |W (s)[1 − G(s)Q(s)]|s=2/θ| = θ/2 Zhang, W.D., CRC Press, 2011 Version 1.0 11/71