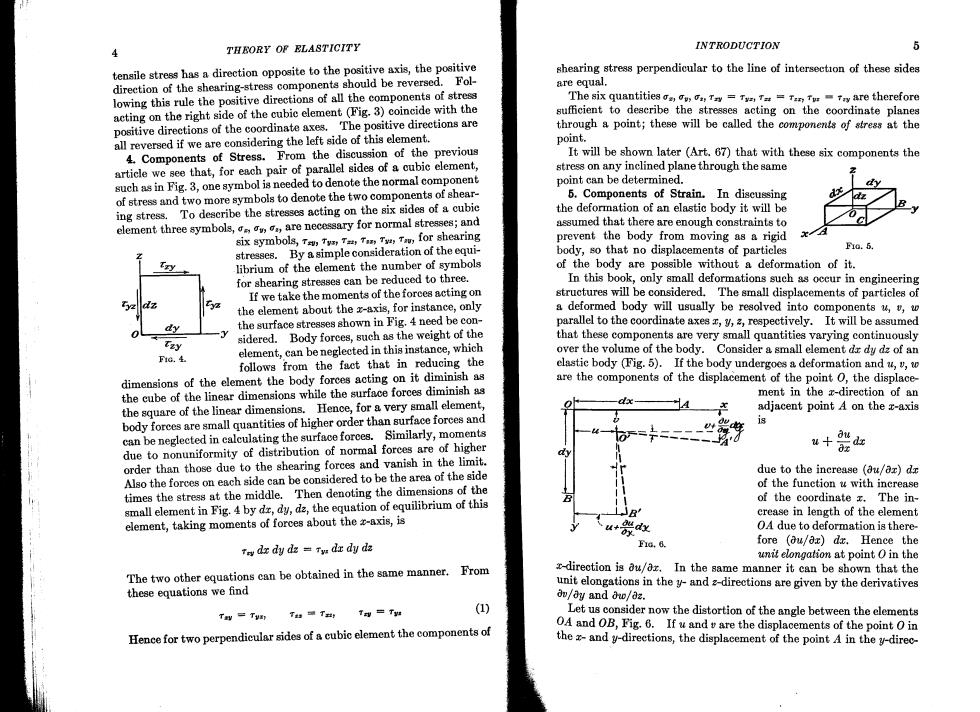
THEORY OF ELASTICITY INTRODUCTION 5 tensile stress has a direction opposite to the positive axis,the positive shearing stress perpendicular to the line of intersection of these sides direction of the shearing-stress components should be reversed.Fol- are equal. lowing this rule the positive directions of all the components of stress The six quantities v,T=TTa=Tan TurTn are therefore acting on the right side of the cubic element(Fig.3)coincide with the sufficient to describe the stresses acting on the coordinate planes positive directions of the coordinate axes.The positive directions are through a point;these will be called the components of stress at the all reversed if we are considering the left side of this element. point. 4.Components of Stress.From the discussion of the previous It will be shown later (Art.67)that with these six components the article we see that,for each pair of parallel sides of a cubic element, stress on any inclined plane through the same such as in Fig.3,one symbol is needed to denote the normal component point can be determined. of stress and two more symbols to denote the two components of shear- 5.Components of Strain.In discussing ing stress.To describe the stresses acting on the six sides of a cubic the deformation of an elastic body it will be element three symbols,,are necessary for normal stresses;and assumed that there are enough constraints to six symbols,Ta,TyTT,T,Tau,for shearing prevent the body from moving as a rigid stresses.By a simple consideration of the equi- body,so that no displacements of particles 上0.5. 2 librium of the element the number of symbols of the body are possible without a deformation of it. for shearing stresses can be reduced to three. In this book,only small deformations such as occur in engineering If we take the moments of the forces acting on structures will be considered.The small displacements of particles of dz the element about the x-axis,for instance,only a deformed body will usually be resolved into components u,v,w dy the surface stresses shown in Fig.4 need be con- parallel to the coordinate axes r,y,2,respectively.It will be assumed sidered.Body forces,such as the weight of the that these components are very small quantities varying continuously F1G.4 element,can be neglected in this instance,which over the volume of the body.Consider a small element dr dy da of an follows from the fact that in reducing the elastie body (Fig.5).If the body undergoes a deformation and v,v,w dimensions of the element the body forces acting on it diminish as are the components of the displacement of the point O,the displace- the cube of the linear dimensions while the surface forces diminish as ment in the x-direction of an the square of the linear dimensions.Hence,for a very small element, dx- adjacent point A on the x-axis body forees are small quantities of higher order than surface forces and is can be neglected in calculating the surface forces. Similarly,moments due to nonuniformity of distribution of normal forces are of higher u+器业 order than those due to the shearing forces and vanish in the limit. due to the increase (ou/oz)dx Also the forces on each side can be considered to be the area of the side times the stress at the middle.Then denoting the dimensions of the of the function u with increase of the coordinate x.The in- small element in Fig.4 by dr,dy,dz,the equation of equilibrium of this crease in length of the element element,taking moments of forces about the x-axis,is u+器 OA due to deformation is there- Tey dx dy dz Ty:dx dy dz ic,6. fore (ou/ar)dr.Hence the unit elongation at point 0 in the The two other equations can be obtained in the same manner. From x-direction is au/az.In the same manner it can be shown that the these equations we find unit elongations in the y-and z-directions are given by the derivatives dv/ay and ow/az. TT Ta Tyr 1) T=T Let us consider now the distortion of the angle between the elements Hence for two perpendicular sides of a cubic element the components of OA and OB,Fig.6.If u and v are the displacements of the point O in the x-and y-directions,the displacement of the point A in the y-direc-

THEORY OF ELASTICITY INTRODUCTION 7 6 tion and of the point B in the z-direction are +(dv/ax)dz and Extension of the element in the x-direction is accompanied by lateral u+(ou/ay)dy,respectively.Due to these displacements the new contractions, direction O'A'of the element OA is inelined to the initial direction by () the small angle indicated in the figure,equal to av/oz.In the same =-觉 。=-喷 manner the direction O'B'is inclined to OB by the small angle au/ay. From this it will be seen that the initially right angle A0B between the in which y is a constant called Poisson's ratio.For many materials two elements OA and OB is diminished by the angle av/az+au/ay. Poisson's ratio can be taken equal to 0.25.For struetural steel it is This is the shearing strain between the planes re and yz.The shearing usually taken equal to 0.3. strains between the planes zy and ze and the planes yz and yz can be Equations (a)and (b)can be used also for simple compression. Within the elastic limit the modulus of elasticity and Poisson's ratio obtained in the same manner. We shall use the letter e for unit elongation and the letter y for unit in compression are the same as in tension. If the above element is submitted to the action of normal stresses shearing strain.To indicate the directions of strain we shall use the same subscripts to these letters as for the stress components.Then ,uniformly distributed over the sides,the resultant components of strain can be obtained by using Eqs.(a)and ()Experiments from the above discussion show that to get these components we have to superpose the strain com- u a 8w ponents produced by each of the three stresses.By this method of 6a≠8 fy= ay' e:=02 superposition we obtain the equations (2 w-箭+品 + du Yu= Yw:= +删 e=Eo:-a,+] It will be shown later that,having the three unit elongations in three 1 (3) perpendicular directions and three unit shear strains related to the 6=2,-(o:+训 same directions,the elongation in any direction and the distortion of 1 the angle between any two directions can be calculated (see Art.73). 6=El-a:十w】 The six quantities,·.·,Yare called the componentso对strain, In our further discussion we shall often use this method of superposi- 6.Hooke's Law.The relations between the components of stress lion in caleulating total deformations and stresses produced by several and the components of strain have been established experimentally and are known as Hooke's law.Imagine an elemental rectangular paral- forces.This method is legitimate as long as the deformations are small lelopiped with the sides parallel to the coordinate axes and submitted and the corresponding small displacements do not affect substantially the action of the external forces.In such cases we neglect small to the action of normal stress uniformly distributed over two oppo- changes in dimensions of deformed bodies and also small displacements site sides.Experiments show that in the case of an isotropic material of the points of application of external forces and base our calculations these normal stresses do not produce any distortion of angles of the ele- on initial dimensions and initial shape of the body.The resultant dis- ment.The magnitude of the unit elongation of the element is given placements will then be obtained by superposition in the form of linear by the equation functions of external forces,as in deriving Eqs.(3). (a) There are,however,exceptional cases in which small deformations cannot be neglected but must be taken into consideration.As an in which E is the modulus of elasticity in tension.Materials used in example of this kind the case of the simultaneous action on a thin bar engineering structures have moduli which are very large in comparison of axial and lateral forces may be mentioned.Axial forces alone pro- with allowable stresses,and the unit elongation (a)is a very small duce simple tension or compression,but they may have a substantial quantity.In the case of structural steel,for instance,it is usually effect on the bending of the bar if they are acting simultaneously with smaller than 0.001. lateral forces.In calculating the deformation of bars under such con-
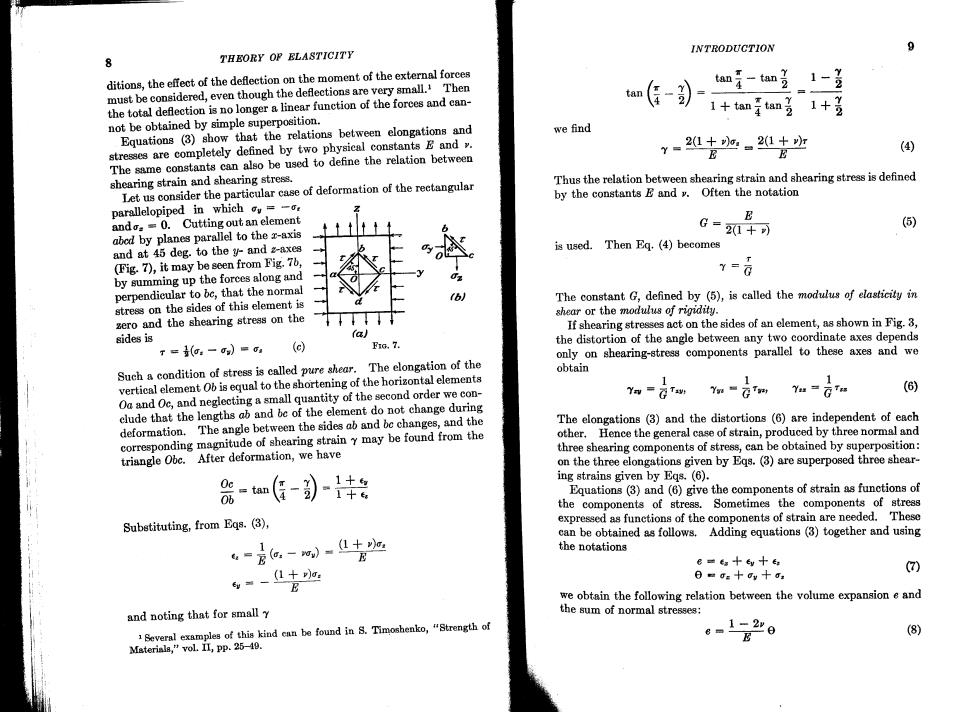
INTRODUCTION 9 8 THEORY OP ELASTICITY ditions,the effect of the deflection on the moment of the external forces tan must be considered,even though the deflections are very small.Then 4-tan Y 2 1-多 tan the total deflection is no longer a linear function of the forces and can- 1+amam1+宽 not be obtained by simple superposition. Equations (3)show that the relations between elongations and we find stresses are completely defined by two physical constants E and v. Y=21+。21+归 (4 The same constants can also be used to define the relation between 内 E shearing strain and shearing stress. Thus the relation between shearing strain and shearing stress is defined Let us consider the particular case of deformation of the rectangular by the constants E and v.Often the notation parallelopiped in which=- ando==0.Cutting out an element E G=21+可 () abcd by planes parallel to the r-axis and at 45 deg.to the y-and z-axes is used.Then Eq.(4)becomes (Fig.7),it may be seen from Fig.70, T Y=G by summing up the forces along and perpendicular to be,that the normal b The constant G,defined by (5),is called the modulus of elasticity in stress on the sides of this element is shear or the modulus of rigidity. zero and the shearing stress on the If shearing stresses act on the sides of an element,as shown in Fig.3, sides is a T=(a:一)= (e) Fa,7. the distortion of the angle between any two coordinate axes depends only on shearing-stress components parallel to these axes and we Such a condition of stress is called pure shear.The elongation of the obtain vertical element Ob is equal to the shortening of the horizontal elements 1 1 Ya-gTa Ya -GTm Ya-GTe (6) Oa and Oc,and neglecting a small quantity of the second order we con- clude that the lengths ab and be of the element do not change during The angle between the sides ab and be changes,and the The elongations (3)and the distortions(6)are independent of each deformation. corresponding magnitude of shearing strain y may be found from the other.Hence the general case of strain,produced by three normal and triangle Obc. After deformation,we have three shearing components of stress,can be obtained by superposition: on the three elongations given by Eqs.(3)are superposed three shear- 1十 ing strains given by Eqs.(6). Equations(3)and(6)give the components of strain as functions of the components of stress.Sometimes the components of stress Substituting,from Eqs.(3), expressed as functions of the components of strain are needed.These can be obtained as follows.Adding equations (3)together and using 。言a~,)-1+ 1 the notations e=ea十ey十ea =-L+a 0=g:十0g十, () we obtain the following relation between the volume expansion e and and noting that for small y the sum of normal stresses: iSeveral examples of this kind can be found in S.Timoshenko,"Strength of -1-220 (⑧) Materisla,"voL.II,pp.25-49

10 THEORY OF ELASTICITY In the case of a uniform hydrostatie pressure of the amount p we have 0:=0y=gx=一卫 and Eq.(8)gives e=-3(1-2p CHAPTER 2 PLANE STRESS AND PLANE STRAIN which represents the relation between unit volume expansion e and hydrostatic pressure p. 7.Plane Stress.If a thin plate is loaded by forces applied at the The quantity E/3(1-2)is called the modulus of volume expansion. boundary,parallel to the plane of the plate and distributed uniformly Using notations (7)and solving Eqs.(3)for we find over the thickness (Fig.8),the stress components are zero on B both faces of the plate,and it may be assumed,tentatively,that they -1+1-2阿6+1+, 6 are zero also within the plate.The state of stress is then specified by E ,only,and is called plane stress.It may also be assumed that ,=0+1-2可e+1千, (9) E ,=1+1-2可6+1+,% or using the notation vE (10) 入=0+)(1-2可 and Eq.(5),these become Ga he 2Ges au =Ne 2Gey (11) Ga he +2Ge rG.8. Problems these three components are independent of z,i.e.,they do not vary 1.Show that Eqs.(1)continue to hold if the element of Fig.4 is in motion and through the thiekness.They are then functions of z and y only. has an angular acceleration like a rigid body. 8.Plane Strain.A similar simplification is possible at the other 2.Suppose an elnstie material contains a large number of evenly distributed small magnetized particles,so that a magnetic field exerts on any element ds dy ds extreme when the dimension of the body in the z-direction is very large. a moment dr dy ds about an axis parallel to the z-axis. What modification will If a long cylindrical or prismatical body is loaded by forces which are perpendicular to the longitudinal elements and do not vary along the be needed in Egs.(1)? 3.Give some reasons why the formulas (2)will be valid for small atrains only. length,it may be assumed that all cross sections are in the same condi- 4.An elastic layer is sandwiched between two perfectly rigid plates,to which it tion.It is simplest to suppose at first that the end sections are con- is bonded.The layer is compressed between the plates,the compressive stress being Supposing that the attachment to the plates prevents lateral strain fined between fixed smooth rigid planes,so that displacement in the completely,find the apparent Young's modulus (i.e in terms of gandv. axial direetion is prevented.The effect of removing these will be Show that it is many times E if the material of the layer is nearly incompresaible by examined later.Since there is no axial displacement at the ends,and, by symmetry,at the mid-section,it may be assumed that the same bydrostatic pressure. 5.Prove that Ea.(8)follows from Eqs.(11),(10),and (5). holds at every cross section. There are many important problems of this kind-a retaining wall with lateral pressure(Fig.9),a culvert or tunnel (Fig.10),a cylindrical tube with internal pressure,a cylindrical roller compressed by forces in 11 INSTITUTUL POLITEHN TIMI5OARA· BiBLIOTECA CENTRALA

12 THEORY OF ELASTICITY PLANE STRESS AND PLANE STRAIN 13 a diametral plane as in a roller bearing (Fig.11).In each case of By Egs.(a)and (6),the stress components r and r are zero,and, course the loading must not vary along the length.Since conditions by Eq.(b),o,can be found from os and Thus the plane strain prob- are the same at all cross sections,it is sufficient to consider only a slice lem,like the plane stress problem,reduces to the determination of between two sections unit distance apart.The components u and v of v,and T as functions of x and y only. the displacement are functions of z and y but are independent of the 9.Stress at a Point.Knowing the stress components at any point of a plate in a condition of plane stress or plane strain,the stress acting on any plane through this point perpendicular to the plate and inclined to the x-and y-axes can be calculated from the equations of statics.Let O be a point of the stressed plate and suppose the stress components a,,Tav are known (Fig.12).To find the stress for any plane through the z-axis and inclined to the x-and y-axes,we take a plane BC parallel to it,at a small distance from O,so that this latter plane together with the coordinate planes cuts out from the plate a very small triangular prism OBC.Since the stresses vary continuously over the volume of the body the stress acting F1a,12 on the plane BC will approach the stress on the parallel plane through O as the element is made smaller. In discussing the conditions of equilibrium of the small triangular F0.11. prism,the body force can be neglected as a small quantity of a higher longitudinal coordinate 2.Since the longitudinal displacement w is order (page 4).Likewise,if the element is very small,we can negleet zero,Egs.(②)give the variation of the stresses over the sides and assume that the stresses are uniformly distributed.The forees acting on the triangular prism can therefore be determined by multiplying the stress components by at =0 加-架+器 (a) the areas of the sides.Let N be the direction of the normal to the plane BC,and denote the cosines of the angles between the normal N du2 0 6=a2 and the axes x and y by cos Nx =1,cos Ny m The longitudinal normal stress o.can be found in terms of o and o by means of Hooke's law,Eqs.(3).Since e=0 we find Then,if A denotes the area of the side BC of the element,the areas of the other two sides are Al and Am. o:-(a=十aw〉■0 If we denote by and P the components of stress acting on the side :=(ca十) () BC,the equations of equilibrium of the prismatical element give These normal stresses act over the cross sections,including the ends, 及=laa十mry (12) where they represent forces required to maintain the plane strain,and 卫=mag十rm provided by the fixed smooth rigid planes. Thus the components of stress on any plane defined by the direction