
Definition(Cartesian Products) The Cartesian product A x B of A and B is defined as A×Be{(a,b)|a∈AAb∈B} X2会X×X 4口,¥①,43,t夏,30Q0 Jun iE jtmomjtedn.cn 1-9 Set Theory (II):Relations 2021 1202 7/52
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Definition (Cartesian Products) The Cartesian product A × B of A and B is defined as A × B , {(a, b) | a ∈ A ∧ b ∈ B} X2 , X × X Theorem A × B is a set. Proof. A × B , {(a, b) ∈ ? | a ∈ A ∧ b ∈ B} { {a}, {a, b} } ∈ Jun Ma (majun@nju.edu.cn) 1-9 Set Theory (II): Relations 2021 年 12 月 02 日 7 / 52

Definition(Cartesian Products) The Cartesian product A x B of A and B is defined as A×Be{(a,b)|a∈AAb∈B} X2会X×X Theorem A×B is a set.. 4口,¥①,43,t夏,30Q0 Jun i jumcmjtedn.cn 1-9 Set Theory (II):Relations 2021 1202 7/52
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Definition (Cartesian Products) The Cartesian product A × B of A and B is defined as A × B , {(a, b) | a ∈ A ∧ b ∈ B} X2 , X × X Theorem A × B is a set. Proof. A × B , {(a, b) ∈ ? | a ∈ A ∧ b ∈ B} { {a}, {a, b} } ∈ Jun Ma (majun@nju.edu.cn) 1-9 Set Theory (II): Relations 2021 年 12 月 02 日 7 / 52

Definition(Cartesian Products) The Cartesian product A x B of A and B is defined as A×B≌{(a,b)|a∈AAb∈B} X2会X×X Theorem A×B is a set. Proof. A×B≌{(a,b)∈?|a∈AAb∈B} Jun Ma (majun&inju.edu.cn) 1-9 Set Theory (II):Relations 2021年12月02日 7/52
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Definition (Cartesian Products) The Cartesian product A × B of A and B is defined as A × B , {(a, b) | a ∈ A ∧ b ∈ B} X2 , X × X Theorem A × B is a set. Proof. A × B , {(a, b) ∈ ? | a ∈ A ∧ b ∈ B} { {a}, {a, b} } ∈ Jun Ma (majun@nju.edu.cn) 1-9 Set Theory (II): Relations 2021 年 12 月 02 日 7 / 52
Definition(Cartesian Products) The Cartesian product A x B of A and B is defined as A×Be{(a,b)|a∈AAb∈B} X2会X×X Theorem A×B is a set.. Proof. A×B≌{(a,b)∈?|a∈AAb∈B} {a,{a,b}∈? Jun Ma (majun&inju.edu.cn) 1-9 Set Theory (II):Relations 2021年12月02日 7/52
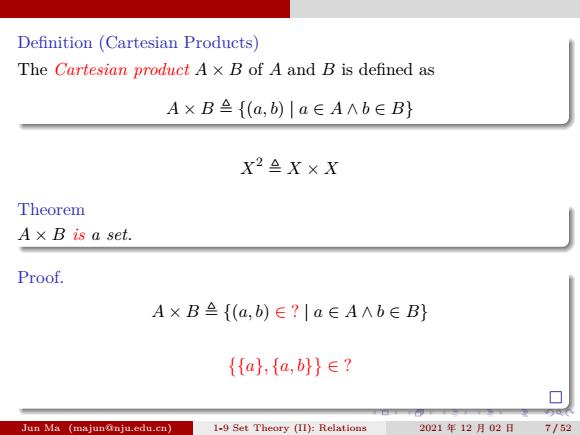
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Definition (Cartesian Products) The Cartesian product A × B of A and B is defined as A × B , {(a, b) | a ∈ A ∧ b ∈ B} X2 , X × X Theorem A × B is a set. Proof. A × B , {(a, b) ∈ ? | a ∈ A ∧ b ∈ B} { {a}, {a, b} } ∈ ? Jun Ma (majun@nju.edu.cn) 1-9 Set Theory (II): Relations 2021 年 12 月 02 日 7 / 52

Definition(Cartesian Products) The Cartesian product A x B of A and B is defined as A×B≌{(a,b)|a∈AAb∈B} X2会X×X Theorem A×B is a set.. Proof. A×B≌{(a,b)∈?|a∈AAb∈B} {a},{a,b}∈P(P(AUB)》 Jun Ma (majunnju.edu.cn) 1-9 Set Theory (II):Relations 2021年12月02日 7/52
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Definition (Cartesian Products) The Cartesian product A × B of A and B is defined as A × B , {(a, b) | a ∈ A ∧ b ∈ B} X2 , X × X Theorem A × B is a set. Proof. A × B , {(a, b) ∈ ? | a ∈ A ∧ b ∈ B} { {a}, {a, b} } ∈ P(P(A ∪ B)) Jun Ma (majun@nju.edu.cn) 1-9 Set Theory (II): Relations 2021 年 12 月 02 日 7 / 52