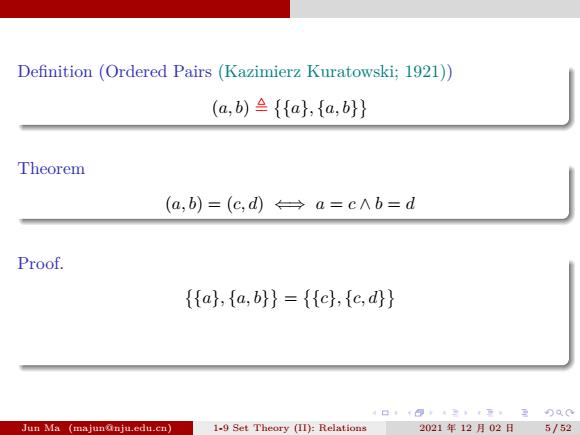
Definition(Ordered Pairs (Kazimierz Kuratowski;1921)) (a,b){a},{a,b} Theorem (a,b)=(c,d→a=c∧b=d Proof. {ah,{a,b}={c,{c,d} 4口,1①,43,t夏,30Q0 Jun iE jtmomjtedn.cn 1-9 Set Theory (II):Relations 2021 1202 5/52
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Definition (Ordered Pairs (Kazimierz Kuratowski; 1921)) (a, b) , { {a}, {a, b} } Theorem (a, b) = (c, d) ⇐⇒ a = c ∧ b = d Proof. { {a}, {a, b} } = { {c}, {c, d} } Case I : a = b Case II : a ̸= b Jun Ma (majun@nju.edu.cn) 1-9 Set Theory (II): Relations 2021 年 12 月 02 日 5 / 52
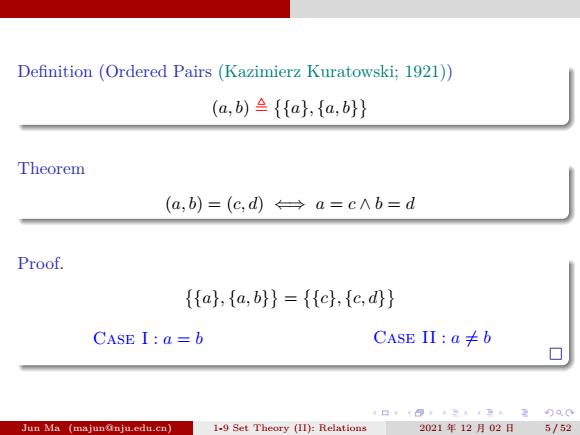
Definition(Ordered Pairs (Kazimierz Kuratowski;1921)) (a,b){a},{a,b}} Theorem (a,b)=(c,d)→a=c∧b=d Proof. {a,{a,b}={c,{c,d} CASE I:a=b CASE II:a≠b 4口·¥①,43,t夏,里Q0 Jun Ma (majunainju.edu.cn) 1-9 Set Theory (II):Relations 2021年12月02日5/52
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Definition (Ordered Pairs (Kazimierz Kuratowski; 1921)) (a, b) , { {a}, {a, b} } Theorem (a, b) = (c, d) ⇐⇒ a = c ∧ b = d Proof. { {a}, {a, b} } = { {c}, {c, d} } Case I : a = b Case II : a ̸= b Jun Ma (majun@nju.edu.cn) 1-9 Set Theory (II): Relations 2021 年 12 月 02 日 5 / 52

Definition (Ordered Pairs(Norbert Wiener;1914)) (a,b){{a,0,{Hb} p内 F 4口,1①,43,t夏,30Q0 Jun iE jtmomjtedn.cn 1-9 Set Theory (II):Relations 2021 1202 6/52
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Definition (Ordered Pairs (Norbert Wiener; 1914)) (a, b) , {{ {a}, ∅ } , { {b} }} Theorem (a, b) = (c, d) ⇐⇒ a = c ∧ b = d Jun Ma (majun@nju.edu.cn) 1-9 Set Theory (II): Relations 2021 年 12 月 02 日 6 / 52

Definition (Ordered Pairs (Norbert Wiener;1914)) (a,b){{a,0,{Hb} p“内4 8F飞) Theorem (a,b)=(c,d→a=cAb=d 4口,1①,43,t夏,30Q0 Jun Ma (majunainju.edu.cn) 1-9 Set Theory(I):Relations2021年12月02日 6/52
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Definition (Ordered Pairs (Norbert Wiener; 1914)) (a, b) , {{ {a}, ∅ } , { {b} }} Theorem (a, b) = (c, d) ⇐⇒ a = c ∧ b = d Jun Ma (majun@nju.edu.cn) 1-9 Set Theory (II): Relations 2021 年 12 月 02 日 6 / 52

Definition(Cartesian Products) The Cartesian product A x B of A and B is defined as A×Be{(a,b)|a∈AAb∈B} 4口,¥①,43,t夏,30Q0 Jun i jumcmjtedn.cn 1-9 Set Theory (II):Relations 2021 1202 7/52
. . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Definition (Cartesian Products) The Cartesian product A × B of A and B is defined as A × B , {(a, b) | a ∈ A ∧ b ∈ B} X2 , X × X Theorem A × B is a set. Proof. A × B , {(a, b) ∈ ? | a ∈ A ∧ b ∈ B} { {a}, {a, b} } ∈ Jun Ma (majun@nju.edu.cn) 1-9 Set Theory (II): Relations 2021 年 12 月 02 日 7 / 52