
版权所有:华东理工大学物理化学教研室 11 4) The general case of a reaction It follows that r C D BA Δ G = cμ + dμ − aμ − bμ ⎟⎟⎠⎞ ⎜⎜⎝⎛ = ba dc aa aa Q BA DC 9.1 The Gibbs energy minimum C D A B r BADC ln ln ln ln Δ abRTaaRTadRTacRT badcG + + − − −−+= oooo μμμμ Since JJ J += ln aRT o μμ ⎟⎟⎠⎞ ⎜⎜⎝⎛ += ba dc aa aa RTGG BA DC rr ΔΔ ln o
版权所有:华东理工大学物理化学教研室 11 4) The general case of a reaction It follows that r C D BA Δ G = cμ + dμ − aμ − bμ ⎟⎟⎠⎞ ⎜⎜⎝⎛ = ba dc aa aa Q BA DC 9.1 The Gibbs energy minimum C D A B r BADC ln ln ln ln Δ abRTaaRTadRTacRT badcG + + − − −−+= oooo μμμμ Since JJ J += ln aRT o μμ ⎟⎟⎠⎞ ⎜⎜⎝⎛ += ba dc aa aa RTGG BA DC rr ΔΔ ln o
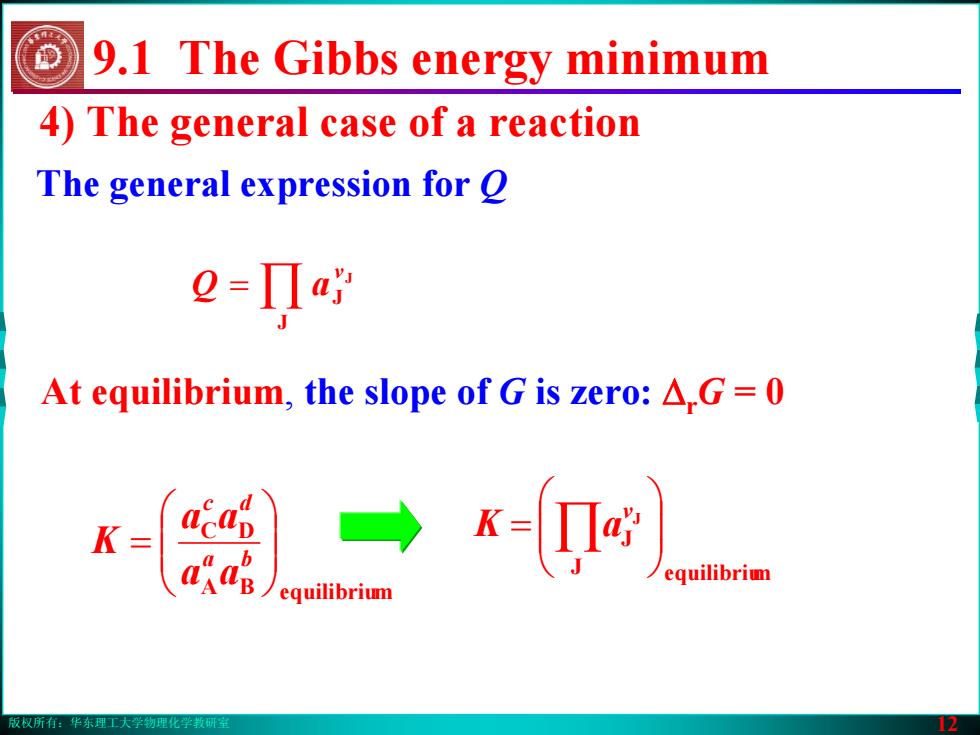
版权所有:华东理工大学物理化学教研室 12 4) The general case of a reaction The general expression for Q = ∏ J J J ν aQ At equilibrium, the slope of G is zero: ΔrG = 0 BA mequilibriu DC ⎟⎟⎠⎞ ⎜⎜⎝⎛ = ba dc aa aa K J mequilibriu JJ ⎟⎟⎠⎞ ⎜⎜⎝⎛ = ∏ ν aK 9.1 The Gibbs energy minimum
版权所有:华东理工大学物理化学教研室 12 4) The general case of a reaction The general expression for Q = ∏ J J J ν aQ At equilibrium, the slope of G is zero: ΔrG = 0 BA mequilibriu DC ⎟⎟⎠⎞ ⎜⎜⎝⎛ = ba dc aa aa K J mequilibriu JJ ⎟⎟⎠⎞ ⎜⎜⎝⎛ = ∏ ν aK 9.1 The Gibbs energy minimum

版权所有:华东理工大学物理化学教研室 13 4) The general case of a reaction 9.1 The Gibbs energy minimum An equilibrium constant K expressed in terms of activities is called a thermodynamic equilibrium constant. The thermodynamic equilibrium constant is also dimensionless because activities are dimensionless numbers. At equilibrium, ΔrG = 0 This important thermodynamic relation enables us to predict the equilibrium constant of any reaction from tables of thermodynamic data, and hence to predict the equilibrium composition of reaction mixtures. o ln −= ΔrGKRT
版权所有:华东理工大学物理化学教研室 13 4) The general case of a reaction 9.1 The Gibbs energy minimum An equilibrium constant K expressed in terms of activities is called a thermodynamic equilibrium constant. The thermodynamic equilibrium constant is also dimensionless because activities are dimensionless numbers. At equilibrium, ΔrG = 0 This important thermodynamic relation enables us to predict the equilibrium constant of any reaction from tables of thermodynamic data, and hence to predict the equilibrium composition of reaction mixtures. o ln −= ΔrGKRT
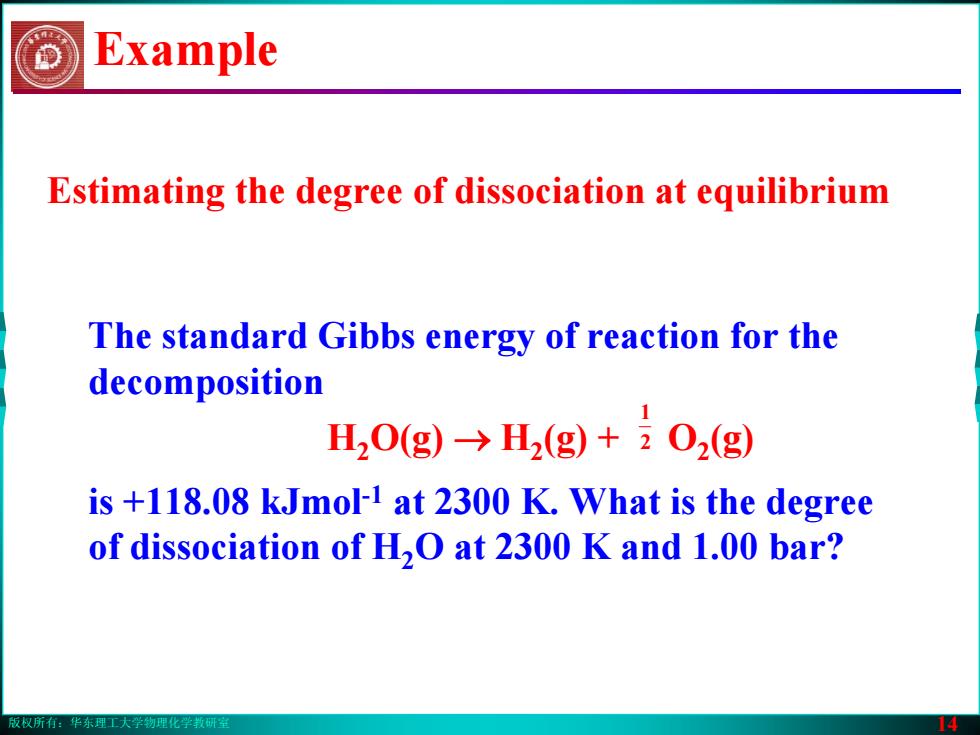
版权所有:华东理工大学物理化学教研室 14 Example The standard Gibbs energy of reaction for the decomposition H2O(g) → H2(g) + O2(g) is +118.08 kJmol-1 at 2300 K. What is the degree of dissociation of H2O at 2300 K and 1.00 bar? 2 1 Estimating the degree of dissociation at equilibrium
版权所有:华东理工大学物理化学教研室 14 Example The standard Gibbs energy of reaction for the decomposition H2O(g) → H2(g) + O2(g) is +118.08 kJmol-1 at 2300 K. What is the degree of dissociation of H2O at 2300 K and 1.00 bar? 2 1 Estimating the degree of dissociation at equilibrium

版权所有:华东理工大学物理化学教研室 15 Method: The equilibrium constant is obtained from the standard Gibbs energy of reaction, so we need to relate the degree of dissociation, α, to K and then to find its numerical value. Proceed by expressing the equilibrium compositions in terms of α, and solve for α in terms of K. Because the standard Gibbs energy of reaction is large and positive, we can anticipate that K will be small, and hence that α << 1, which opens the way to making approximations to obtain its numerical value. Example
版权所有:华东理工大学物理化学教研室 15 Method: The equilibrium constant is obtained from the standard Gibbs energy of reaction, so we need to relate the degree of dissociation, α, to K and then to find its numerical value. Proceed by expressing the equilibrium compositions in terms of α, and solve for α in terms of K. Because the standard Gibbs energy of reaction is large and positive, we can anticipate that K will be small, and hence that α << 1, which opens the way to making approximations to obtain its numerical value. Example