
Heat exchange and 先进材料疑固实验室 Laboratory of Advanced Materials Solidification temperature field One dimensional heat exchange problem Assumptions: 1.Uniform temperature of mold and mold cavity,Pouring temperature T10,mold temperature T20: 2.Pure metal solidification 3. No latent heat release 4. Parameters:Casting:入1、C1、p1 5. Mold:入2、C2、P2 6. No heat resistance between casting and mold Then: ot 02T &t er2 a---Heat diffusion coefficient,=cp) 上降充通大学 SHANGHAI JIAO TONG UNIVERSITY
Assumptions: 1. Uniform temperature of mold and mold cavity, Pouring temperature T10, mold temperature T20: 2. Pure metal solidification 3. No latent heat release 4. Parameters: Casting: λ1 、c1 、ρ1 5. Mold: λ2 、c2 、ρ2 6. No heat resistance between casting and mold Then: Heat exchange and temperature field One dimensional heat exchange problem α---Heat diffusion coefficient , = λ/(cρ)
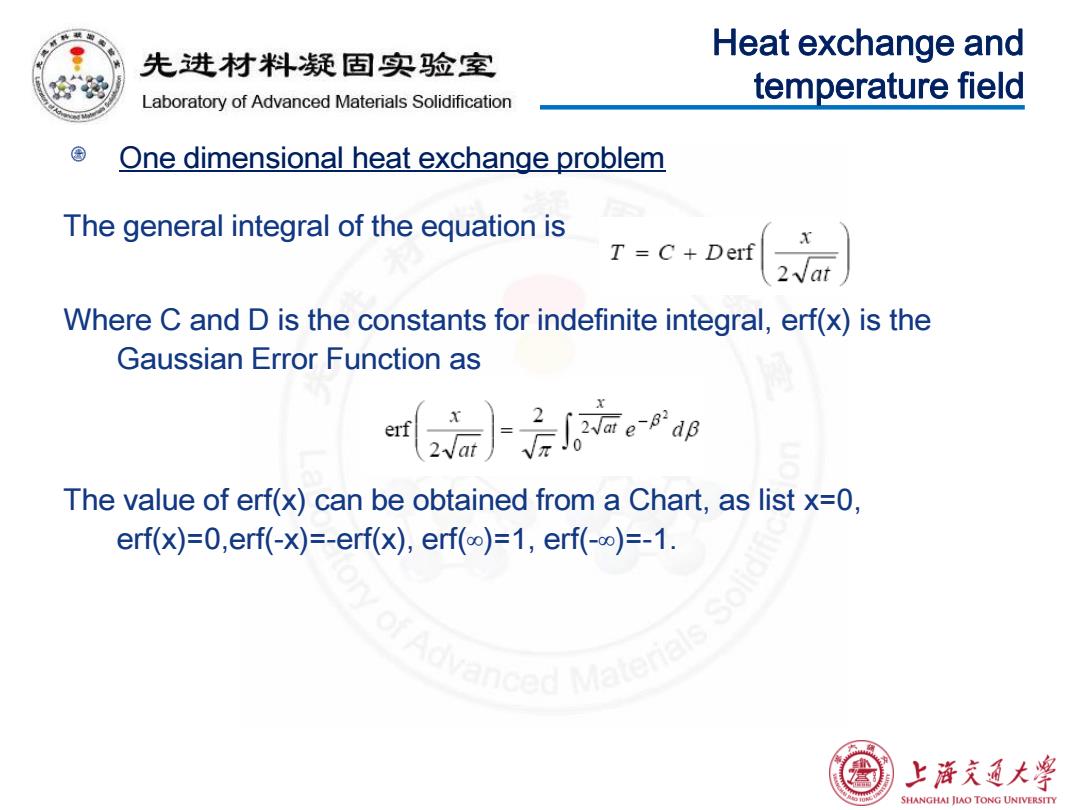
Heat exchange and 先进材料疑固实验室 Laboratory of Advanced Materials Solidification temperature field One dimensional heat exchange problem The general integral of the equation is T =C+Derf 2√at Where C and D is the constants for indefinite integral,erf(x)is the Gaussian Error Function as erf The value of erf(x)can be obtained from a Chart,as list x=0, erf(x)=0,erf(-x)=-erf(x),erf(co)=1,erf(-co)=-1. 上降充通大学 SHANGHAI JIAO TONG UNIVERSITY
The general integral of the equation is Where C and D is the constants for indefinite integral, erf(x) is the Gaussian Error Function as The value of erf(x) can be obtained from a Chart, as list x=0, erf(x)=0,erf(-x)=-erf(x), erf(∞)=1, erf(-∞)=-1. Heat exchange and temperature field One dimensional heat exchange problem
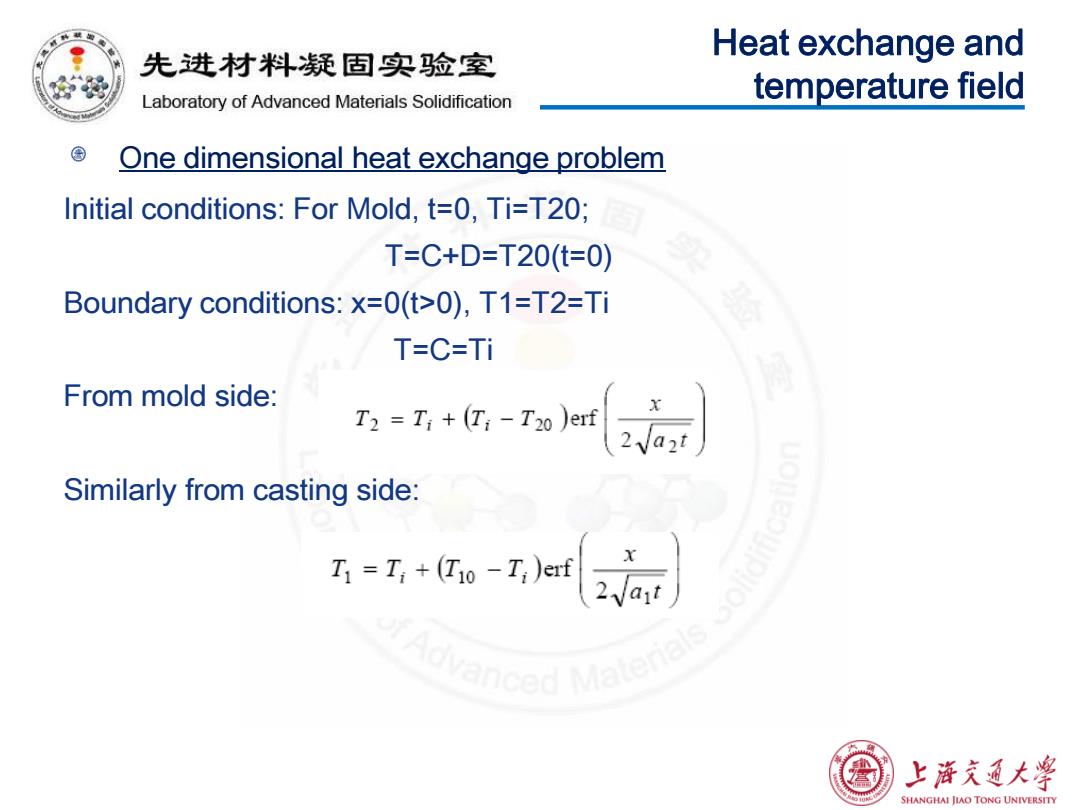
Heat exchange and 先进材料疑固实验室 Laboratory of Advanced Materials Solidification temperature field One dimensional heat exchange problem Initial conditions:For Mold,t=0,Ti=T20; T=C+D=T20(t=0) Boundary conditions:x=0(t>0),T1=T2=Ti T=C=Ti From mold side: T2 =T;+(T;-T20)erf Similarly from casting side: T1=T;+(T1o -T;)erf ced Mat 上降充通大学 SHANGHAI JIAO TONG UNIVERSITY
Initial conditions: For Mold, t=0, Ti=T20; T=C+D=T20(t=0) Boundary conditions: x=0(t>0), T1=T2=Ti T=C=Ti From mold side: Similarly from casting side: Heat exchange and temperature field One dimensional heat exchange problem

Heat exchange and 先进材料疑固实验室 Laboratory of Advanced Materials Solidification temperature field One dimensional heat exchange problem Then easily get: T-%0+a210 a1+Q2 (steady state) a and a corresponding to casting and mold. Substitute this equation to previous one ent va) C1+2 1+2 I+a+aerf(a T= 1+2 a1+a2 nced Materals 上降充通大学 SHANGHAI JIAO TONG UNIVERSITY
Then easily get: α1 and α2 corresponding to casting and mold. Substitute this equation to previous one Heat exchange and temperature field One dimensional heat exchange problem (steady state)