南京大学：《大学物理》课程教学资源（PPT讲座）Beam, beam, beam——无衍射光束的产生与调控

版 Nanjing University CEAS Beam,beam,Beam 无衍射光束的产生与调控 Tao Li(李涛) taoli@nju.edu.cn National Laboratory of Microstructures, College of Engineering and Applied Sciences, Nanjing University,China Nat.Lab.Microstructures Dr.Tao LI,http://dsl.nju.edu.cn/litao

Nanjing University CEAS Nat. Lab. Microstructures Dr. Tao LI, http://dsl.nju.edu.cn/litao Tao Li (李涛) taoli@nju.edu.cn National Laboratory of Microstructures, College of Engineering and Applied Sciences, Nanjing University, China Beam, beam, Beam

成 Nanjing University CEAS UNIVE Outline ▣Helmholtz Equation ▣Gaussian beam ▣Bessel beam Nondiffracting beams ▣Airy beam Mathieu and Weber beam 1 I Plasmonic counterpart 1 1 Nat.Lab.Microstructures Dr.Tao LI,http://dsl.nju.edu.cn/litao

Nanjing University CEAS Nat. Lab. Microstructures Dr. Tao LI, http://dsl.nju.edu.cn/litao  Helmholtz Equation  Gaussian beam  Bessel beam  Airy beam  Mathieu and Weber beam  Plasmonic counterpart Nondiffracting beams

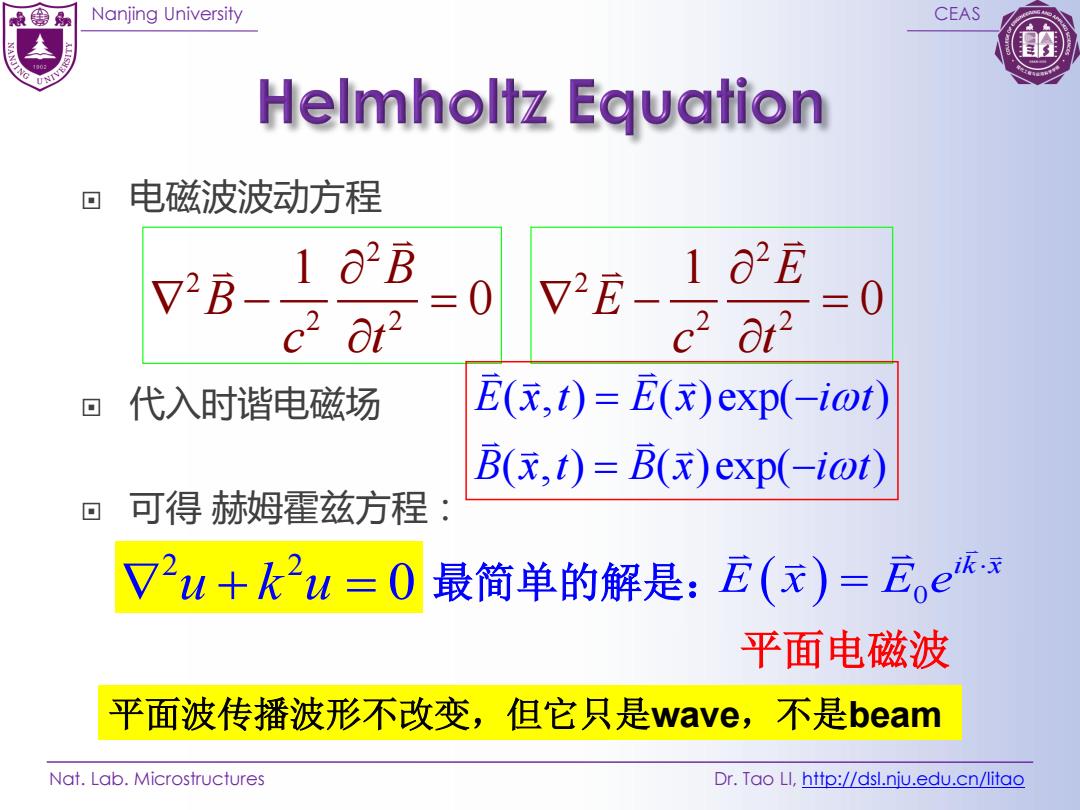
Nanjing University CEAS Helmholtz Equation 电磁波波动方程 72B、 1∂B 182E =0 0 c20t2 ▣代入时谐电磁场 E(,t)=E()exp(-iot) B(,t)=B()exp(-iot) ▣可得赫姆霍兹方程 V2u+k2u=0 最简单的解是：E()=E。ek 平面电磁波 平面波传播波形不改变，但它只是wave,不是beam Nat.Lab.Microstructures Dr.Tao LI,http://dsl.niu.edu.cn/litao

Nanjing University CEAS Nat. Lab. Microstructures Dr. Tao LI, http://dsl.nju.edu.cn/litao  电磁波波动方程  代入时谐电磁场  可得 赫姆霍兹方程： 2 2 2 2 1 0 B B c t      2 2 2 2 1 0 E E c t      ( , ) ( )exp( ) ( , ) ( )exp( ) E x t E x i t B x t B x i t       2 2    u k u 0 最简单的解是：   0 ik x E x E e   平面电磁波 平面波传播波形不改变，但它只是wave，不是beam

Nanjing University CEAS (1)Gaussian beam 亥姆霍兹方程的波束解 考虑波束能量分布具有轴对称性，中间场强最大 靠近边缘强度迅速衰减。在横载面上具有这种分 布性质的最简单函数就是高斯函数： 参数w表示光束的宽度 波束宽度通常还是z的函数 波幅通常也是z的函数，我们以(xy,z)代表电磁 场任意直角分量，它可以具有如下形式 u(x,y,z)=g(z)ete Nat.Lab.Microstructures Dr.Tao LI,http://dsl.niu.edu.cn/litao

Nanjing University CEAS Nat. Lab. Microstructures Dr. Tao LI, http://dsl.nju.edu.cn/litao 考虑波束能量分布具有轴对称性，中间场强最大， 靠近边缘强度迅速衰减。在横截面上具有这种分 布性质的最简单函数就是高斯函数： 亥姆霍兹方程的波束解 2 2 2 x y w e   参数w表示光束的宽度 波束宽度通常还是z的函数 波幅通常也是z的函数，我们以u(x,y,z)代表电磁 场任意直角分量，它可以具有如下形式 2 2 ( )( ) ( , , ) ( ) f z x y ikz u x y z g z e e   

Nanjing University CEAS u(x,y,z)=g(z)e-r(=Xx'+y)e 式中e表示以来与z的主要因子，剩下的因子中 还有对z的缓变函数g(z)和z), 因子fr+)是限制波束的空间宽度的因子。 因子g(z)主要表示波的振幅，同时也含有传播因 子中与纯平面因子e偏离的部分。令 (x,y,2)=8(z)ef(er+y2) 它满足z的缓变振幅近似。 因此它对z的展开式中 的高次项可以忽略。 Nat.Lab.Microstructures Dr.Tao LI,http://dsl.nju.edu.cn/litao

Nanjing University CEAS Nat. Lab. Microstructures Dr. Tao LI, http://dsl.nju.edu.cn/litao 式中 表示以来不z的主要因子，剩下的因子中 还有对z的缓变函数g(z)和f(z)， 因子 是限制波束的空间宽度的因子。 因子g(z)主要表示波的振幅，同时也含有传播因 子中与纯平面因子 偏离的部分。令 2 2 ( )( ) ( , , ) ( ) f z x y  x y z g z e    2 2 ( )( ) ( , , ) ( ) f z x y ikz u x y z g z e e    ikz e 2 2 f z x y ( )( ) e   ikz e 它满足z的缓变振幅近似。因此它对z的展开式中 的高次项可以忽略