西安电子科技大学：《场论与复变函数》课程教学资源（课件讲义）第四章 级数 Series

第四章级数 历些毛子代枚大学 XIDIAN UNIVERSITY Series 定理1（数列收敛的充要条件） 数列{an}收敛于a台lima=a,limb=b 00 定理一说明：可将复数列的敛散性转化为 判别两个实数列的敛散性： 场论与复变函数Field Theory and Complex Variable Functions

场论与复变函数 Field Theory and Complex Variable Functions 7 数列 收敛于  n n n  n n α α lima a, lim b b      定理1(数列收敛的充要条件) 定理一说明: 可将复数列的敛散性转化为 判别两个实数列的敛散性. 第四章 级数 Series

第四章级数 历些毛子代枚大学 XIDIAN UNIVERSITY Series 课堂练习： 下列数列是否收敛？如果收敛，求出其极限 1+i (1)zm= 1-i (2)zn=(-1)”+i +1 (4)zn=e2'; (5)zn= 2 n 场论与复变函数Field Theory and Complex Variable Functions

场论与复变函数 Field Theory and Complex Variable Functions 8 课堂练习: 下列数列是否收敛? 如果收敛, 求出其极限. ; 1 1 (1) ni ni zn    ; 1 (2) ( 1)     n i z n n 2 1 (5) . n i n z e n    (3) (1 ) . 3 n n i z    2 (4) ; n i n z e    第四章 级数 Series

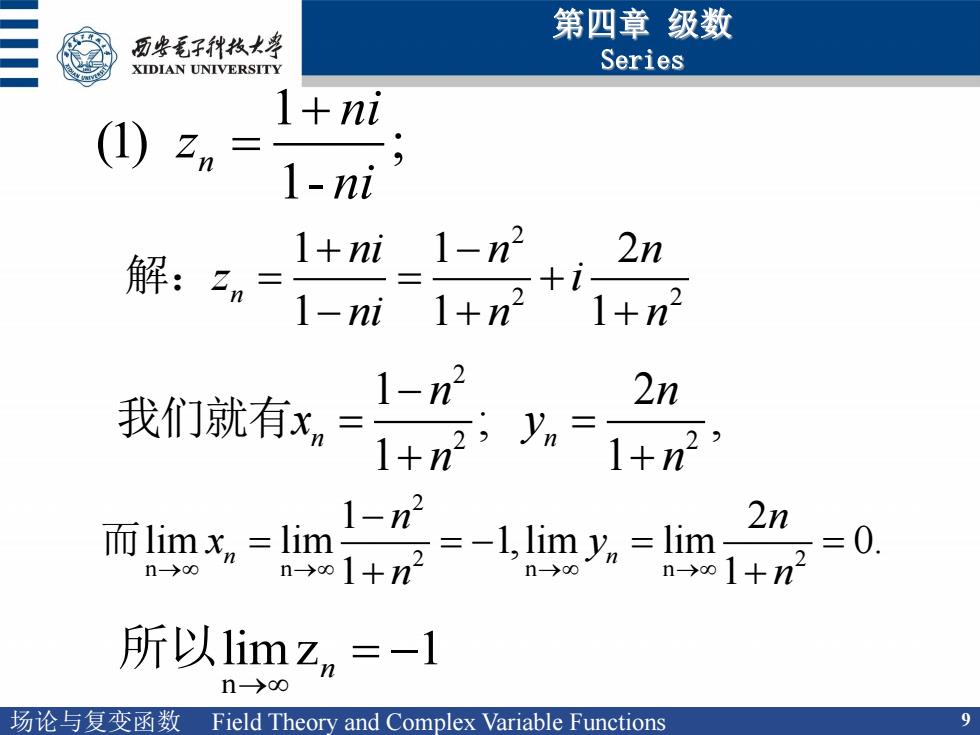
第四章级数 历些毛子代枚大票 XIDIAN UNIVERSITY Series I+ni (1) 2n 二 1-ni 2n 解：2n= 1+ni 1-n2 1-ni i++ 1+n 1-n2 我们就有xn 2n 1+ 1+n 1-n2 n 而limx,=lim 2 =-Llimy lim. =0 n→0 n-→o1+n n1+n2 所以1imzn=-l n>∞ 场论与复变函数Field Theory and Complex Variable Functions 9

场论与复变函数 Field Theory and Complex Variable Functions 9 2 2 2 1 1 2 1 1 1         n ni n n z i ni n n 解： 1 (1) ; 1 - n ni z ni   2 2 2 1 2 ; , 1 1      n n n n x y n n 我们就有 2 2 2 n n n n 1 2 lim lim 1,lim lim 0.     1 1         n n n n x y n n 而 n lim z 1 n  所以   第四章 级数 Series

第四章级数 历安毛子代枚大等 XIDIAN UNIVERSITY Series (2)zm=(-1)”+ n+ (3)n=(1+ -n 解9+ can eos(-名)+isin(-gxl 场论与复变函数Field Theory and Complex Variable Functions 10

场论与复变函数 Field Theory and Complex Variable Functions 10 (3) (1 ) . 3 n n i z   3 3 1 z ( ) 2 2 2 n n n i            解： 3 (cos sin ) 2 6 6 n n i              3 [cos( ) sin( )] 2 6 6 n n n   i             ; 1 (2) ( 1)     n i z n n 第四章 级数 Series

第四章级数 历安毛子代枚大学 XIDIAN UNIVERSITY Series 1 (3) 乙n=(1+ m,9r-98agn limx=0,lim y =0 n0 n->oc →limz=0, n→o 场论与复变函数Field Theory and Complex Variable Functions 11

场论与复变函数 Field Theory and Complex Variable Functions 11 (3) (1 ) . 3 n n i z   3 3 1 z ( ) 2 2 2 n n n i            解： 3 [cos( ) sin( )] 2 6 6 n n n   i             3 3 ( ) cos , ( ) sin 2 6 2 6 n n n n n n     x y   n n lim 0, lim 0 n n x y     n lim z 0, n    第四章 级数 Series