
先进材料疑固实验室 J-H model for regular eutectic Laboratory of Advanced Materials Solidification From the phase diagram,the corresponding solid composition Ca Ce,liquid composition L+a L L+B CaL and CeL have the following relationship: C BM 会k1 Ce =kg>1 A Ca CBL CE CaL B CB/% nced Matere 上浒充通大 SHANGHAI JIAO TONG UNIVERSITY
J-H model for regular eutectic From the phase diagram, the corresponding solid composition Cα, Cβ, liquid composition CαL and CβL have the following relationship:
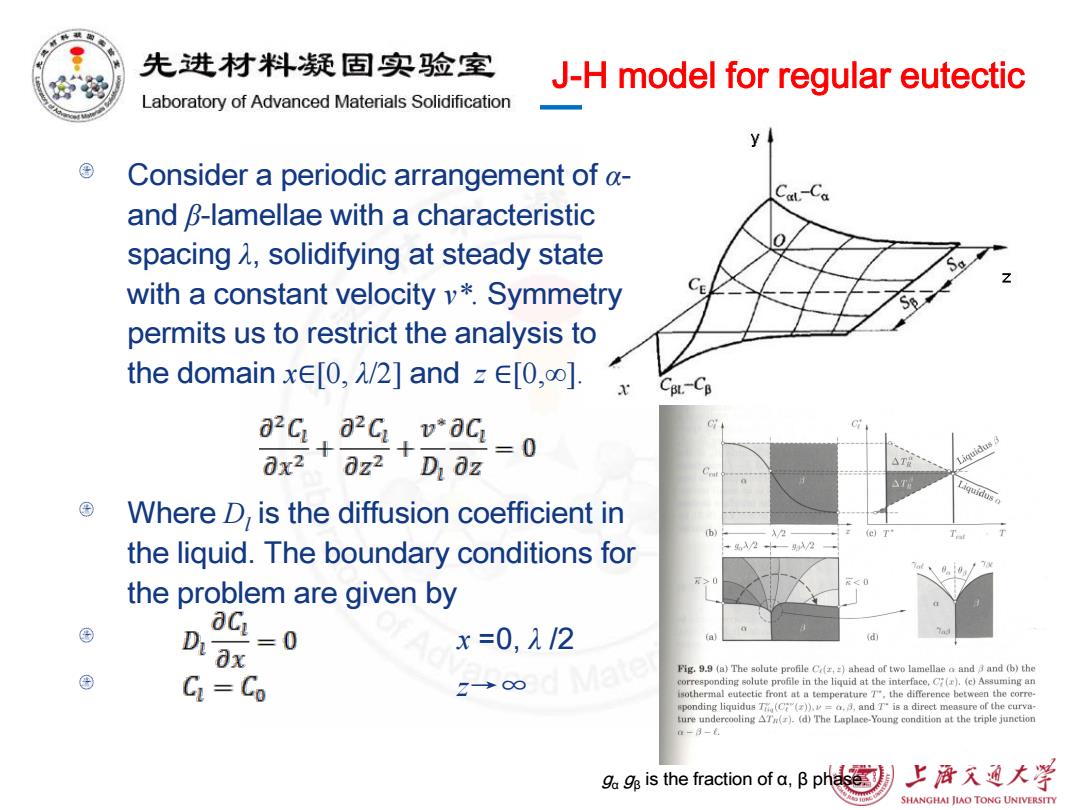
先进材料疑固实验室 J-H model for regular eutectic Laboratory of Advanced Materials Solidification Consider a periodic arrangement of a- C and B-lamellae with a characteristic spacing 1,solidifying at steady state with a constant velocity v*.Symmetry CE permits us to restrict the analysis to the domain xE[0,/2]andz∈[0,ool. CBL.-CB 02C 02C v0Gi 0x20z2 D0z 0 △T Where D,is the diffusion coefficient in Ib) the liquid.The boundary conditions for +%入/2十—/2 the problem are given by ⊕ O 20 Dia x=0,1/2 d Fig.9.9 (a)The solute profile C(r,:ahead of two lamellae a and 3 and (b)the ⊕ CL=Co corresponding solute profile in the liquid at the interface,Ci(r).(c)Assuming an isothermal eutectie front at a temperature T.the difference between the corre ponding liquidus T(C()).=o.3,and T is a direct measure of the curva ture undercooling ATn(r).(d)The Laplace-Young condition at the triple junction a-3-t. ggs is the fraction of a,B phase. 上酒天通大学 SHANGHAI JIAO TONG UNIVERSITY
J-H model for regular eutectic Consider a periodic arrangement of α- and β-lamellae with a characteristic spacing λ, solidifying at steady state with a constant velocity ν*. Symmetry permits us to restrict the analysis to the domain x∈[0, λ/2] and z ∈[0,∞]. Where Dl is the diffusion coefficient in the liquid. The boundary conditions for the problem are given by x =0, λ /2 z→∞ gα gβ is the fraction of α, β phase
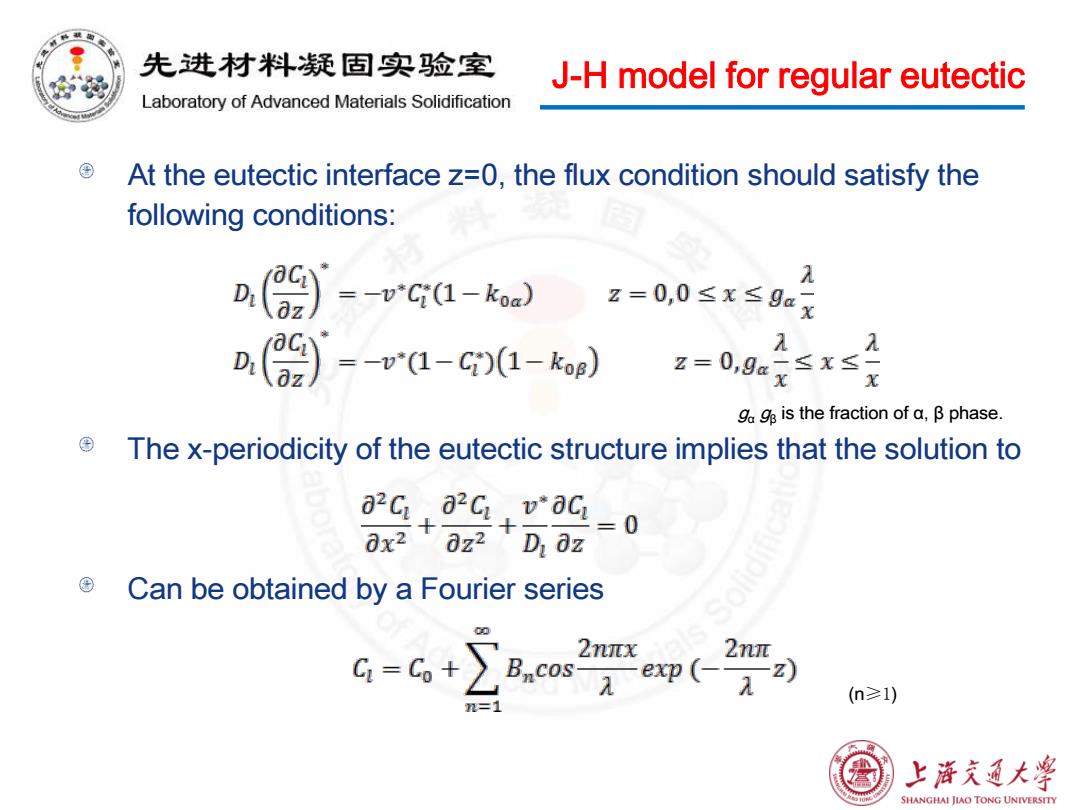
种 先进材料疑固实验室 J-H model for regular eutectic Laboratory of Advanced Materials Solidification At the eutectic interface z=0,the flux condition should satisfy the following conditions: (az) =-v*Ci(1-koa) z=0,0≤x≤9ax (9)-p1-G1-kag) z=0,9x≤x≤ X gge is the fraction of a,B phase. The x-periodicity of the eutectic structure implies that the solution to 02C02C vOCL D oz 20 Can be obtained by a Fourier series G=C+∑B,cos元e 2πX 2mπ (n≥1) 1=1 熟 上浒充通大学 SHANGHAI JIAO TONG UNIVERSITY
J-H model for regular eutectic At the eutectic interface z=0, the flux condition should satisfy the following conditions: The x-periodicity of the eutectic structure implies that the solution to Can be obtained by a Fourier series gα gβ is the fraction of α, β phase. (n≥1)