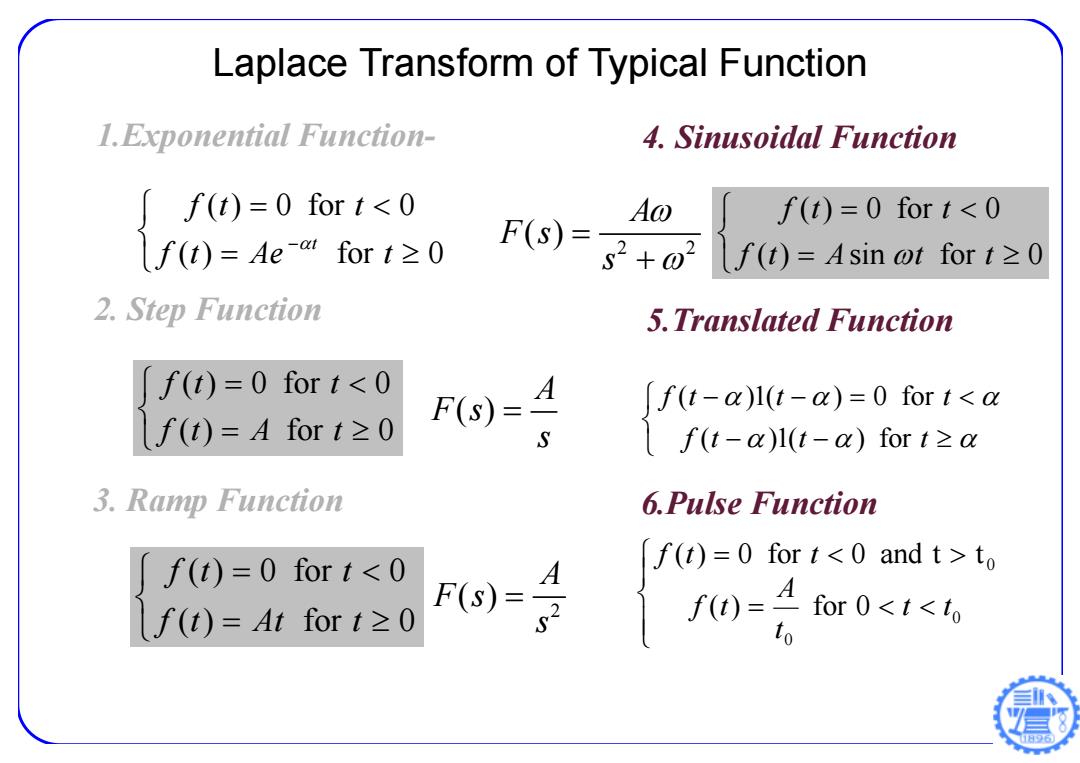
Laplace Transform of Typical Function 1.Exponential Function- 4.Sinusoidal Function [f(t)=0 for t<0 Aw f(t)=0 for t<0 f(t)=Ae-for t≥0 F(s)=g2+o2 f(t)=Asin ot for t≥0 2.Step Function 5.Translated Function f(t)=0 for t<0 f(t)=A for t≥0 F()-4 f(t-a)1(t-a)=0 for t<a lf(t-)l(t-a)fort≥a 3.Ramp Function 6.Pulse Function f(t)=0 for t<0 f(t)=0 for t<0 and t>to A f(t)=At for t≥0 F(s)= f0=or0<1<1, to
Laplace Transform of Typical Function 1.Exponential Function- ( ) for 0 ( ) 0 for 0 f t Ae t f t t t 2. Step Function ( ) for 0 ( ) 0 for 0 f t A t f t t 3. Ramp Function ( ) for 0 ( ) 0 for 0 f t At t f t t ( ) sin for 0 ( ) 0 for 0 f t A t t f t t 4. Sinusoidal Function 5.Translated Function f t t t f t t t ( ) 1 ( ) for ( ) 1 ( ) 0 for 6.Pulse Function 0 0 0 ( ) for 0 ( ) 0 for 0 and t t t t t A f t f t t ( ) A F s s 2 ( ) A F s s 2 2 ( ) A F s s
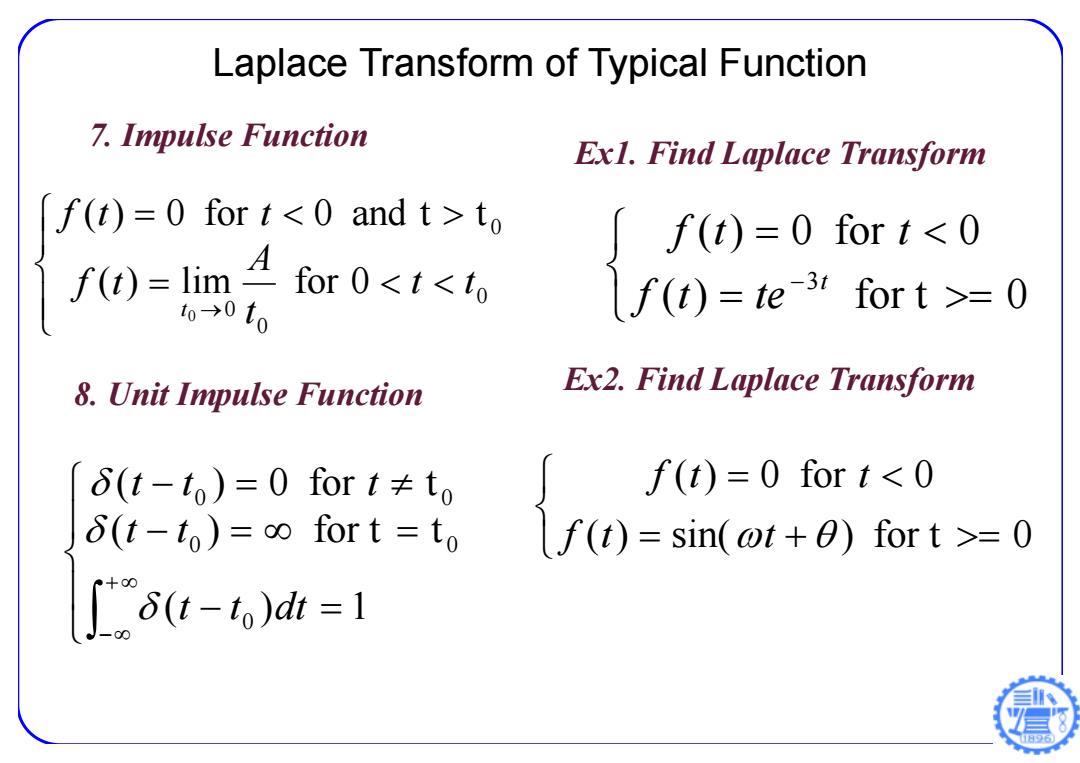
Laplace Transform of Typical Function 7.Impulse Function Ex1.Find Laplace Transform f(t)=0 for t<0 and t>to f(t)=0 for t<0 f()=lim for to→0t0 f(t)=te-3 fort>=0 8.Unit Impulse Function Ex2.Find Laplace Transform 6(t-to)=0fort≠to f(1)=0 fort<0 δ(t-to)=o fort=to f(t)=sin(ot+0)fort>=0 δ(t-to)dt=1
Laplace Transform of Typical Function 7. Impulse Function 8. Unit Impulse Function 0 0 0 0 ( ) lim for 0 ( ) 0 for 0 and t t 0 t t t A f t f t t t ( ) 1 ( ) for t t ( ) 0 for t 0 0 0 0 0 t t dt t t t t t ( ) for t 0 ( ) 0 for 0 3 t f t te f t t Ex1. Find Laplace Transform Ex2. Find Laplace Transform ( ) sin( ) for t 0 ( ) 0 for 0 f t t f t t