上海交通大学：《现代控制理论》课程教学资源（讲稿）Chapter 6 Controllability and Observability

Chapter 6 12 Example:Determine the controllability of following systems using alternative method. -7 ●0 (1) x- 1 (2) 2 Solution: (1)Uncontrollable. (2)Controllable

12 Example: Determine the controllability of following systems using alternative method. u                          9 0 2 0 1 5 7 0 x x u                          7 5 4 0 0 1 0 1 5 7 0 x x （1） （2） Solution: (1) Uncontrollable. (2) Controllable. Chapter 6

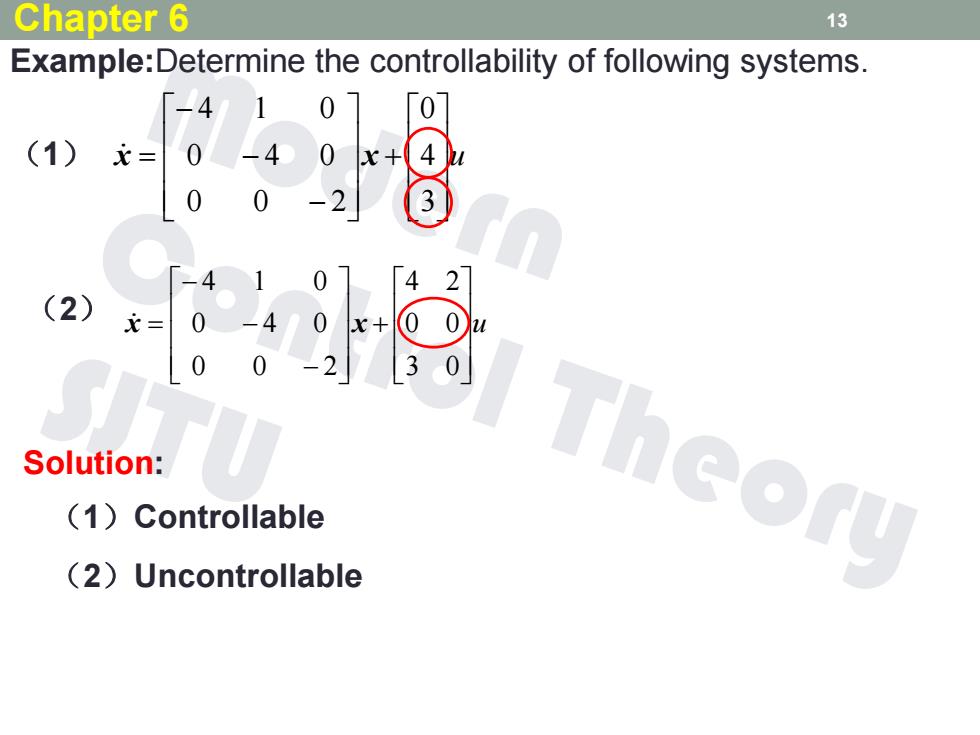
Chapter 6 13 Example:Determine the controllability of following systems. -41 0 (1)x=0 -4 0 0 -2 3 -4 2 (2) 文= 0 -4 0 0 -2 0 Solution: (1)Controllable Theory (2)Uncontrollable

13 Example:Determine the controllability of following systems. u                          3 4 0 0 0 2 0 4 0 4 1 0 x x u                          3 0 0 0 4 2 0 0 2 0 4 0 4 1 0 x x （1） （2） Solution: （1）Controllable （2）Uncontrollable Chapter 6

Chapter 6 14 6.2 Observability 。Definition: A linear state system or pair (A,C)is said to be observable if for any unknown initial state x(0),there exist a finite t>0 such that the acknowledge of input u and output y over [0,t]suffices to determine uniquely the initial state x(0) Otherwise the system is said to be unobservable

6.2 Observability • Definition: • A linear state system or pair (A,C) is said to be observable if for any unknown initial state x(0), there exist a finite t1>0 such that the acknowledge of input u and output y over [0, t1 ] suffices to determine uniquely the initial state x(0). Otherwise the system is said to be unobservable. Chapter 6 14

Chapter 6 15 Example:Unobservable network -2 1 1H 12 x() y=Cx=[1-1kx →e= e+e-3 e--e-3r 10 etR次 e+e-3 F→0=ew0+e4-bu-x)dr then u(t)=0 x(t)=e4 x(0) Theory → y(t)=Ce"x(0)=[x(0)-x,(O]e31 The value of output only determined by the difference of initial states.Therefore,the states are unobservable

Example: Unobservable network 15 y Cx  x x u - - x Ax Bu 1 1 0 1 1 2 2 1                                        t t t t t t t t t 3 3 3 3 e e e e e e e e 2 1 e A t u t τ τ t τ t t ( ) e (0) e ( )d ( ) 0      x x b A A u(t)  0 • If then x( ) e x(0) At t  t t y t x x 3 1 2 ( ) e (0) [ (0) (0)]e   C x   A • The value of output only determined by the difference of initial states. Therefore, the states are unobservable. Chapter 6

Chapter 6 16 Theorem:The following statements are equivalent. 1.The n-dimensional pair(A,C)is observable 2.The following nxn matrix is nonsingular for any t>0. W(-eC"Ce"d 3 The ngxn observability matrix has rank n full column rank) CA 2= CA"-1

• Theorem: The following statements are equivalent. 1. The n-dimensional pair (A,C) is observable. 2. The following nn matrix is nonsingular for any t>0. 3. The nqn observability matrix has rank n ( full column rank) 16     t A τ T Aτ WC (t) e C Ce dτ T 0 nm n n                1 CA CA C QO  Chapter 6