
Chapter 3 2 Chapter 3 Linear algebra Objectives: ·Mathematic review Concepts,laws and rules ·Linear algebra rol Theory Computation of exponential matrix
Chapter 3 Linear algebra Objectives: • Mathematic review • Concepts, laws and rules • Linear algebra • Computation of exponential matrix Chapter 3 2
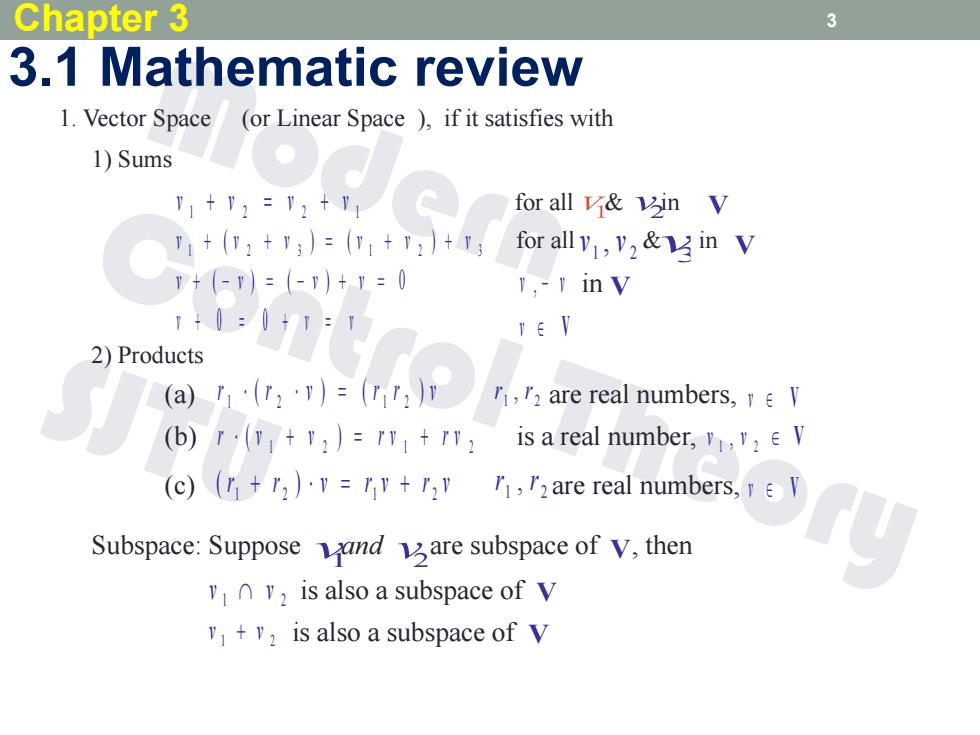
Chapter 3 3 3.1 Mathematic review 1.Vector Space (or Linear Space )if it satisfies with 1)Sums "1+1=1+ for all&in V (:+;)=(+:)+T;for allv,vin V 1+(-)=(-)+1:0 1,-I inV 1+0:01:1 re V 2)Products (a)1(r:)=(n n1,"2 are real numbers,V (b)r ()=r+r:isareal number,:e V (c)(2)=+1,rare real numbers,V Subspace:Suppose andare subspace of V,then v2 is also a subspace of V +2 is also a subspace of V
1. Vector Space (or Linear Space ), if it satisfies with 1) Sums 1 2 2 1 v v v v 1 2 3 1 2 3 v ( v v ) ( v v ) v v ( v ) ( v ) v 0 v 0 0 v v for all & in v1 v2 V for all & in v1 , v 2 v3 V v , v in V v V 2) Products (a) r ( r v ) ( r r ) v are real numbers, 1 2 1 2 1 2 r , r v V (b) is a real number, 1 2 1 2 r ( v v ) r v r v v 1 , v 2 V r r v r v r v 1 2 1 2 (c) ( ) 1 2 are real numbers, r , r v V Subspace: Suppose and are subspace of , then v1 v2 V v 1 v 2 is also a subspace of V v 1 v 2 is also a subspace of V Chapter 3 3 3.1 Mathematic review

Chapter 3 4 2.Inner Products of 下&W Suppose =[2&w=, vw=W,w〉=[,2yn =w,+w2+ty,w,=∑,w, two vectors are orthogonal (or perpendicular)if<v,w >=vw=0 3.Outer Product of V W V W2 … VWn V2 V2W1 V2W2 V2Wn VW = w,..- VnW2 vnwn
v & w 2. Inner Products of 3. Outer Product of v & w Suppose & , T n [ v , v , , v ] v 1 2 T w w w n [ , , , ] w 1 2 v V , w W n i n n i i n n T v w v w v w v w w w w v v v 1 1 1 2 2 2 1 1 2 ... ... v w v, w , ,... two vectors are orthogonal (or perpendicular) if v & w v , w v w 0 T n n n n n n n n v w v w v w v w v w v w v w v w v w w w w v v v T ... ... ... ... ... ... ... , ,... ... 1 2 2 1 2 2 2 1 1 1 2 1 1 2 2 1 v w Chapter 3 4

Chapter 3 5 4.Euclidean Norm of Vector where v=,Y The geometrical meaning 10,0,30) cory
4. Euclidean Norm of Vector 2 1 1 2 n i i v v v v 2 n 2 2 2 1 v v , v where . T n [ v , v , , v ] v 1 2 v V The geometrical meaning v1 v3 v2 v ( , , ) 1 0 2 0 3 0 v v v v Chapter 3 5

Chapter 3 6 5.The distance of x&y awg-小-区g-j月 I[x2,,i,]'&y=1J1,…,]',ieV,】eV SJTu cor Obviously,d(x,y)=0,if and only if =y
5. The distance of x & y 2 1 1 2 ( ) ( ) n i i i d x , y x y x - y , x - y x y x [ 1 , 2 , , ] & y [ 1 , 2 , , ] , x V , y V T n T n x x x y y y Obviously, d ( x , y ) 0 , i f a n d o n ly i f x y y d ( x , y ) Chapter 3 6