上海交通大学：《现代控制理论》课程教学资源（讲稿）Chapter 6 Controllability and Observability

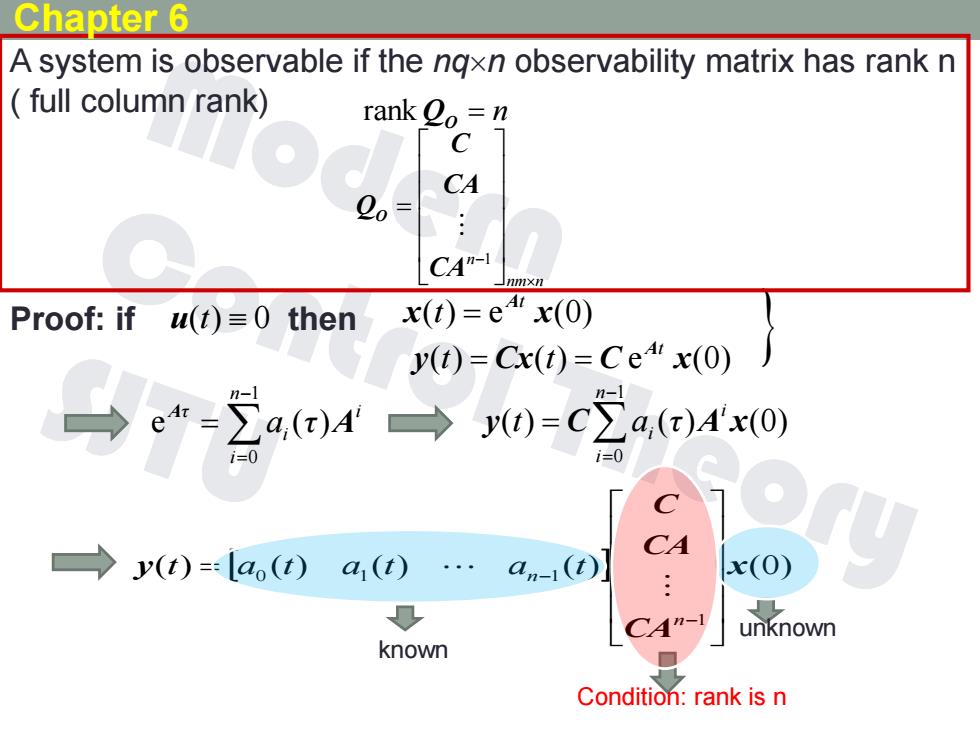
Chapter 6 A system is observable if the ngxn observability matrix has rank n full column rank) rank 0o n C CA 00- : CA-1 nmxn Proof:if u()=0 then x(t)=e4x(0) y(t)=Cx(t)=Ce x(0) n n-l e 4r ∑a,()A→y(0=C∑a,()Ax(0) i= i=0 →y(t)=la(t)a(t) a-1(t) x(0) ↓ CA"- unknown known Condition:rank is n

A system is observable if the nqn observability matrix has rank n ( full column rank) nm n n                1 CA CA C QO  rank QO  n Proof: if then u(t)  0 y( ) Cx( ) C e x(0) At t  t  x( ) e x(0) At t       1 0 e ( ) n i i i τ a τ A A ( ) ( ) (0) 1 0 y C A x    n i i i t a τ ( )  ( ) ( ) ( ) (0) 1 0 1 1 x CA CA C y                n n t a t a t a t   known Condition: rank is n unknown Chapter 6

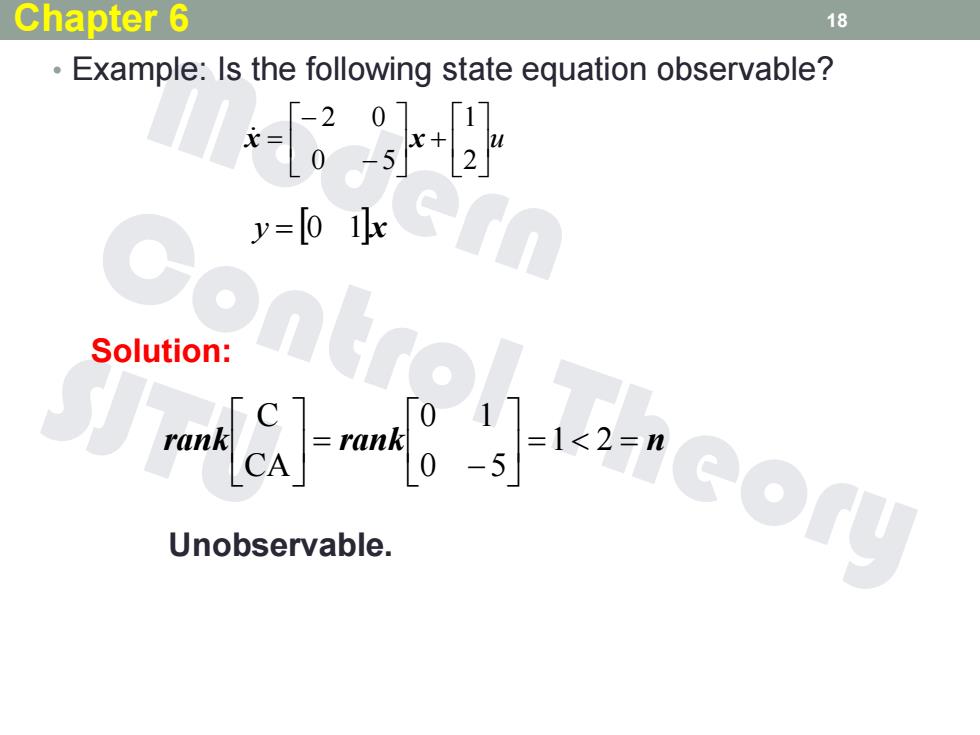
Chapter 6 18 Example:Is the following state equation observable? y=[0x Solution: rank Unobservable

• Example: Is the following state equation observable? 18 u                2 1 0 5 2 0 x x y  0 1x Solution: rank rank    n              1 2 0 5 0 1 CA C Unobservable. Chapter 6