
Bubble growth and collapse
Bubble growth and collapse

Introduction Gas bubbles can grow or collapse in flow Introducing many important phenomena General assumptions in this chapter Flow far away from bubble is at rest -Spherical symmetric bubble Single bubble
Introduction • Gas bubbles can grow or collapse in flow • Introducing many important phenomena • General assumptions in this chapter – Flow far away from bubble is at rest – Spherical symmetric bubble – Single bubble
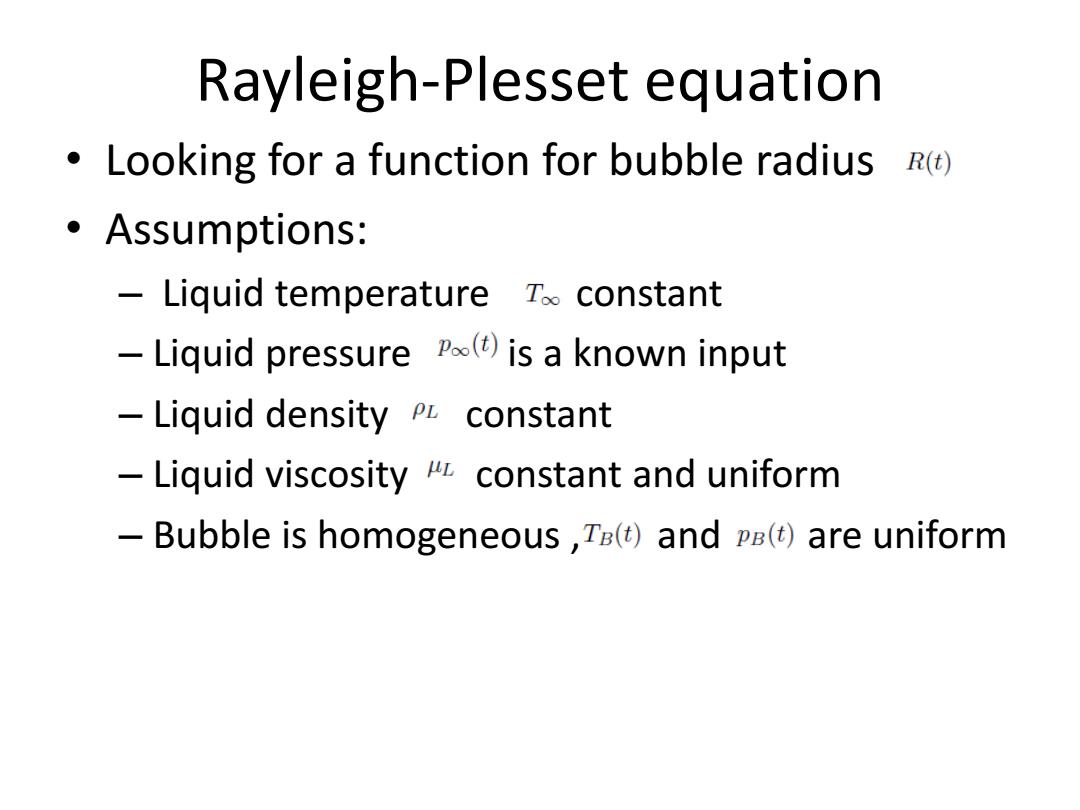
Rayleigh-Plesset equation Looking for a function for bubble radius R(t) ·Assumptions: Liquid temperature Too constant Liquid pressure Po()is a known input Liquid density pL constant Liquid viscosity constant and uniform Bubble is homogeneous,Ta(t)and pE()are uniform
Rayleigh-Plesset equation • Looking for a function for bubble radius • Assumptions: – Liquid temperature constant – Liquid pressure is a known input – Liquid density constant – Liquid viscosity constant and uniform – Bubble is homogeneous , and are uniform
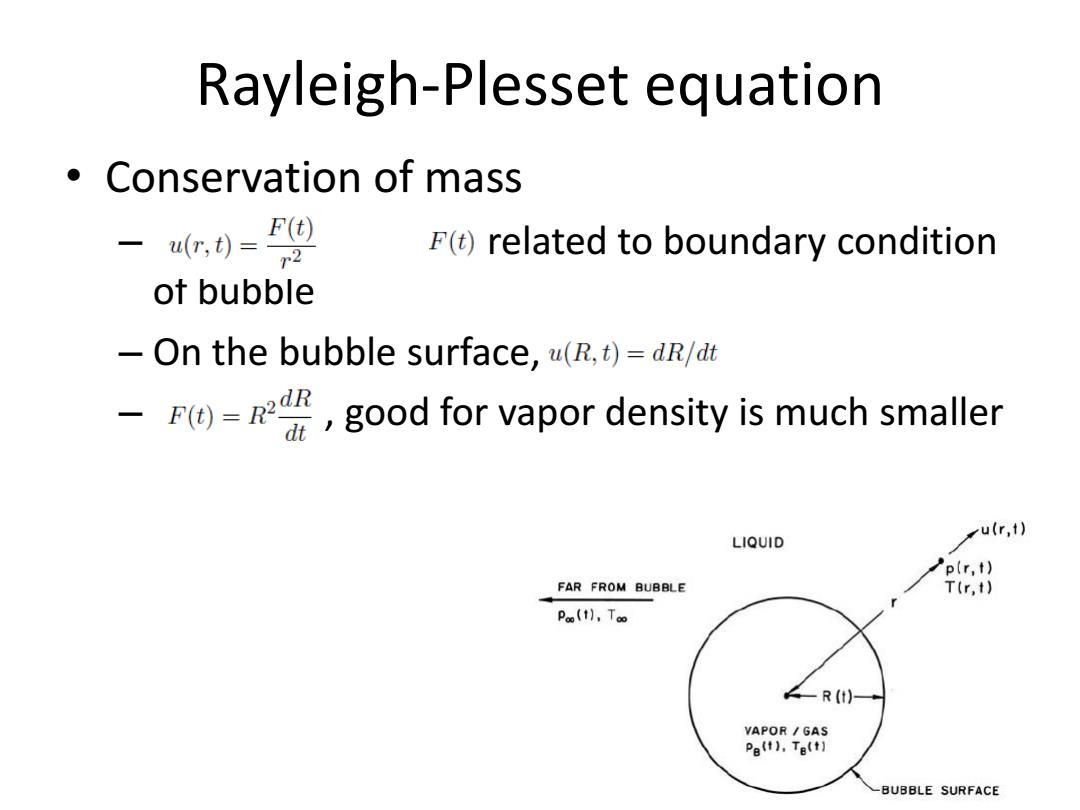
Rayleigh-Plesset equation 。Conservation of mass -4(,)=F F()related to boundary condition of bubble -On the bubble surface,u(R,t)=dR/dt -good for vapor density is much smaller u(r,t) LIQUID p(r,t) FAR FROM BUBBLE T(r,t) Poo(t],Too ∠-R(t)+ VAPOR GAS Pe(t).Te(t] -BUBBLE SURFACE
Rayleigh-Plesset equation • Conservation of mass – related to boundary condition of bubble – On the bubble surface, – , good for vapor density is much smaller

Rayleigh-Plesset equation N-S equation in r direction 1∂p0u,0u pLar=t+“ar {品 ·Introducing u=F(t)/r2 1 Op 1 dF 2F2 PL Orr2 dt r5 ·Integrate to get p-Poo 1dF 1F2 PL r dt 2r4 Net force on the surface radially outward 2S (Orr)r=R+PB-R Orr =-p+2uLou/Or. AuL dR PB -(p)r-R-R dt 2S replacing (p),=R R pB(t)-p∞() d2R 3 dR 2 AvL dR 2S PL -2 dt R dt PLR
Rayleigh-Plesset equation • N-S equation in r direction • Introducing • Integrate to get • Net force on the surface radially outward • replacing