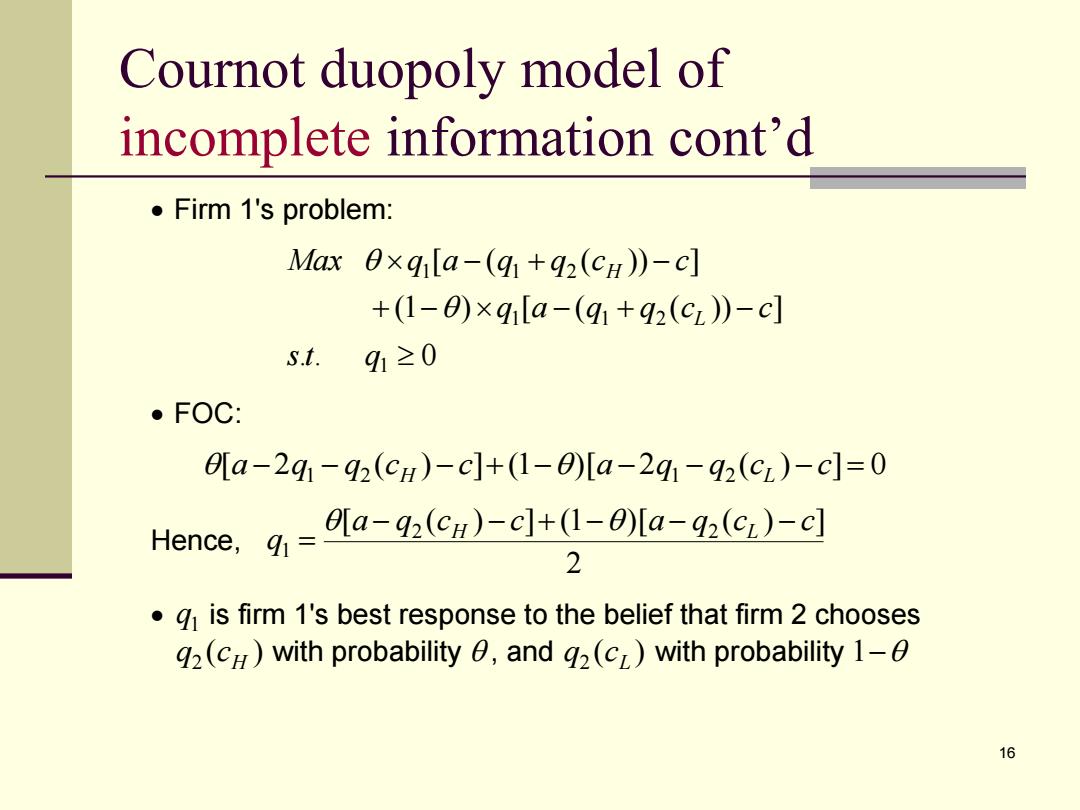
Cournot duopoly model of incomplete information cont'd Firm 1's problem: MaxB×q1[a-(91+92(cH)-c] +(1-θ)×q1[a-(q1+q2(c)-c] s.t. 91≥0 ·FOC: 0L[a-2q1-92(cH)-c]+(1-0)[a-2q1-92(cz)-c]=0 Hence.g=Ola-q(Cu)-c]+(1-O)a-gz(C;)-c] 2 g is firm 1's best response to the belief that firm 2 chooses g2(CH)with probability 0,and g2(cL)with probability 1-0 16
16 Cournot duopoly model of incomplete information cont’d Firm 1's problem: . . 0 (1 ) [ ( ( )) ] [ ( ( )) ] 1 1 1 2 1 1 2 st q q a q q c c Max q a q q c c L H FOC: [a 2q1 q2 (cH ) c] (1)[a 2q1 q2 (cL ) c] 0 Hence, 2 [ ( ) ] (1 )[ ( ) ] 2 2 1 a q c c a q c c q H L 1 q is firm 1's best response to the belief that firm 2 chooses ( ) 2 H q c with probability , and ( ) 2 L q c with probability 1
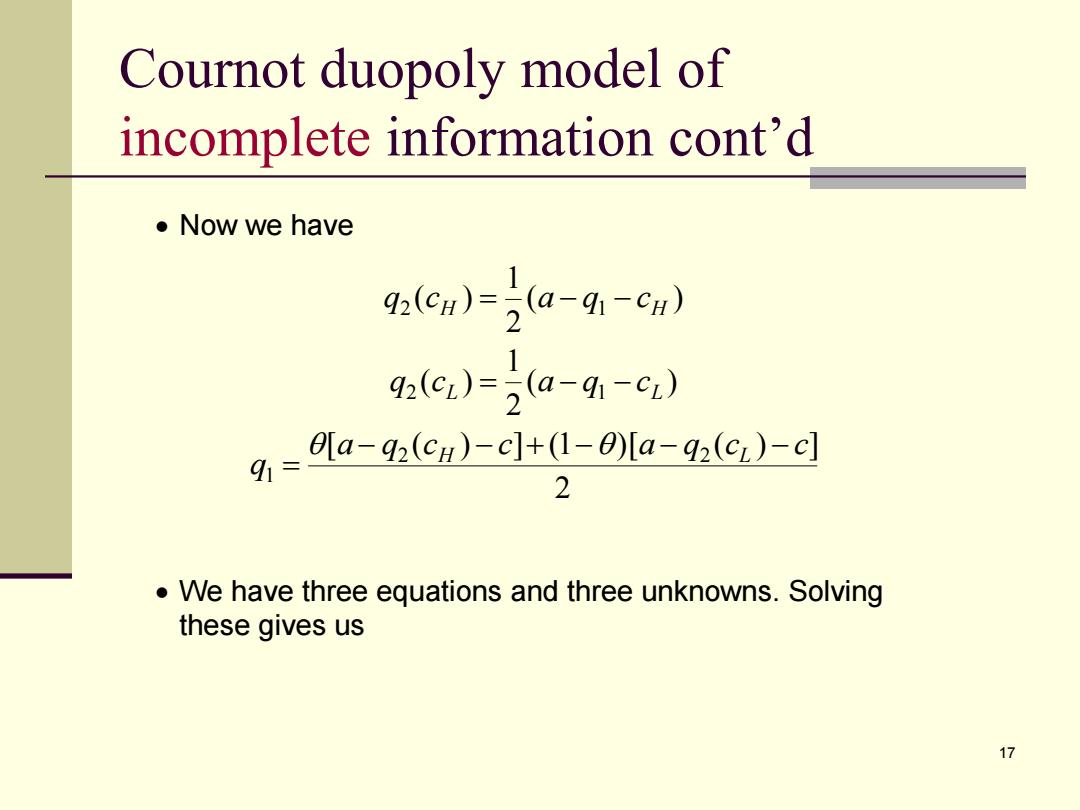
Cournot duopoly model of incomplete information cont'd ●Now we have Q:(cn)-j(a-q-ew) 9s(e)=a--c) 9,=0La-9cH)-c+1-0[a-92(G)-d 2 We have three equations and three unknowns.Solving these gives us 17
17 Cournot duopoly model of incomplete information cont’d Now we have ( ) 2 1 ( ) 2 H 1 H q c a q c ( ) 2 1 ( ) 2 L 1 L q c a q c 2 [ ( ) ] (1 )[ ( ) ] 2 2 1 a q c c a q c c q H L We have three equations and three unknowns. Solving these gives us
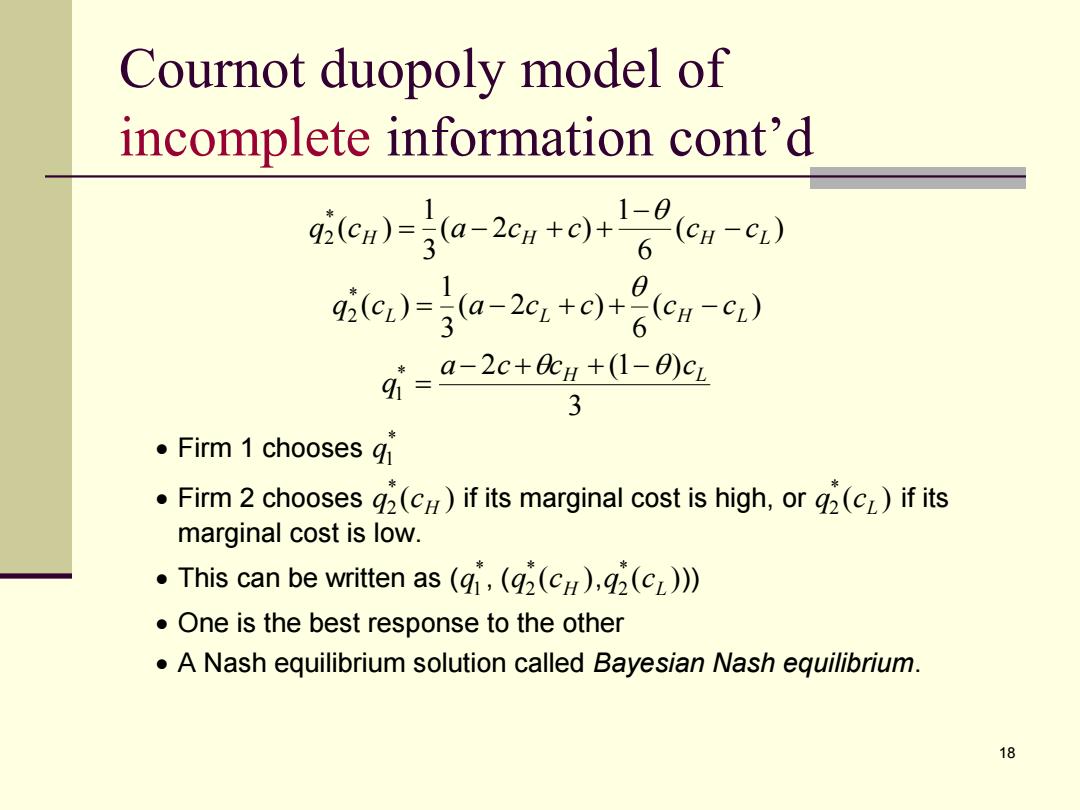
Cournot duopoly model of incomplete information cont'd 1- 9i(cH)=3a-2cH+c)+6 (cH-c) 98e)=5a-2c+o)+gem-c) g=a-2c+比1+0-)e2 3 ·Firm1 chooses q Firm 2 chooses g2(cH)if its marginal cost is high,or g2(cL)if its marginal cost is low. This can be written as (qi,(g2(cH),2(c))) One is the best response to the other A Nash equilibrium solution called Bayesian Nash equilibrium 18
18 Cournot duopoly model of incomplete information cont’d ( ) 6 1 ( 2 ) 3 1 ( ) * 2 H H H L q c a c c c c ( ) 6 ( 2 ) 3 1 ( ) * 2 L L H L q c a c c c c 3 * 2 (1 ) 1 H L a c c c q Firm 1 chooses * 1q Firm 2 chooses ( ) * 2 H q c if its marginal cost is high, or ( ) * 2 L q c if its marginal cost is low. This can be written as ( * 1q , ( ( ) * 2 H q c , ( ) * 2 L q c )) One is the best response to the other A Nash equilibrium solution called Bayesian Nash equilibrium
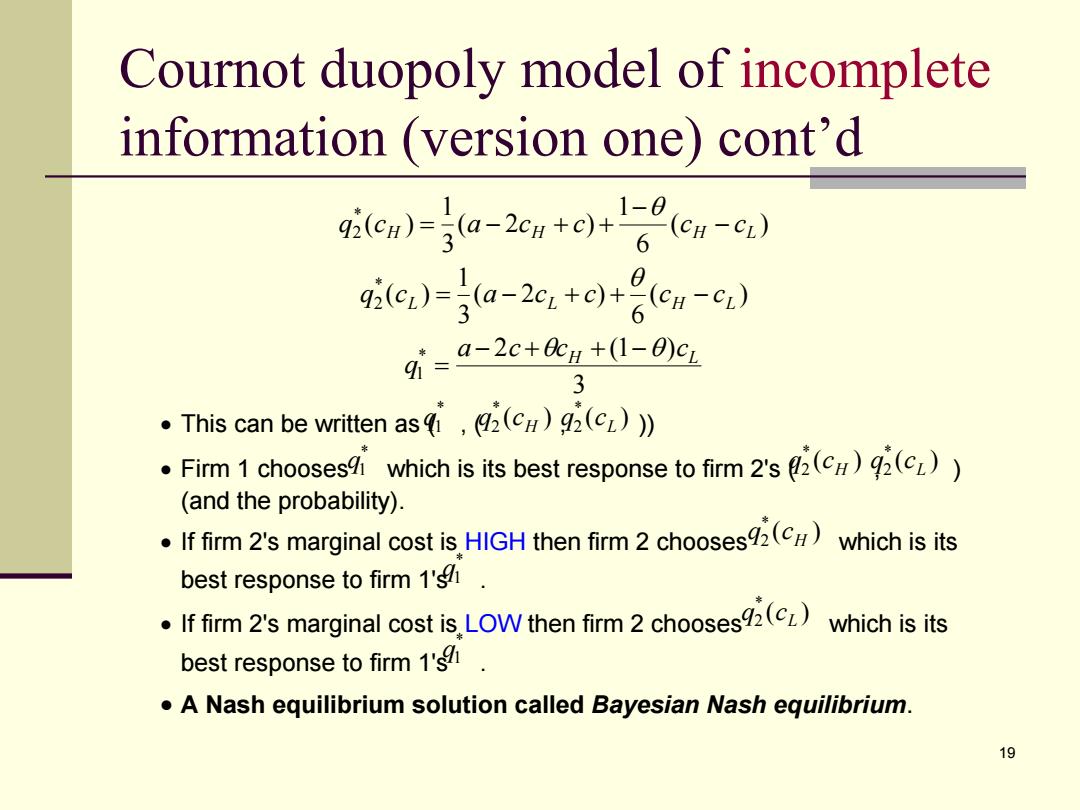
Cournot duopoly model of incomplete information (version one)cont'd qieu)-5a-29r+e+lgcu-小 G(c)-j( -2e)0(c) 9g=a-2c+m+1-0)c2 3 。This can be written as4,A(cH)9(cz)》 Firm 1 chooses which is its best response to firm 2's (c)92(cL)) (and the probability). .If firm 2's marginal cost is HIGH then firm 2 choosesC)which is its best response to firm 1'sh .If firm 2's marginal cost is LOW then firm 2 chooses)which is its best response to firm 1'sh A Nash equilibrium solution called Bayesian Nash equilibrium. 19
19 Cournot duopoly model of incomplete information (version one) cont’d ( ) 6 1 ( 2 ) 3 1 ( ) * 2 H H H L q c a c c c c ( ) 6 ( 2 ) 3 1 ( ) * 2 L L H L q c a c c c c 3 * 2 (1 ) 1 H L a c c c q This can be written as ( * q1 , ( ( ) * 2 H q c , ( ) * 2 L q c )) Firm 1 chooses * q1 which is its best response to firm 2's ( ( ) * 2 H q c , ( ) * 2 L q c ) (and the probability). If firm 2's marginal cost is HIGH then firm 2 chooses ( ) * 2 H q c which is its best response to firm 1's * q1 . If firm 2's marginal cost is LOW then firm 2 chooses ( ) * 2 L q c which is its best response to firm 1's * q1 . A Nash equilibrium solution called Bayesian Nash equilibrium
Cournot duopoly model of incomplete information (version two) A homogeneous product is produced by only two firms:firm 1 and firm 2.The quantities are denoted by g and 42,respectively. ■ They choose their quantities simultaneously. ■ The market price:P(Q)=a-2,where a is a constant number and O=q1+92. All the above are common knowledge 20
20 Cournot duopoly model of incomplete information (version two) A homogeneous product is produced by only two firms: firm 1 and firm 2. The quantities are denoted by q1 and q2 , respectively. They choose their quantities simultaneously. The market price: P(Q)=a-Q, where a is a constant number and Q=q1+q2 . All the above are common knowledge