
Cournot duopoly model of incomplete information A homogeneous product is produced by only two firms:firm 1 and firm 2.The quantities are denoted by q,and 42,respectively. They choose their quantities simultaneously. ■ The market price:P(O)=a-0,where a is a constant number and =q1+92. ■ Firm 1's cost function:Ci(q)=cq1. All the above are common knowledge 11
11 Cournot duopoly model of incomplete information A homogeneous product is produced by only two firms: firm 1 and firm 2. The quantities are denoted by q1 and q2 , respectively. They choose their quantities simultaneously. The market price: P(Q)=a-Q, where a is a constant number and Q=q1+q2 . Firm 1’s cost function: C1 (q1 )=cq1 . All the above are common knowledge

Cournot duopoly model of incomplete information cont'd Firm 2's marginal cost depends on some factor(e.g.technology) that only firm 2 knows.Its marginal cost can be HIGH:cost function:C2(g2)=CH42. LOW:cost function:C2(42)=cL42. ■ Before production,firm 2 can observe the factor and know exactly which level of marginal cost is in. ■ However,firm 1 cannot know exactly firm 2's cost.Equivalently. it is uncertain about firm 2's payoff. Firm 1 believes that firm 2's cost function is > C2(g2)=cH42 with probability 0,and > C2(q2)=c1q2 with probability 1-0. ■ All the above are common knowledge ■ The Harsanyi Transformation Independent types 12
12 Cournot duopoly model of incomplete information cont’d Firm 2’s marginal cost depends on some factor (e.g. technology) that only firm 2 knows. Its marginal cost can be HIGH: cost function: C2 (q2 )=cHq2 . LOW: cost function: C2 (q2 )=cLq2 . Before production, firm 2 can observe the factor and know exactly which level of marginal cost is in. However, firm 1 cannot know exactly firm 2’s cost. Equivalently, it is uncertain about firm 2’s payoff. Firm 1 believes that firm 2’s cost function is C2 (q2 )=cHq2 with probability , and C2 (q2 )=cLq2 with probability 1–. All the above are common knowledge The Harsanyi Transformation Independent types

Cournot duopoly model of incomplete information cont'd A solution for the Cournot duopoly model of incomplete information Firm 2 knows exactly its marginal cost is high or low. If its marginal cost is high,i.e.C2(2)=cHg2,then,for any given g,it will solve Max q92[a-(91+92)-cH] s.t. 92≥0 ·F0c:a-9-29-cH=0→9(cH)=)a-9h-cH) g2(CH)is firm 2's best response to g1,if its marginal cost is high. 13
13 Cournot duopoly model of incomplete information cont’d A solution for the Cournot duopoly model of incomplete information Firm 2 knows exactly its marginal cost is high or low. If its marginal cost is high, i.e. 2 2 2 C (q ) cH q , then, for any given 1 q , it will solve . . 0 [ ( ) ] 2 2 1 2 st q Max q a q q cH FOC: ( ) 2 1 2 0 ( ) 1 2 H 2 H 1 H a q q c q c a q c ( ) 2 H q c is firm 2's best response to 1 q , if its marginal cost is high
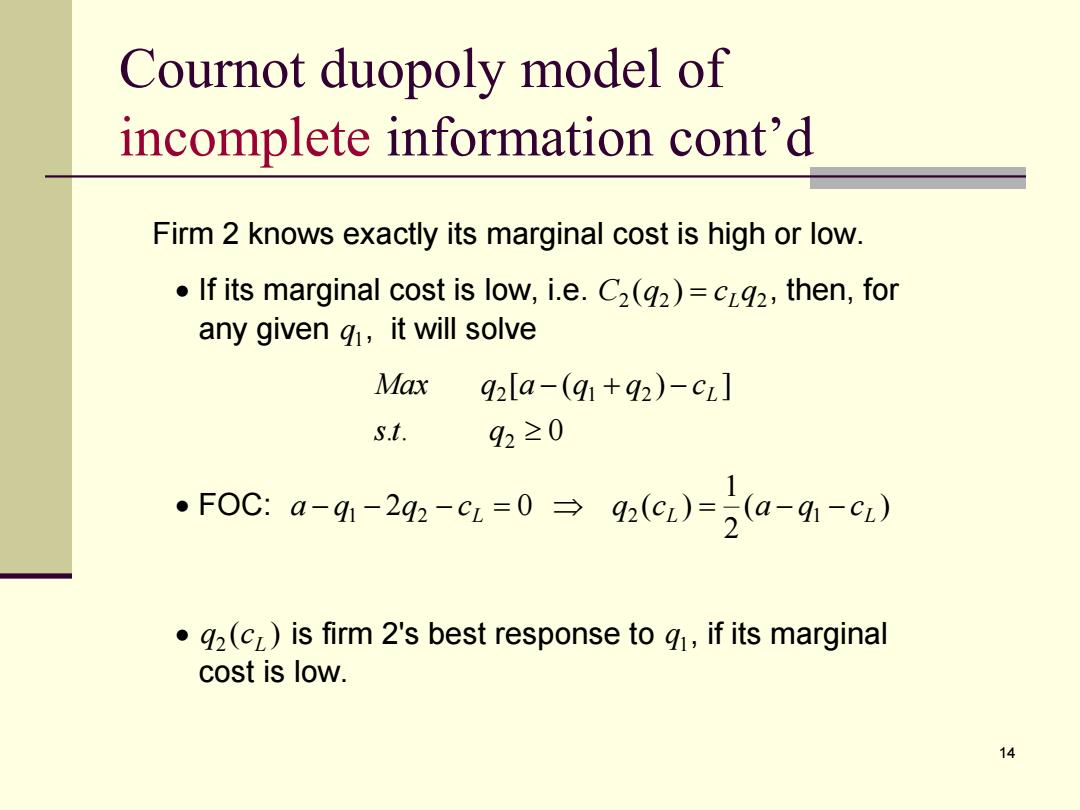
Cournot duopoly model of incomplete information cont'd Firm 2 knows exactly its marginal cost is high or low. If its marginal cost is low,i.e.C2(g2)=cLg2,then,for any given g,it will solve Max 92[a-(91+92)-cz] s.t. 92≥0 F0ca-9h-24:-6=0→9(c)a-9-c) .g2(cL)is firm 2's best response to g,if its marginal cost is low. 14
14 Cournot duopoly model of incomplete information cont’d Firm 2 knows exactly its marginal cost is high or low. If its marginal cost is low, i.e. 2 2 2 C (q ) cLq , then, for any given 1 q , it will solve . . 0 [ ( ) ] 2 2 1 2 st q Max q a q q cL FOC: ( ) 2 1 2 0 ( ) 1 2 L 2 L 1 L a q q c q c a q c ( ) 2 L q c is firm 2's best response to 1 q , if its marginal cost is low

Cournot duopoly model of incomplete information cont'd Firm 1 knows exactly its cost function C()=cq. Firm 1 does not know exactly firm 2's marginal cost is high or low. But it believes that firm 2's cost function is C2(2)=C2 with probability 0,and C2(g2)=cL2 with probability 1-0 Equivalently,it knows that the probability that firm 2's quantity is g2(c)is 0,and the probability that firm 2's quantity is g2(cL)is 1-0.So it solves Max 0xq[a-(g+92(CH))-c] +(1-8)×91[a-(91+92(cz)-c] S.t. 91≥0 15
15 Cournot duopoly model of incomplete information cont’d Firm 1 knows exactly its cost function 1 1 1 C (q ) cq . Firm 1 does not know exactly firm 2's marginal cost is high or low. But it believes that firm 2's cost function is 2 2 2 C (q ) cH q with probability , and 2 2 2 C (q ) cLq with probability 1 Equivalently, it knows that the probability that firm 2's quantity is ( ) 2 H q c is , and the probability that firm 2's quantity is ( ) 2 L q c is 1 . So it solves . . 0 (1 ) [ ( ( )) ] [ ( ( )) ] 1 1 1 2 1 1 2 st q q a q q c c Max q a q q c c L H