
CHAPTER 14 COMPLEX STRAIN AND THE ELASTIC CONSTANTS Summary The relationships between the elastic constants are E=2G(1+v)and E=3K(1-2v) Poisson's ratio v being defined as the ratio of lateral strain to longitudinal strain and bulk modulus K as the ratio of volumetric stress to volumetric strain. The strain in the x direction in a material subjected to three mutually perpendicular stresses in the x,y and z directions is given by -2=Ea-o,-o,) Similar equations apply for E,and e. Thus the principal strain in a given direction can be found in terms of the principal stresses, since 6=是-管2-,-0-o For a two-dimensional stress system (i.e.a3 =0),principal stresses can be found from known principal strains,since -(e+veE and 02 eveE 01 (1-2) (1-2) When the linear strains in two perpendicular directions are known,together with the associated shear strain,or when three linear strains are known,the principal strains are easily determined by the use of Mohr's strain circle. 14.1.Linear strain for tri-axial stress state Consider an element subjected to three mutually perpendicular tensile stresses ao,and o. as shown in Fig.14.1. If o,ando were not present the strain in the x direction would,from the basic definition of Young's modulus E,be Ex= E 361
CHAPTER 14 COMPLEX STRAIN AND THE ELASTIC CONSTANTS Summary The relationships between the elastic constants are E = 2G(1 +v) and E = 3K(1-2v) Poisson's ratio v being defined as the ratio of lateral strain to longitudinal strain and bulk modulus K as the ratio of volumetric stress to volumetric strain. The strain in the x direction in a material subjected to three mutually perpendicular stresses in the x, y and z directions is given by Qx 0 0, 1 & =--v-L,-v-=-((a -VQ -VQ) "E E EE" " Similar equations apply for E, and E,. since Thus the principal strain in a given direction can be found in terms of the principal stresses, For a two-dimensional stress system (i.e. u3 = 0), principal stresses can be found from known principal strains, since E (-52 + VEl) (1 - v2) E and o2 = (81 + YE21 (1 - v2) 61 = When the linear strains in two perpendicular directions are known, together with the associated shear strain, or when three linear strains are known, the principal strains are easily determined by the use of Mohr's strain circle. 14.1. Linear strain for tri-axial stress state Consider an element subjected to three mutually perpendicular tensile stresses Q,, Q, and Q, If Q, and Q, were not present the strain in the x direction would, from the basic definition of as shown in Fig. 14.1. Young's modulus E, be QX E, = - E 36 1
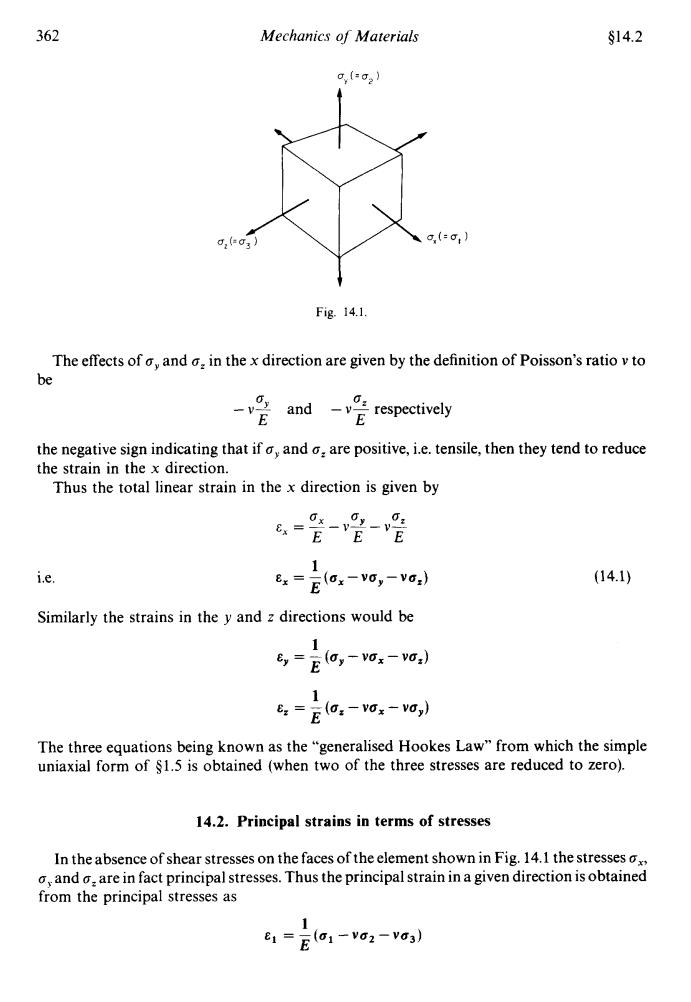
362 Mechanics of Materials S14.2 c(=g2) g,(=g,) Fig.14.1. The effects of and in the x direction are given by the definition of Poisson's ratio v to be and respectively E the negative sign indicating that if a,and o:are positive,i.e.tensile,then they tend to reduce the strain in the x direction. Thus the total linear strain in the x direction is given by 6-2--呢 1 i.e. 6x=E(ox-vo,-vo;) (14.1) Similarly the strains in the y and z directions would be 1 ,=Eo,-vox-vo,) 1 e:=E(:-vo,-vo,) The three equations being known as the "generalised Hookes Law"from which the simple uniaxial form of $1.5 is obtained (when two of the three stresses are reduced to zero). 14.2.Principal strains in terms of stresses In the absence of shear stresses on the faces of the element shown in Fig.14.1 the stresses ox o and o.are in fact principal stresses.Thus the principal strain in a given direction is obtained from the principal stresses as 1 6 =E(01-vo2-va3)
362 Mechankr oj Materials g14.2 t Fig. 14.1 The effects of Q, and cZ in the x direction are given by the definition of Poisson’s ratio v to be Q 0 - v2 and - vP respectively E E the negative sign indicating that if Q, and Q, are positive, i.e. tensile, then they tend to reduce the strain in the x direction. Thus the total linear strain in the x direction is given by E, = -((6,-VfJy-V~,) 1 E Le. (14.1) Similarly the strains in the y and z directions would be 1 E E, = - (Qy - vox - va,) E, = -(a, 1 - vox - YOy) E The three equations being known as the “generalised Hookes Law” from which the simple uniaxial form of $1.5 is obtained (when two of the three stresses are reduced to zero). 14.2. Principal strains in terms of stresses In the absence of shear stresses on the faces of the element shown in Fig. 14.1 the stresses ox, CJ) and Q, are in fact principal stresses. Thus the principal strain in a given direction is obtained from the principal stresses as 1 E E1 = - (ul - vu2 - vu3)

§14.3 Complex Strain and the Elastic Constants 363 1 or 82=Eo2-vo1-va3) (14.2) 1 or 63 =E(03-vo1-va2) 14.3.Principal stresses in terms of strains-two-dimensional stress system For a two-dimensional stress system,i.e.o3 =0,the above equations reduce to 1 81=Eo1-o2) 1 and 82=E(o2-vo) 1 with g=E(-o1-G2) E81=01-o2 E82=02-vo1 Solving these equations simultaneously yields the following values for the principal stresses: E 01= (1-v2) (E1+VE2) E (14.3) and 2=1-v吗e2+ve) 14.4.Bulk modulus K It has been shown previously that Young's modulus E and the shear modulus G are defined as the ratio of stress to strain under direct load and shear respectively.Bulk modulus is similarly defined as a ratio of stress to strain under uniform pressure conditions.Thus if a material is subjected to a uniform pressure (or volumetric stress)a in all directions then volumetric stress bulk modulus volumetric strain 0 i.e. K= (14.4) Ev the volumetric strain being defined below. 14.5.Volumetric strain Consider a rectangular block of sides x,y and z subjected to a system of equal direct stresses a on each face.Let the sides be changed in length by &x,oy and oz respectively under stress (Fig.14.2)
514.3 or or Complex Strain and the Elastic Constants 1 E - -(az-val -V63) z- E E3 = - (63 - vu1 - vaz) 1 E 363 (14.2) 14.3. Principal stresses in terms of strains - two-dimensional stress system For a two-dimensional stress system, Le. a3 = 0, the above equations reduce to 1 E 1 E 1 E E1 = - (crl - va2) E2 = - (az - Vbl) E3 = - (- Val- YO2) and with .. = a1 - va2 EEZ = 02 - VO~ Solving these equations simultaneously yields the following values for the principal stresses: and (14.3) 14.4. Bulk modulus K It has been shown previously that Young’s modulus E and the shear modulus G are defined as the ratio of stress to strain under direct load and shear respectively. Bulk modulus is similarly defined as a ratio of stress to strain under uniform pressure conditions. Thus if a material is subjected to a uniform pressure (or volumetric stress) 0 in all directions then volumetric stress volumetric strain bulk modulus = a i.e. K=- E, the volumetric strain being defined below. 14.5. Volumetric strain ( 14.4) Consider a rectangular block of sides x, y and z subjected to a system of equal direct stresses a on each face. Let the sides be changed in length by Sx, 6y and 6z respectively under stress (Fig. 14.2)
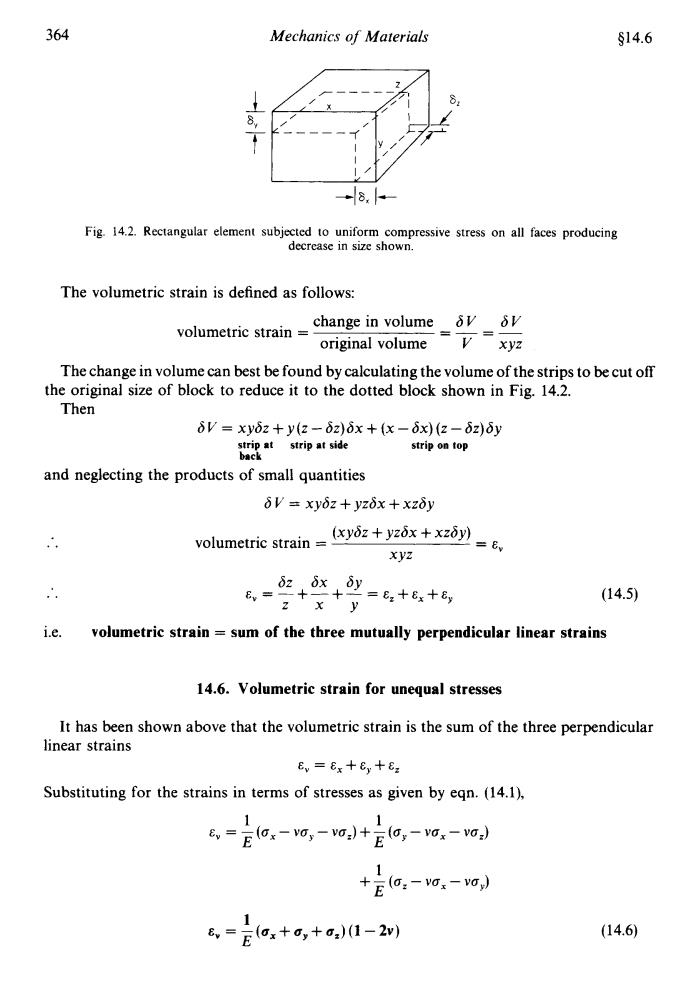
364 Mechanics of Materials §14.6 8, 8, Fig.14.2.Rectangular element subjected to uniform compressive stress on all faces producing decrease in size shown. The volumetric strain is defined as follows: volumetric strain change in volume 6v 1 original volume -Vxy: The change in volume can best be found by calculating the volume of the strips to be cut off the original size of block to reduce it to the dotted block shown in Fig.14.2. Then 6V=xyδz+y(z-6z)6x+(x-6x)(z-6z)δy strip at strip at side strip on top back and neglecting the products of small quantities δV=xyδz+yzδx+xzòy volumetric strainyxxy) xyz 828x8y=+ex+6 6= (14.5) z x y i.e. volumetric strain sum of the three mutually perpendicular linear strains 14.6.Volumetric strain for unequal stresses It has been shown above that the volumetric strain is the sum of the three perpendicular linear strains Ev=Ex+Ey+E Substituting for the strains in terms of stresses as given by eqn.(14.1), 1 1 6=E(ax-vo,-vo;)+E(o,-va,-vo,) 1 FE(a:-va:-va,) 1 8,= Eox+o,+)(1-2) (14.6)
364 Mechanics of Materials 914.6 Fig. 14.2. Rectangular element subjected to uniform compressive stress on all faces producing decrease in size shown. The volumetric strain is defined as follows: change in volume 6V SV volumetric strain = =- =- original volume V xyz The change in volume can best be found by calculating the volume of the strips to be cut off Then the original size of block to reduce it to the dotted block shown in Fig. 14.2. sv = xysz + y(z - 6Z)hX + (x - 6x) (z - 6z)sy strip at strip at side strip on top back and neglecting the products of small quantities .. 6 v = xysz + yzdx + xzsy volumetric strain = = E, (xydz + yzsx + xzhy) XYZ .. (14.5) i.e. volumetric strain = sum of tbe three mutually perpendicular linear strains 14.6. Volumetric strain for unequal stresses It has been shown above that the volumetric strain is the sum of the three perpendicular linear strains E, = E, + Eg + E, Substituting for the strains in terms of stresses as given by eqn. (14.1), 1 1 E E 1 E E, = - (a, - VbY - va,) +-(ay - va, - vaJ + - (a, - Vb, - vay) 1 EV = ~(u*+U,+u,)(l-2") (14.6)
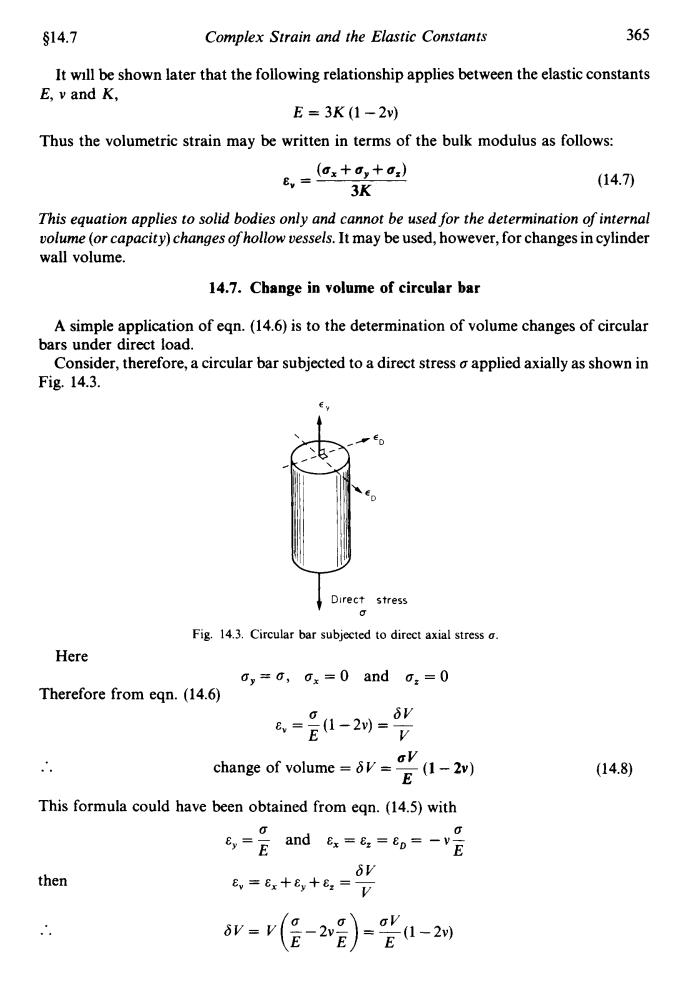
§14.7 Complex Strain and the Elastic Constants 365 It will be shown later that the following relationship applies between the elastic constants E,v and K, E=3K(1-2y) Thus the volumetric strain may be written in terms of the bulk modulus as follows: 6,t* (14.7) 3K This equation applies to solid bodies only and cannot be used for the determination of internal volume (or capacity)changes ofhollow vessels.It may be used,however,for changes in cylinder wall volume. 14.7.Change in volume of circular bar A simple application of egn.(14.6)is to the determination of volume changes of circular bars under direct load. Consider,therefore,a circular bar subjected to a direct stress a applied axially as shown in Fig.14.3. Direct stress Fig.14.3.Circular bar subjected to direct axial stress o. Here 0y=0,0x=0andg,=0 Therefore from eqn.(14.6) 8V 8.=E1-2)= change of volume=(1-2v) (14.8) This formula could have been obtained from eqn.(14.5)with e=E and 6s=6=6o=-VE oV then e,=6x+8,+8:= 6v=v(但-2)=Y4-2刘
$14.7 Complex Strain and the Elastic Constants 365 It will be shown later that the following relationship applies between the elastic constants E, v and K, Thus the volumetric strain may be written in terms of the bulk modulus as follows: E=3K(1-2~) (14.7) This equation applies to solid bodies only and cannot be used for the determination of internal volume (or capacity) changes of hollow vessels. It may be used, however, for changes in cylinder wall volume. 14.7. Change in volume of circular bar A simple application of eqn. (14.6) is to the determination of volume changes of circular Consider, therefore, a circular bar subjected to a direct stress 6 applied axially as shown in bars under direct load. Fig. 14.3. EY * % c Direct stress U Fig. 14.3. Circular bar subjected to direct axial stress u. cy= 6, cx = 0 and 0, = 0 Here Therefore from eqn. (14.6) 6V E, = -(1-2v) = - E V 6 UV E .. This formula could have been obtained from eqn. (14.5) with change of volume = 6 V = - (1 - 2v) 6 d E, = - and E, = E, = E,, = - v- E E 6V V then E,=E,+E,+E,=- (14.8)