CHAPTER 9 THIN CYLINDERS AND SHELLS Summary The stresses set up in the walls of a thin cylinder owing to an internal pressure p are: circumferential or hoop stress o#= pd 2t pd longitudinal or axial stress 4t where d is the internal diameter and t is the wall thickness of the cylinder. Then: longitudinal strain ELGL-van] hoop strain & ELon-vaL] change of interal volume of cylinder under pressurep 4E[5-4p]r change of volume of contained liquid under pressure- pV K where K is the bulk modulus of the liquid. For thin rotating cylinders of mean radius R the tensile hoop stress set up when rotating at ωrad/s is given by H=p02R2. For thin spheres: cirumferentialor hoop stressd 4t change of volume under pressure 3pd 4E1-y Effects of end plates and joints-add "joint efficiency factor"n to denominator of stress equations above. 9.1.Thin cylinders under internal pressure When a thin-walled cylinder is subjected to internal pressure,three mutually perpendicular principal stresses will be set up in the cylinder material,namely the circumferential or hoop 198
CHAPTER 9 THIN CYLINDERS AND SHELLS Summary The stresses set up in the walls of a thin cylinder owing to an internal pressure p are: circumferential or hmp stress aH = Pd Pd longitudinal or axial stress aL = - 4t where d is the internal diameter and t is the wall thickness of the cylinder. longitudinal strain cL = - [aL - VaH] 1 E 1 E Then: hoop strain cH = - [aH - vaL] Fd 4tE PV change of volume of contained liquid under pressure = - K change of internal volume of cylinder under pressure = - [ 5 - 4v] V where K is the bulk modulus of the liquid. For thin rotating cylinders of mean radius R the tensile hoop stress set up when rotating at For thin spheres: w rad/s is given by GH = po2R2. Pd circumferential or hoop stress aH = - 4t 3Pd change of volume under pressure = - [ 1 - v] V 4tE Eflects of end plates and joints-add “joint efficiency factor” ‘1 to denominator of stress equations above. 9.1. Thin cylinders under internal pressure When a thin-walled cylinder is subjected to internal pressure, three mutually perpendicular principal stresses will be set up in the cylinder material, namely the circumferential or hoop 198
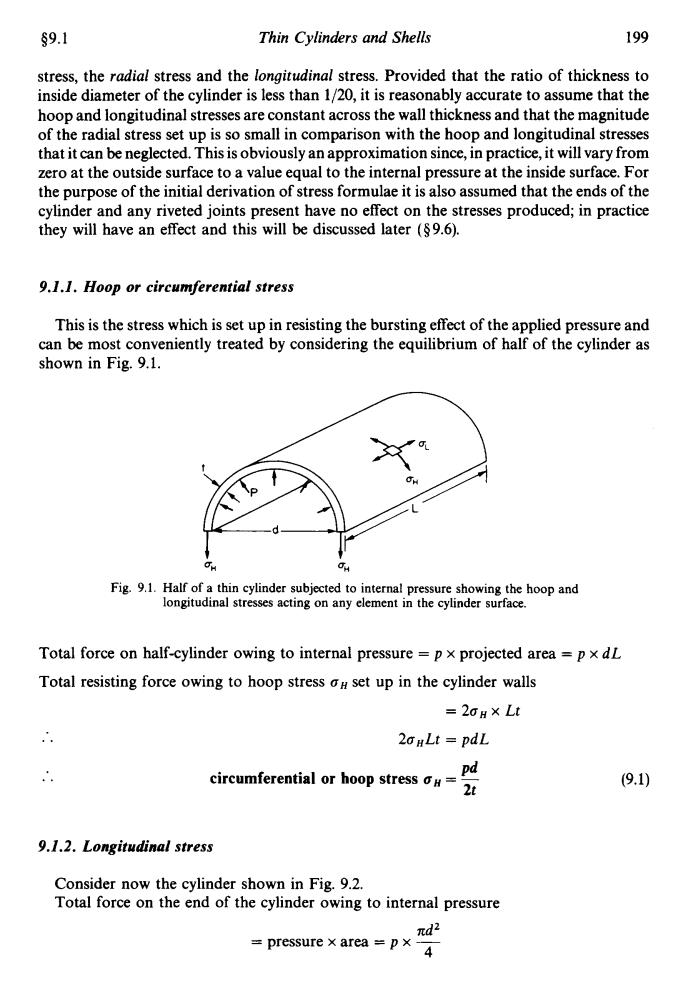
s9.1 Thin Cylinders and Shells 199 stress,the radial stress and the longitudinal stress.Provided that the ratio of thickness to inside diameter of the cylinder is less than 1/20,it is reasonably accurate to assume that the hoop and longitudinal stresses are constant across the wall thickness and that the magnitude of the radial stress set up is so small in comparison with the hoop and longitudinal stresses that it can be neglected.This is obviously an approximation since,in practice,it will vary from zero at the outside surface to a value equal to the internal pressure at the inside surface.For the purpose of the initial derivation of stress formulae it is also assumed that the ends of the cylinder and any riveted joints present have no effect on the stresses produced;in practice they will have an effect and this will be discussed later ($9.6). 9.1.1.Hoop or circumferential stress This is the stress which is set up in resisting the bursting effect of the applied pressure and can be most conveniently treated by considering the equilibrium of half of the cylinder as shown in Fig.9.1. CH 60 Fig.9.1.Half of a thin cylinder subjected to internal pressure showing the hoop and longitudinal stresses acting on any element in the cylinder surface. Total force on half-cylinder owing to internal pressure p x projected area =p x dL Total resisting force owing to hoop stress aH set up in the cylinder walls =2oH×Lt 20HLt pdL circumferential or hoop stress o#= pd 2t (9.1) 9.1.2.Longitudinal stress Consider now the cylinder shown in Fig.9.2. Total force on the end of the cylinder owing to internal pressure nd2 pressure x area=px4
59.1 Thin Cylinders and Shells 199 stress, the radial stress and the longitudinal stress. Provided that the ratio of thickness to inside diameter of the cylinder is less than 1/20, it is reasonably accurate to assume that the hoop and longitudinal stresses are constant across the wall thickness and that the magnitude of the radial stress set up is so small in comparison with the hoop and longitudinal stresses that it can be neglected. This is obviously an approximation since, in practice, it will vary from zero at the outside surface to a value equal to the internal pressure at the inside surface. For the purpose of the initial derivation of stress formulae it is also assumed that the ends of the cylinder and any riveted joints present have no effect on the stresses produced; in practice they will have an effect and this will be discussed later (5 9.6). 9.1.1. Hoop or circumferential stress This is the stress which is set up in resisting the bursting effect of the applied pressure and can be most conveniently treated by considering the equilibrium of half of the cylinder as shown in Fig. 9.1. QU Qn Fig. 9.1. Half of a thin cylinder subjected to internal pressure showing the hoop and longitudinal stresses acting on any element in the cylinder surface. Total force on half-cylinder owing to internal pressure = p x projected area = p x dL Total resisting force owing to hoop stress on set up in the cylinder walls = 2oH x Lt .. 2aHLt = pdL Pd .. circumferential or hoop stress uH = - 2t 9.1.2. Longitudinal stress Consider now the cylinder shown in Fig. 9.2. Total force on the end of the cylinder owing to internal pressure nd2 = pressure x area = p x ~ 4
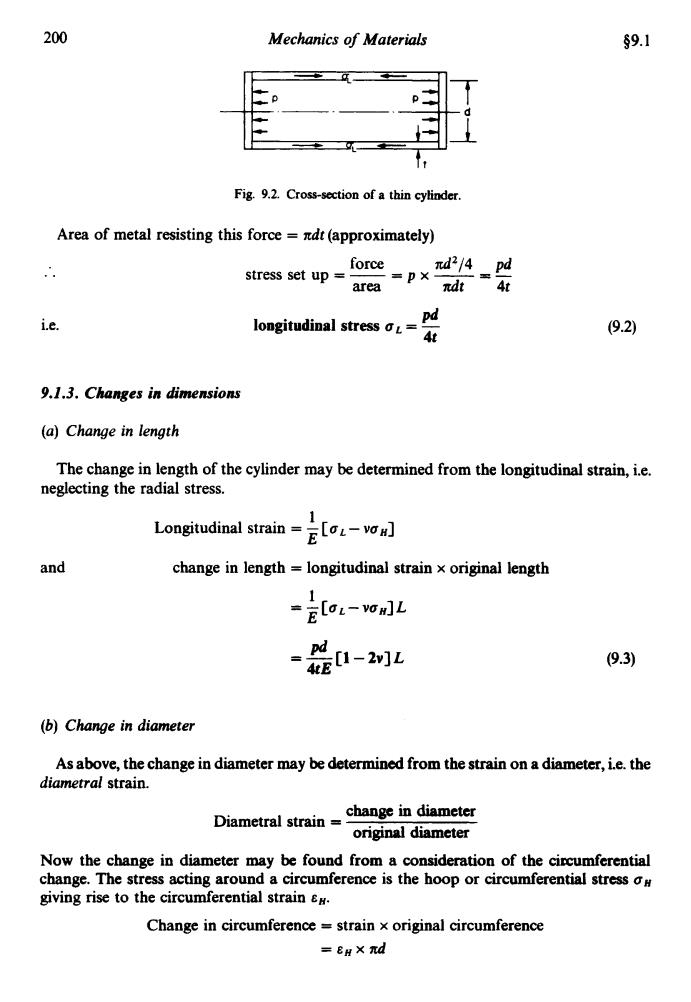
200 Mechanics of Materials $9.1 Fig.9.2.Cross-section of a thin cylinder. Area of metal resisting this force ndt(approximately) force nd2/4 pd stress set up =卫X area 元dt4t i.e. Pd longitudinal stress o= (9.2) 4t 9.1.3.Changes in dimensions (a)Change in length The change in length of the cylinder may be determined from the longitudinal strain,i.e. neglecting the radial stress. Longitudinal strain-E[-vo and change in length longitudinal strain x original length 1 -EloL-vOm]L -是1-2 (9.3) (b)Change in diameter As above,the change in diameter may be determined from the strain on a diameter,i.e.the diametral strain. Diametral strain change in diameter original diameter Now the change in diameter may be found from a consideration of the circumferential change.The stress acting around a circumference is the hoop or circumferential stress o# giving rise to the circumferential strain Change in circumference strain x original circumference =eH×元d
200 Mechanics of Materials 09.1 Fig. 9.2. Cross-section of a thin cylinder. Area of metal resisting this force = ltdt(approximate1y) .. i.e. force d2/4 pd stress set up = - = p x - = - area ndt 4t pd longitudinal stress uL = - 4t 9.1.3. Changes in dinrensions (a) Change in length The change in length of the cylinder may be determined from the longitudinal strain, i.e. neglecting the radial stress. 1 E Longitudinal strain = - [uL - vuH] and change in length = longitudinal strain x original length 1 E = -[uL-vuH]L pd =-[1-2v]L 4tE (b) Change in diameter (9.3) As above, the change in diameter may be determined from the strain on a diameter, i.e. the diametral strain. change in diameter original diameter Diametral strain = Now the change in diameter may be found from a consideration of the cipcumferential change. The stress acting around a circumference is the hoop or circumferential stress on giving rise to the circumferential strain cH. Change in circumference = strain x original circumference =EHXnd

s9.2 Thin Cylinders and Shells 201 New circumference nd+nde# =d(1+eH) But this is the circumference of a circle of diameter d(1+8#) New diameter =d(1+) Change in diameter dem d起班一H Diametral strainod i.e. the diametral strain equals the hoop or circumferential strain (94) d。 Thus change in diameterden-[v E2-] (9.5) (c)Change in internal volume Change in volume volumetric strain x original volume From the work of§14.5,page364. volumetric strain sum of three mutually perpendicular direct strains 8L+26D 1 2 =ELoL-vom]+ECon-voL] 1 =E[oL+20-v(on+201)] pd1+4-v2+2] 5-4幻 Therefore with original internal volume V change in internal volumep 4E5-4n]y (9.6) 9.2.Thin rotating ring or cylinder Consider a thin ring or cylinder as shown in Fig.9.3 subjected to a radial pressure p caused by the centrifugal effect of its own mass when rotating.The centrifugal effect on a unit length
$9.2 Thin Cylinders and Sheh 201 New circumference = xd + 7cd~ H = d(1 +EH) But this is the circumference of a circle of diameter d (1 +E,,) .. .. New diameter = d (1 + E~) Change in diameter = dEH d&H Diametral strain E,, = - = eH the diametral strain equals the hoop or circumferential strain d i.e. (9.4) d E Thus change in diameter = deH = - [aH - voL] Pd’ = ---[2-v] 4tE (c) Change in internal volume Change in volume = volumetric strain x original volume From the work of $14.5, page 364. volumetric strain = sum of three mutually perpendicular direct strains = EL+ 2ED 1 E = -[UL+2aH-v(aH+2aL)J = -[ Pd 1 +4-v(2+2) J 4tE Pd = -[5-4v] 4t E Therefore with original internal volume V Pd cbange in internal volume = - [5 - 4v] Y 4tE 9.2. Thin rotating ring or cylinder (9.5) Consider a thin ring or cylinder as shown in Fig. 9.3 subjected to a radial pressure p caused by the centrifugal effect of its own mass when rotating. The centrifugal effect on a unit length
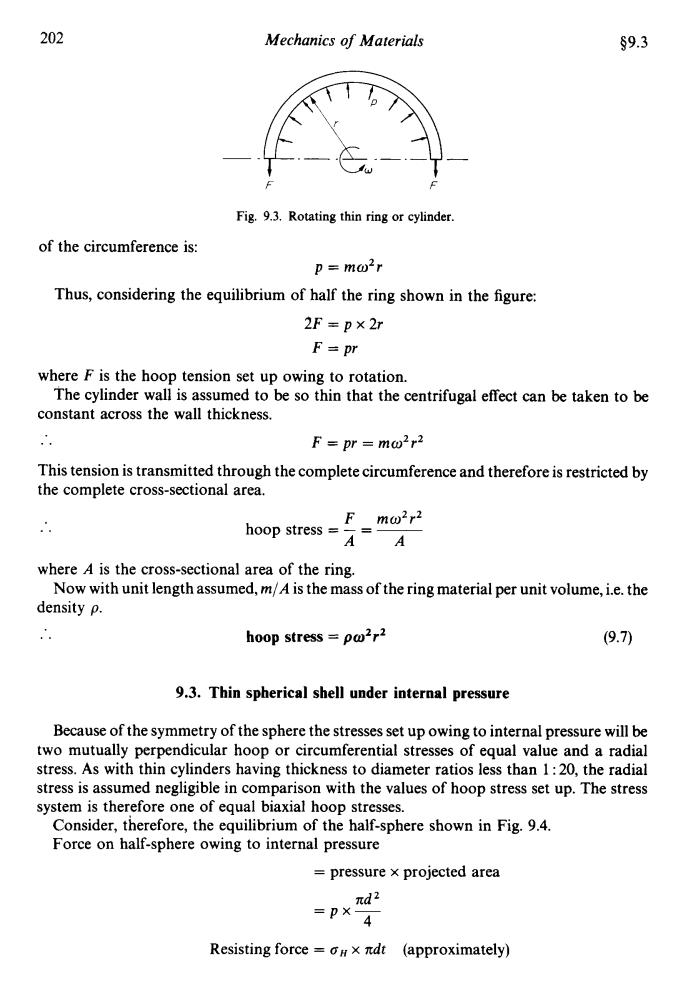
202 Mechanics of Materials §9.3 Fig.9.3.Rotating thin ring or cylinder. of the circumference is: p=mω2r Thus,considering the equilibrium of half the ring shown in the figure: 2F=p×2r F=pr where F is the hoop tension set up owing to rotation. The cylinder wall is assumed to be so thin that the centrifugal effect can be taken to be constant across the wall thickness. F=pr =mo2r2 This tension is transmitted through the complete circumference and therefore is restricted by the complete cross-sectional area. hoop stress F mo2r2 AA where A is the cross-sectional area of the ring. Now with unit length assumed,m/A is the mass of the ring material per unit volume,i.e.the density p. hoop stress =po2r2 (9.7) 9.3.Thin spherical shell under internal pressure Because of the symmetry of the sphere the stresses set up owing to internal pressure will be two mutually perpendicular hoop or circumferential stresses of equal value and a radial stress.As with thin cylinders having thickness to diameter ratios less than 1:20,the radial stress is assumed negligible in comparison with the values of hoop stress set up.The stress system is therefore one of equal biaxial hoop stresses. Consider,therefore,the equilibrium of the half-sphere shown in Fig.9.4. Force on half-sphere owing to internal pressure pressure x projected area nd2 =卫X 4 Resisting force =#x ndt (approximately)
202 Mechanics of Materials $9.3 F F Fig. 9.3. Rotating thin ring or cylinder. of the circumference is: p = mo2r Thus, considering the equilibrium of half the ring shown in the figure: 2F=px2r F = pr where F is the hoop tension set up owing to rotation. constant across the wall thickness. .. This tension is transmitted through the complete circumference and therefore is restricted by the complete cross-sectional area. The cylinder wall is assumed to be so thin that the centrifugal effect can be taken to be F = pr = mo2r2 where A is the cross-sectional area of the ring. density p. Now with unit length assumed, m/A is the mass of the ring material per unit volume, i.e. the .. hoop stress = po2r2 (9.7) 9.3. Thin spherical shell under internal pressure Because of the symmetry of the sphere the stresses set up owing to internal pressure will be two mutually perpendicular hoop or circumferential stresses of equal value and a radial stress. As with thin cylinders having thickness to diameter ratios less than 1 : 20, the radial stress is assumed negligible in comparison with the values of hoop stress set up. The stress system is therefore one of equal biaxial hoop stresses. Consider, therefore, the equilibrium of the half-sphere shown in Fig. 9.4. Force on half-sphere owing to internal pressure = pressure x projected area nd2 =px4 Resisting force = oH x ltdt (approximately)