
Reference Current (Voltage)Direction R B R B E2 E1 A E2 1 >r2 r1 r2 E1-iR-E2-ir2-ir1=0 E1+iR-E2+ir2+ir2=0 E1-E2 E2-E1 i= i r1+r2+R rl+r2+R
Reference Current (Voltage) Direction E 1 iR E 2 ir 2 ir 1 0 E1 B C A i R r1 r2 E2 E1 B C A i R r1 r2 E2 r r R E E i 1 2 1 2 E 1 iR E 2 ir 2 ir 2 0 r r R E E i 1 2 2 1

Reference Current (Voltage)direction i1 A i2 ↑i3 E1-E2+R2i3-Ri1=0 E1 E2 E2-R3i2-R2i3=0 R3 E1-R,i2-Ri1=0 R1 R2 i1+i3=i2 B 日96
Reference Current (Voltage) direction 1 2 0 E E R 2 i 3 R1i1 E1 B A i1 i2 i3 E2 R1 R2 R3 2 0 E R 3 i 2 R 2 i 3 E 1 R 3 i 2 R1i1 0 1 3 2 i i i
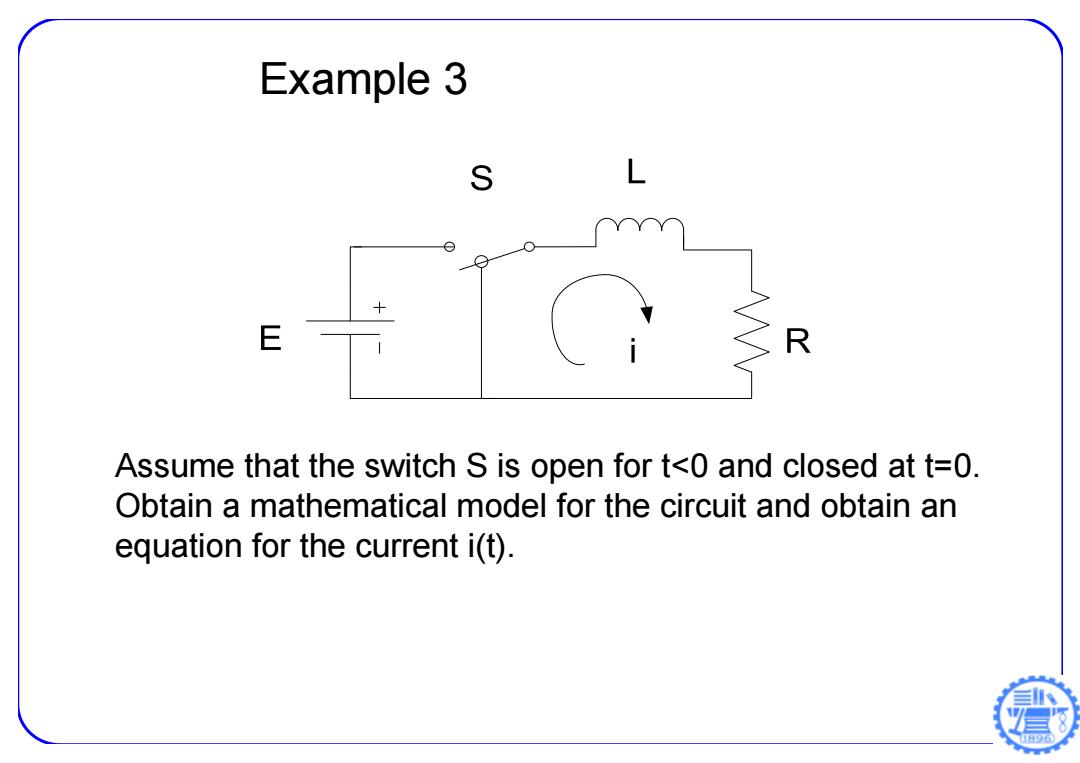
Example 3 S L E R Assume that the switch S is open for t<0 and closed at t=0. Obtain a mathematical model for the circuit and obtain an equation for the current i(t). 6
Example 3 Assume that the switch S is open for t<0 and closed at t=0. Obtain a mathematical model for the circuit and obtain an equation for the current i(t)
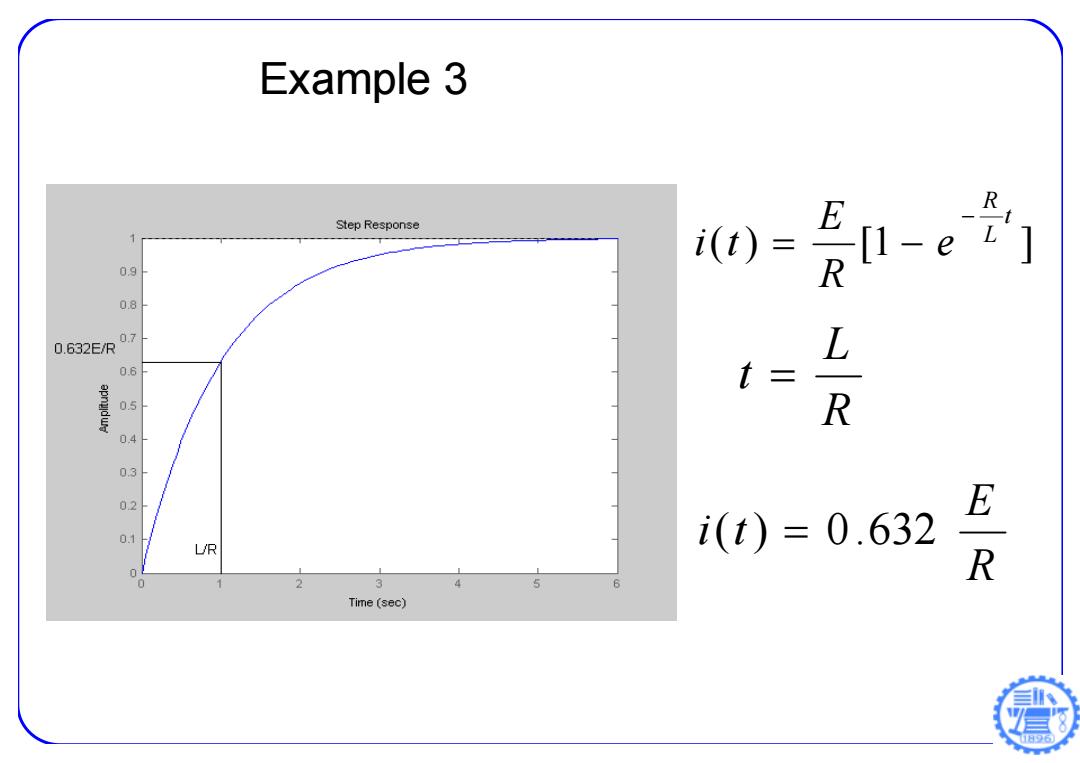
Example 3 E R Step Response i(t) [1-e ] 09 R 08 0.7 0.632E/R L 06 t= 0.5 R 0.4 03 02 E 0.1 i(t)=0.632 UR 0 R 0 2 3 4 5 6 Time(sec)
( ) [1 ] t L R e R E i t R L t R E i(t) 0.632 Example 3

Example 4 S E R Assume that the switch S is open for t<0 and closed at t=0,and is open again at t=t1>0.Obtain a mathematical model for the circuit and obtain an equation for the current i(t). 日6
Example 4 Assume that the switch S is open for t<0 and closed at t=0, and is open again at t=t1>0. Obtain a mathematical model for the circuit and obtain an equation for the current i(t)