
Chapter 3 Transform-Domain Representation of Discrete-Time Signals
Chapter 3 Transform-Domain Representation of Discrete-Time Signals
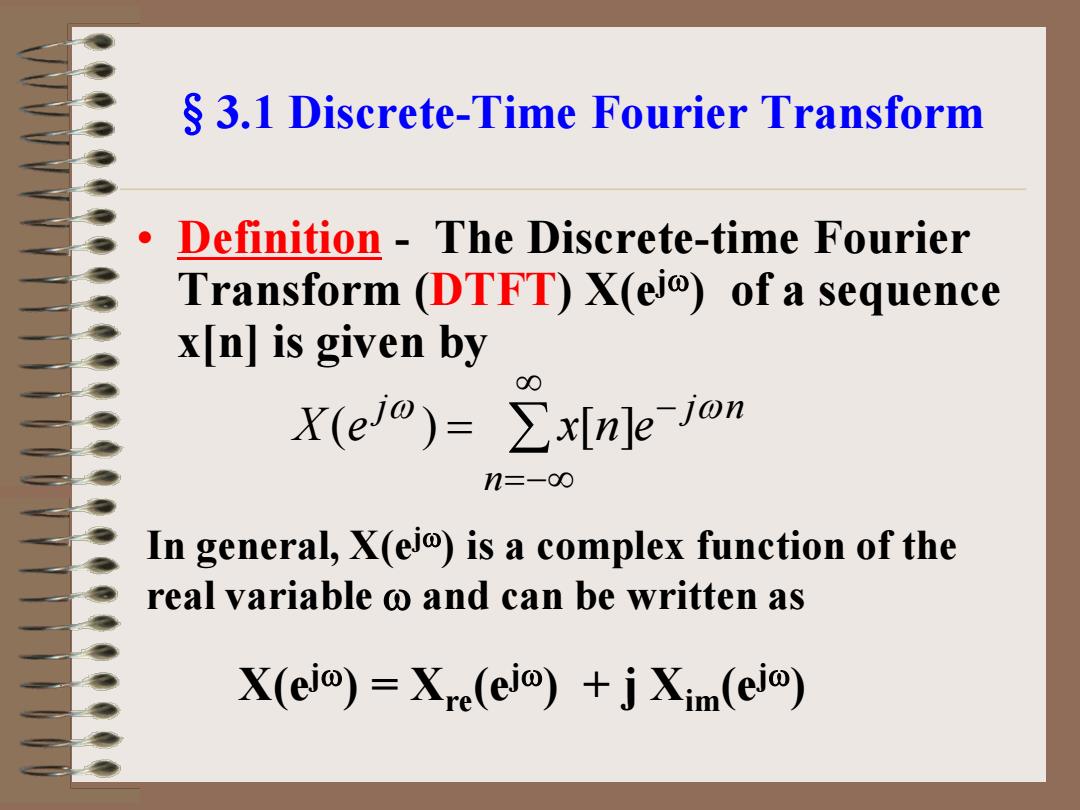
S 3.1 Discrete-Time Fourier Transform Definition The Discrete-time Fourier Transform (DTFT)X(ei)of a sequence x[n]is given by X(eo)=∑x[nle-jon n=-∞ In general,X(ei)is a complex function of the real variable o and can be written as X(ei)=Xre(ei)+jXim(ei)
§3.1 Discrete-Time Fourier Transform • Definition - The Discrete-time Fourier Transform (DTFT) X(ejω) of a sequence x[n] is given by ∑ ∞ =−∞ − = n j j n X e x n e ω ω ( ) [ ] X(ejω) = Xre(ejω) + j Xim(ejω) In general, X(ejω) is a complex function of the real variable ω and can be written as
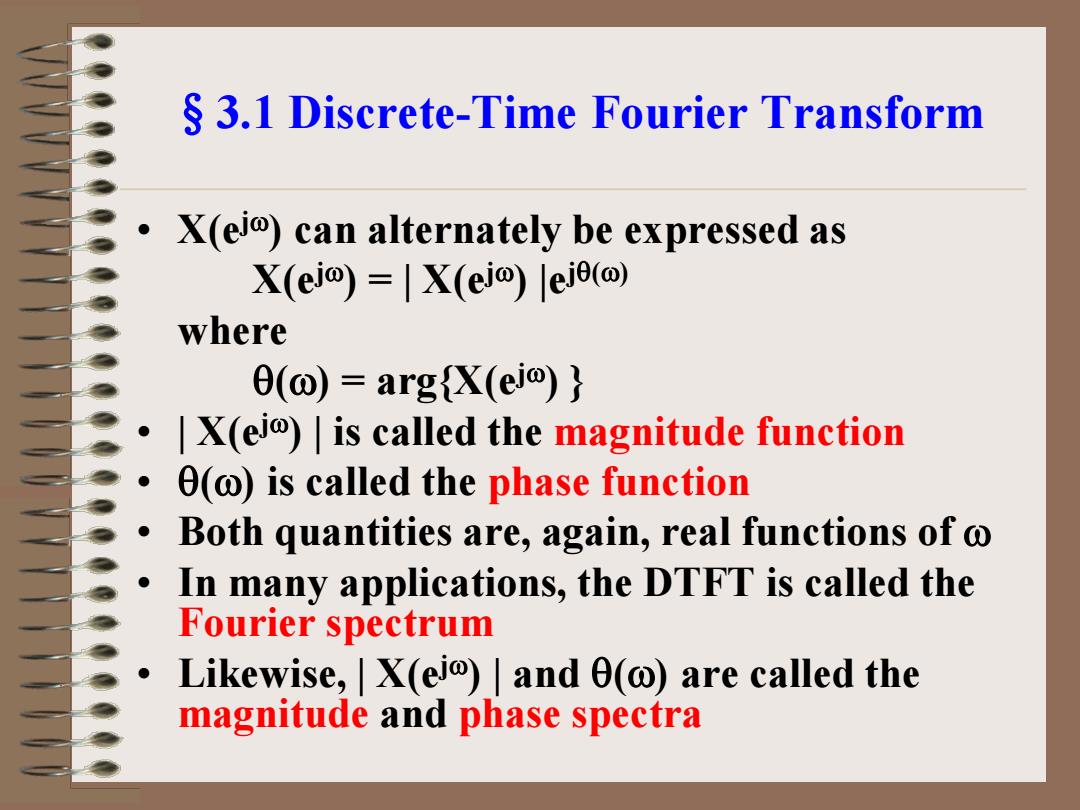
3.1 Discrete-Time Fourier Transform X(ei)can alternately be expressed as X(ei)=X(ei)ei() where ()argx(ei)} X(ei)is called the magnitude function 0()is called the phase function Both quantities are,again,real functions of o In many applications,the DTFT is called the Fourier spectrum Likewise,X(ei)|and 0(@)are called the magnitude and phase spectra
§3.1 Discrete-Time Fourier Transform • X(ejω) can alternately be expressed as X(ejω) = | X(ejω) |ejθ(ω) where θ(ω) = arg{X(ejω) } • | X(ejω) | is called the magnitude function • θ(ω) is called the phase function • Both quantities are, again, real functions of ω • In many applications, the DTFT is called the Fourier spectrum • Likewise, | X(ejω) | and θ(ω) are called the magnitude and phase spectra
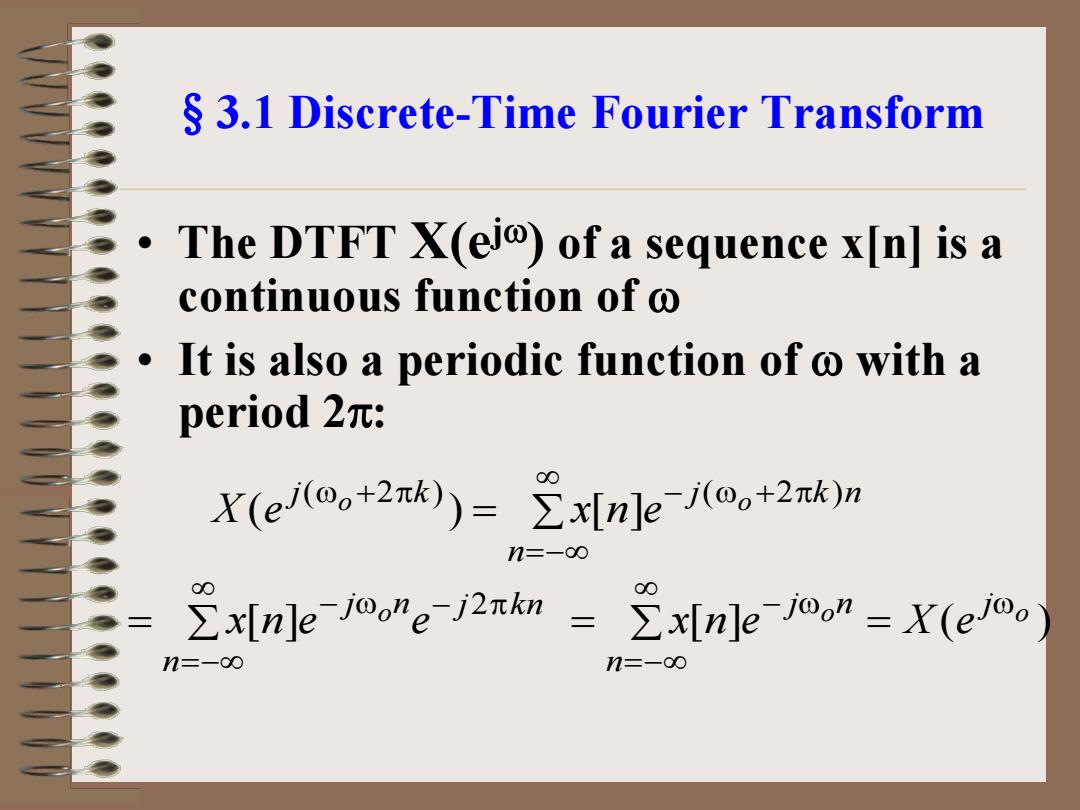
S 3.1 Discrete-Time Fourier Transform The DTFT X(ei)of a sequence x[n]is a continuous function of It is also a periodic function of o with a period2π: X(eo+2xk))=xnle-j(0o+2rk)n 2 x[nJe-j”eJ2πkm=2x[n]]e-Jo"=X(e/oo n=-o
§3.1 Discrete-Time Fourier Transform • The DTFT X(ejω) of a sequence x[n] is a continuous function of ω • It is also a periodic function of ω with a period 2π: = ∑ ∞ =−∞ ω + π − ω + π n j o k j o k n X e x n e ( 2 ) ( 2 ) ( ) [ ] j kn n j n x n e e o − π ∞ =−∞ − ω = ∑ 2 [ ] [ ] ( ) o o j n j n x n e X e ω ∞ =−∞ − ω = ∑ =
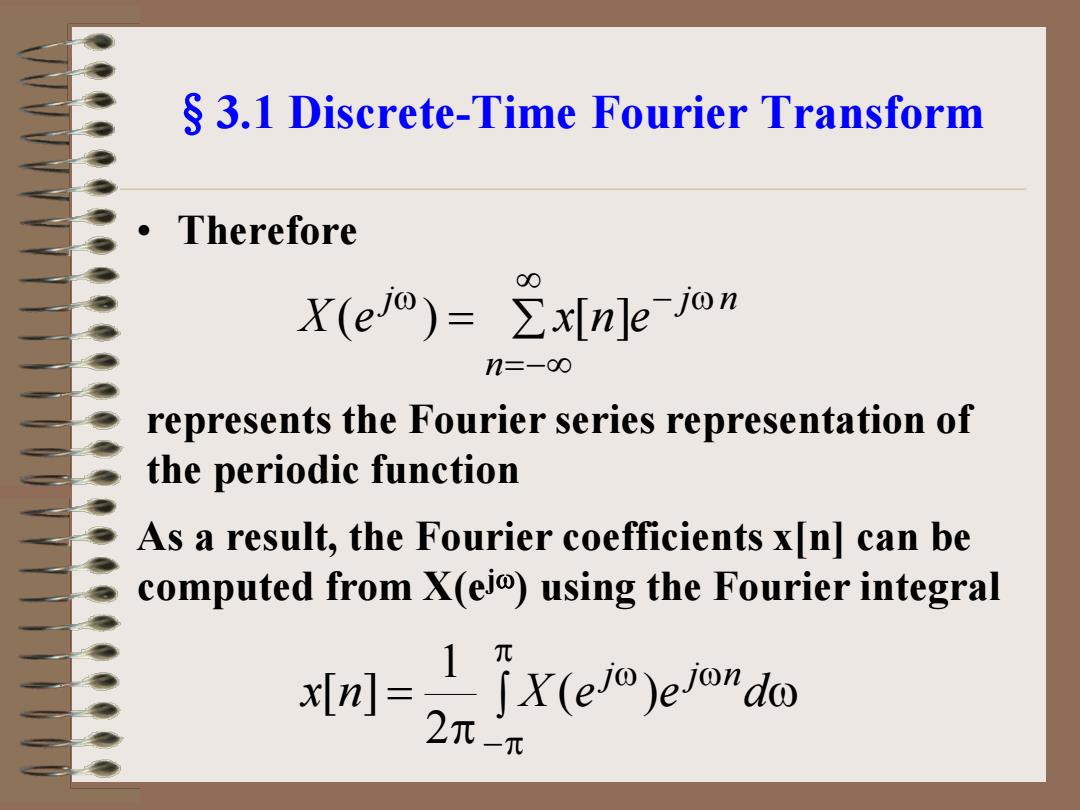
3.1 Discrete-Time Fourier Transform Therefore X(ef)=xInle-jon n=-o0 represents the Fourier series representation of the periodic function As a result,the Fourier coefficients x[n]can be computed from X(ei)using the Fourier integral al2iemema
§3.1 Discrete-Time Fourier Transform • Therefore = ∑ ∞ =−∞ ω − ω n j j n X (e ) x[n]e ∫ ω π = π −π ω ω x n X e e d j j n ( ) 2 1 [ ] As a result, the Fourier coefficients x[n] can be computed from X(ejω) using the Fourier integral represents the Fourier series representation of the periodic function