
Chapter 7 Digital Filter Design
Chapter 7 Digital Filter Design

Objective-Determination of a realizable transfer function G(z)approximating a given frequency response specification is an important step in the development of a digital filter If an IIR filter is desired,G(z)should be a stable real rational function Digital filter design is the process of deriving the transfer function G(z)
• Objective - Determination of a realizable transfer function G(z) approximating a given frequency response specification is an important step in the development of a digital filter • If an IIR filter is desired, G(z) should be a stable real rational function • Digital filter design is the process of deriving the transfer function G(z)
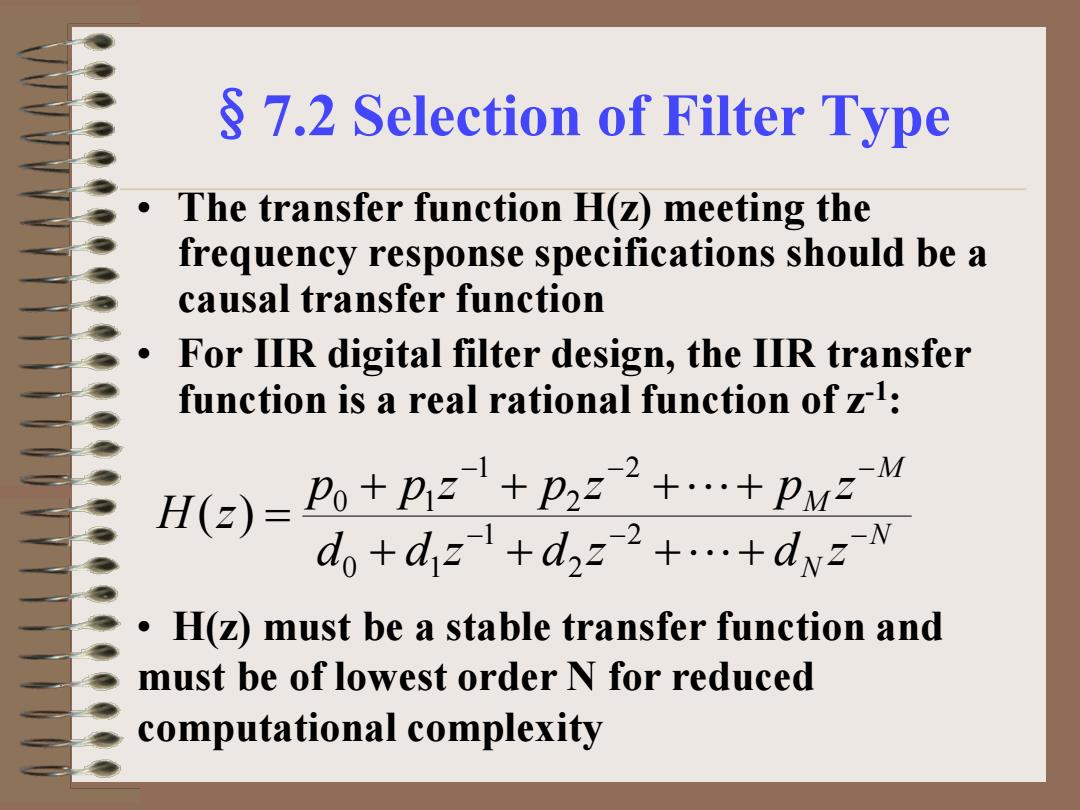
S 7.2 Selection of Filter Type The transfer function H(z)meeting the frequency response specifications should be a causal transfer function For IIR digital filter design,the IIR transfer function is a real rational function of z1: H(z)= +p2+p222+…+pM2M d4+dz+d2z2+…+dwzw H(z)must be a stable transfer function and must be of lowest order N for reduced computational complexity
§7.2 Selection of Filter Type • The transfer function H(z) meeting the frequency response specifications should be a causal transfer function • For IIR digital filter design, the IIR transfer function is a real rational function of z-1: N N M M d d z d z d z p p z p z p z H z − − − − − − + + + + + + + + = 2 2 1 0 1 2 2 1 0 1 ( ) • H(z) must be a stable transfer function and must be of lowest order N for reduced computational complexity

S 7.2 Selection of Filter Type For FIR digital filter design,the FIR transfer function is a polynomial in z1 with real coefficients: H(z)=∑hin]z-" n=0 For reduced computational complexity,degree N of H(z)must be as small as possible If a linear phase is desired,the filter coefficients must satisfy the constraint: h[n=±hN-n
§7.2 Selection of Filter Type For reduced computational complexity, degree N of H(z) must be as small as possible • If a linear phase is desired, the filter coefficients must satisfy the constraint: h[n] = ± h[N-n] = ∑ = − N n n H z h n z 0 ( ) [ ] • For FIR digital filter design, the FIR transfer function is a polynomial in z-1 with real coefficients:

7.2 Selection of Filter Type Advantages in using an FIR filter- (1)Can be designed with exact linear phase, (2)Filter structure always stable with quantized coefficients Disadvantages in using an FIR filter-Order of an FIR filter,in most cases,is considerably higher than the order of an equivalent IIR filter meeting the same specifications,and FIR filter has thus higher computational complexity
§7.2 Selection of Filter Type • Advantages in using an FIR filter - (1) Can be designed with exact linear phase, (2) Filter structure always stable with quantized coefficients • Disadvantages in using an FIR filter - Order of an FIR filter, in most cases, is considerably higher than the order of an equivalent IIR filter meeting the same specifications, and FIR filter has thus higher computational complexity