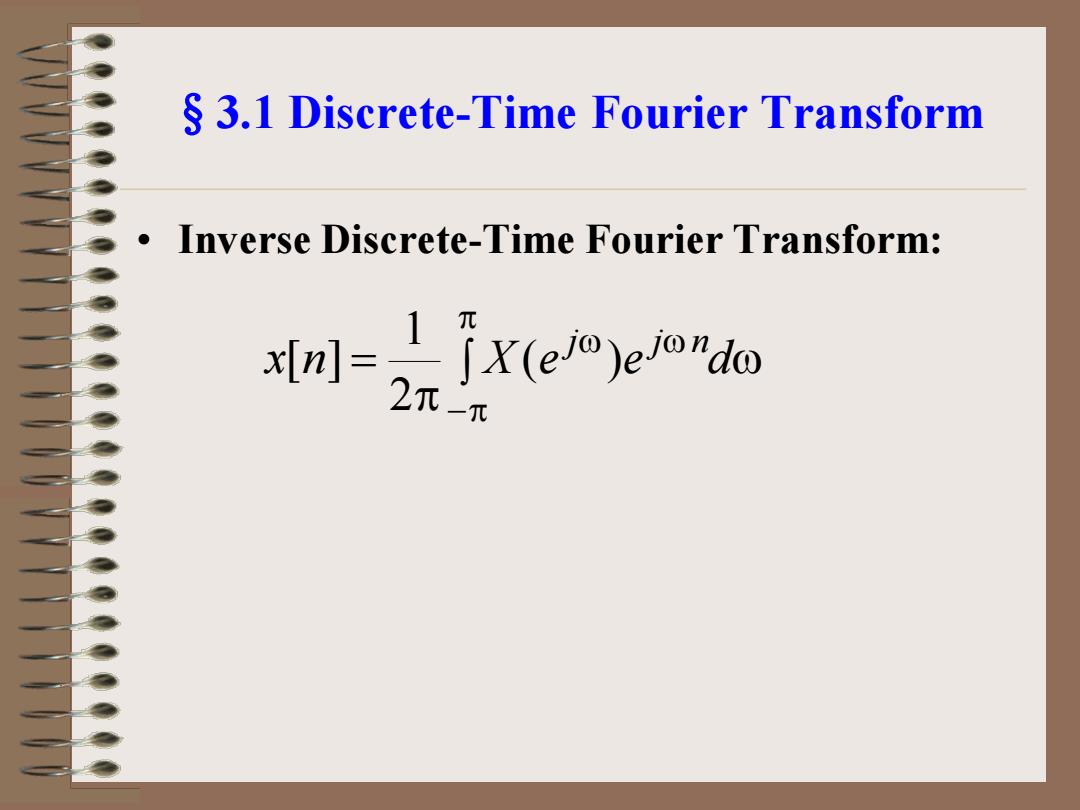
3.1 Discrete-Time Fourier Transform Inverse Discrete-Time Fourier Transform: ]-X(el)eordo
§3.1 Discrete-Time Fourier Transform • Inverse Discrete-Time Fourier Transform: ∫ ω π = π −π ω ω x n X e e d j j n ( ) 2 1 [ ]
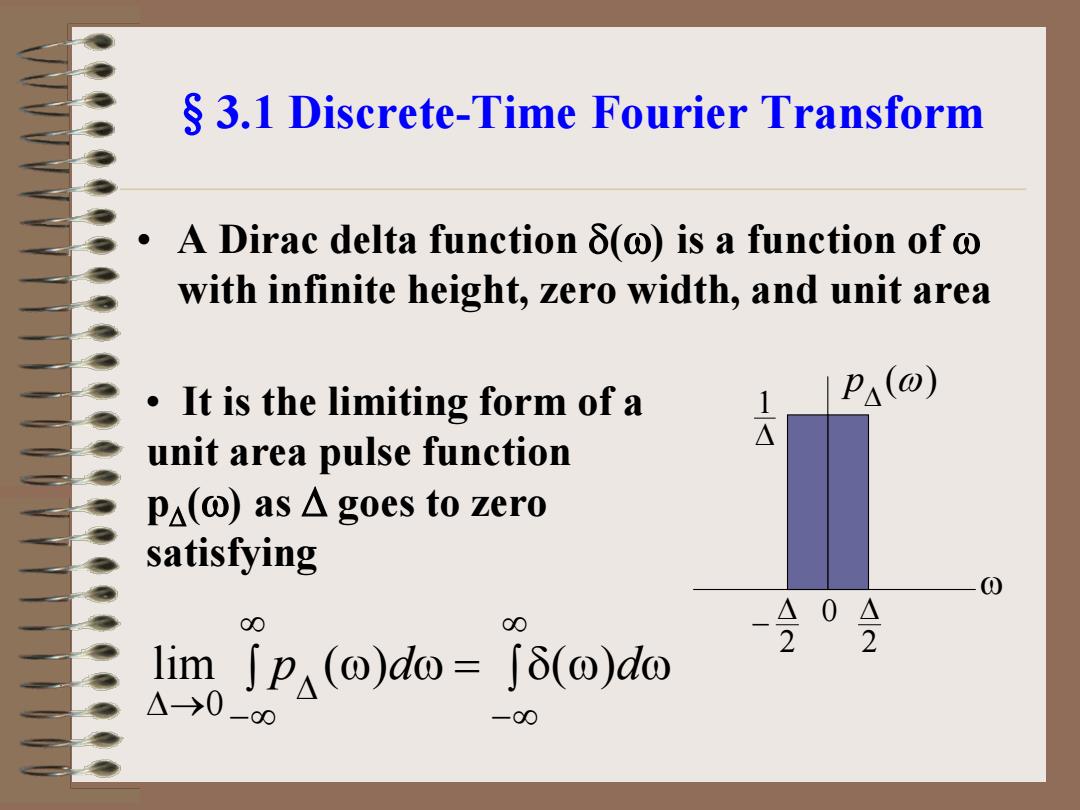
3.1 Discrete-Time Fourier Transform A Dirac delta function 8(@)is a function of o with infinite height,zero width,and unit area It is the limiting form of a P4() unit area pulse function Pa(o)as△goes to zero satisfying 0 0 00 2 △2 lim ∫p4(o)do=∫δ(o)do →0
§3.1 Discrete-Time Fourier Transform • A Dirac delta function δ(ω) is a function of ω with infinite height, zero width, and unit area ∫ ω ω = ∫ δ ω ω ∞ −∞ ∞ −∞ → ∆ ∆ lim p ( )d ( )d 0 ω 2 ∆ − 2 0 ∆ ∆ 1 (ω) ∆p • It is the limiting form of a unit area pulse function p∆(ω) as ∆ goes to zero satisfying
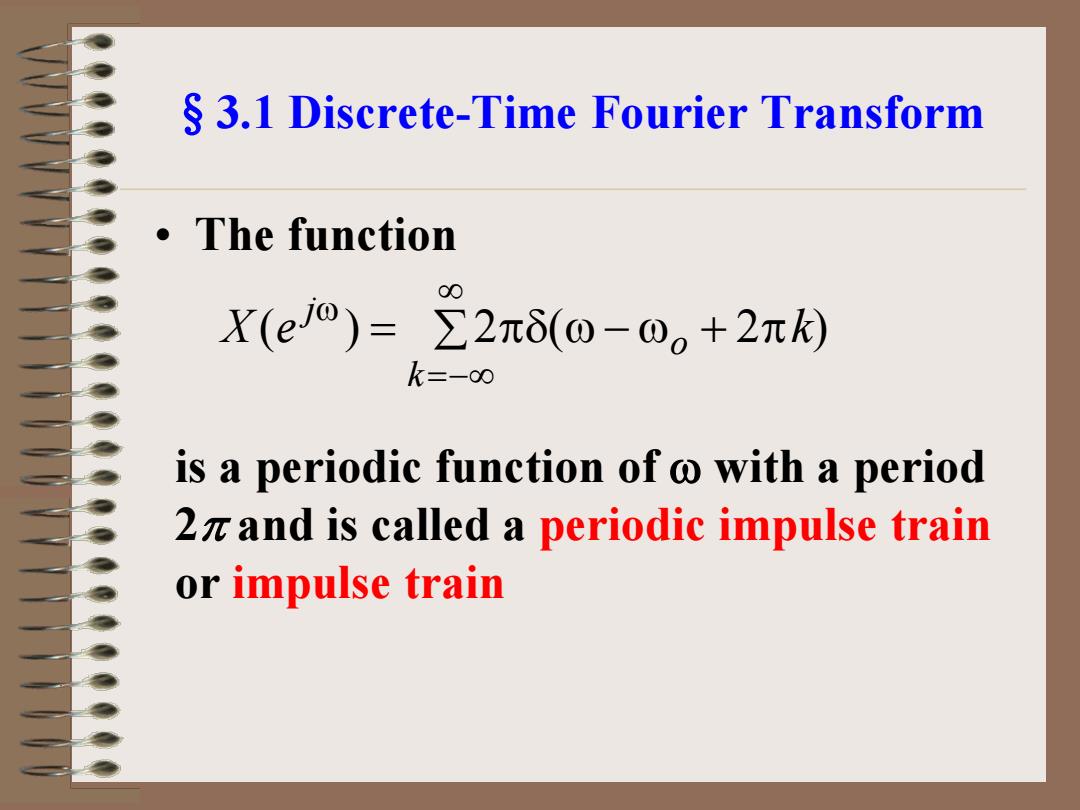
3.1 Discrete-Time Fourier Transform The function X(eo)=∑2πδ(o-0,+2元k) k=-00 is a periodic function of o with a period 2 and is called a periodic impulse train or impulse train
§3.1 Discrete-Time Fourier Transform is a periodic function of ω with a period 2π and is called a periodic impulse train or impulse train = ∑ πδ ω−ω + π ∞ =−∞ ω k o j X (e ) 2 ( 2 k) • The function

Commonly Used DTFT Pairs Sequence DTFT [n] ←> 1 1 > ∑2πδ(Q+2元k) k=-00 00 > ∑2πδ(0-0,+2πk) k=-00 1 00 K> +∑πδ(O+2πk) 1-e-jo k=-00 1 a"4nl,(<1) 1-ae-jo
Commonly Used DTFT Pairs Sequence DTFT δ[n] ↔ 1 ↔ ∑ πδ ω+ π ∞ k=−∞ 1 2 ( 2 k) ↔ ∑ πδ ω−ω + π ∞ =−∞ ω k o j n e k o 2 ( 2 ) + ∑πδ ω+ π − µ ↔ ∞ =−∞ − ω k j k e n ( 2 ) 1 1 [ ] ω α α µ α j n e n − − < ↔ 1 1 [ ], ( 1)

§3.2 DTFT Properties There are a number of important properties of the DTFT that are useful in signal processing applications These are listed here without proof Their proofs are quite straightforward We illustrate the applications of some of the DTFT properties
§3.2 DTFT Properties • There are a number of important properties of the DTFT that are useful in signal processing applications • These are listed here without proof • Their proofs are quite straightforward • We illustrate the applications of some of the DTFT properties