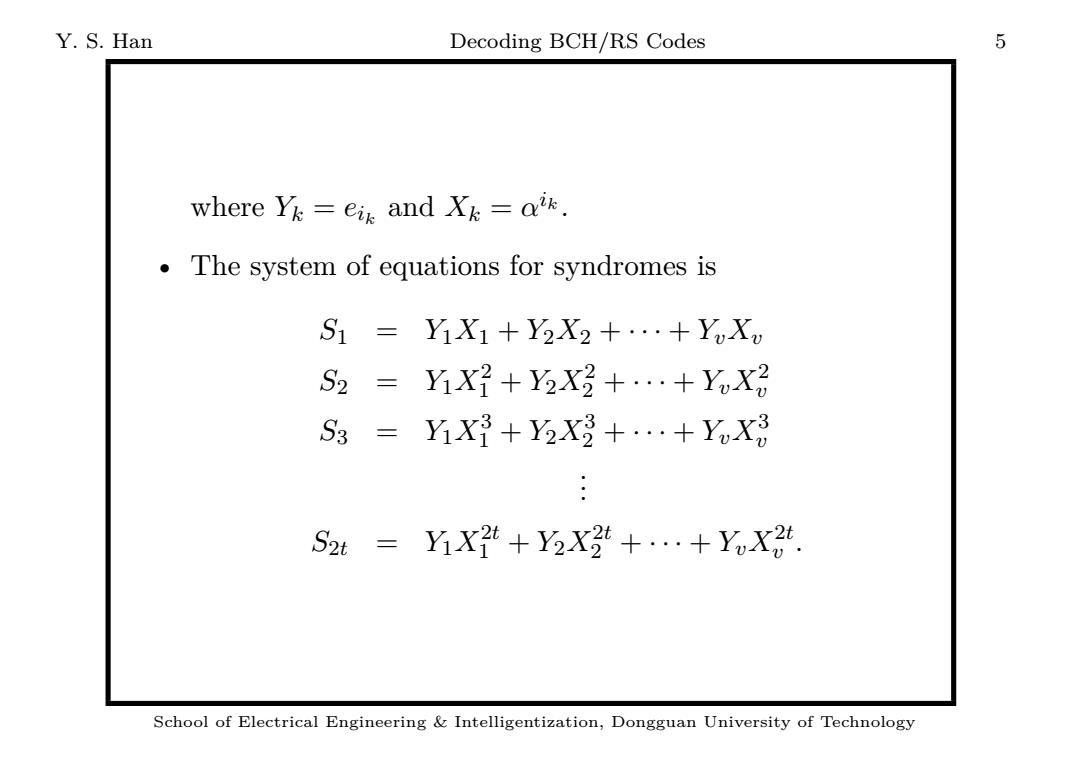
Y.S.Han Decoding BCH/RS Codes 5 where Y eig and Xk=aik. The system of equations for syndromes is S1=YX1+Y2X2+…+Y,X S2=X子+Y2X号+…+YX S3=YiX+Y2X+…+Y,X3 S2t=Yixi+Y2x2+..+Yox2t School of Electrical Engineering Intelligentization,Dongguan University of Technology
Y. S. Han Decoding BCH/RS Codes 5 where Yk = eik and Xk = α ik . • The system of equations for syndromes is S1 = Y1X1 + Y2X2 + · · · + YvXv S2 = Y1X2 1 + Y2X2 2 + · · · + YvX2 v S3 = Y1X3 1 + Y2X3 2 + · · · + YvX3 v . . . S2t = Y1X2t 1 + Y2X2t 2 + · · · + YvX2t v . School of Electrical Engineering & Intelligentization, Dongguan University of Technology

Y.S.Han Decoding BCH/RS Codes 6 Key Equation Recall that the error-locator polynomial is A()=(1-X1-xX2)…1-xX)=A0+∑Aa, where Ao=1. Define the infinite degree syndrome polynomial (though we only know the first 2t coefficients)as 0 S(x)= ∑S+1 j= j=0 k-1 School of Electrical Engineering Intelligentization,Dongguan University of Technology
Y. S. Han Decoding BCH/RS Codes 6 Key Equation • Recall that the error-locator polynomial is Λ(x) = (1 − xX1 )(1 − xX2 )· · ·(1 − xXv ) = Λ0 + ∑ v i=1 Λi x i , where Λ0 = 1. • Define the infinite degree syndrome polynomial (though we only know the first 2t coefficients) as S(x) = ∑ ∞ j=0 Sj+1x j = ∑ ∞ j=0 x j ( ∑ v k=1 YkX j+1 k ) School of Electrical Engineering & Intelligentization, Dongguan University of Technology
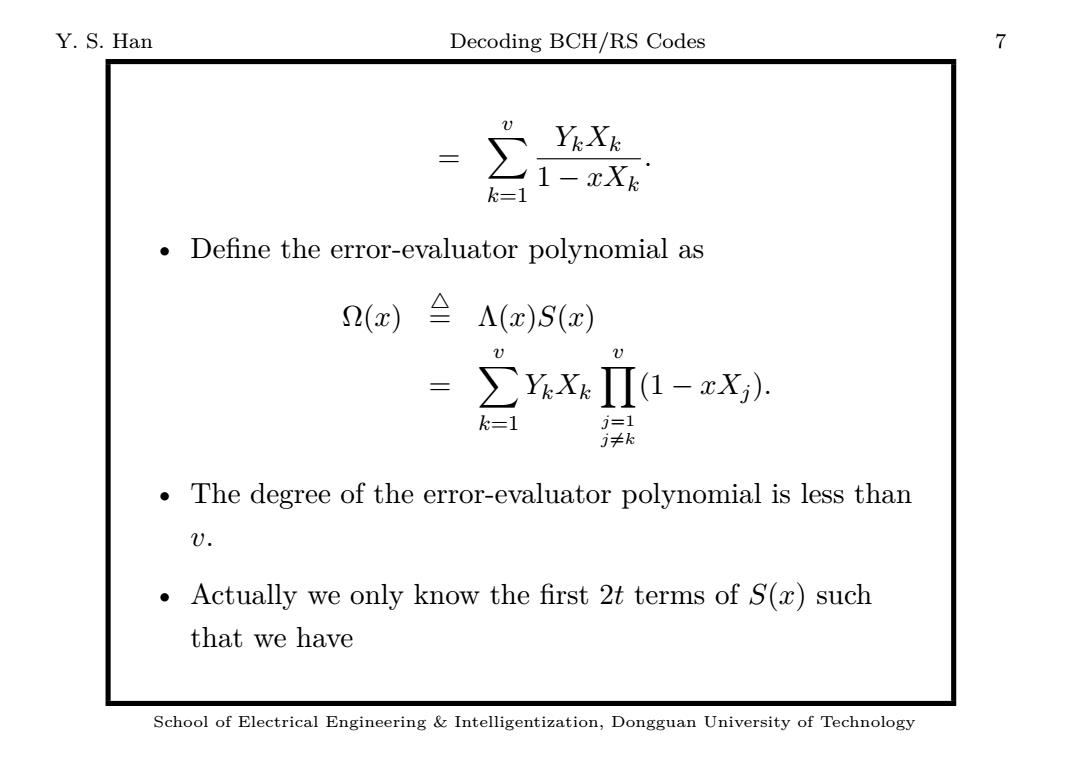
Y.S.Han Decoding BCH/RS Codes YhXk 1-xXk k=1 Define the error-evaluator polynomial as 2(x))AA()S() ∑KXkΠ1-xX) k= The degree of the error-evaluator polynomial is less than U. Actually we only know the first 2t terms of S(x)such that we have School of Electrical Engineering Intelligentization,Dongguan University of Technology
Y. S. Han Decoding BCH/RS Codes 7 = ∑ v k=1 YkXk 1 − xXk . • Define the error-evaluator polynomial as Ω(x) △ = Λ(x)S(x) = ∑ v k=1 YkXk ∏ v j=1 j̸=k (1 − xXj ). • The degree of the error-evaluator polynomial is less than v. • Actually we only know the first 2t terms of S(x) such that we have School of Electrical Engineering & Intelligentization, Dongguan University of Technology
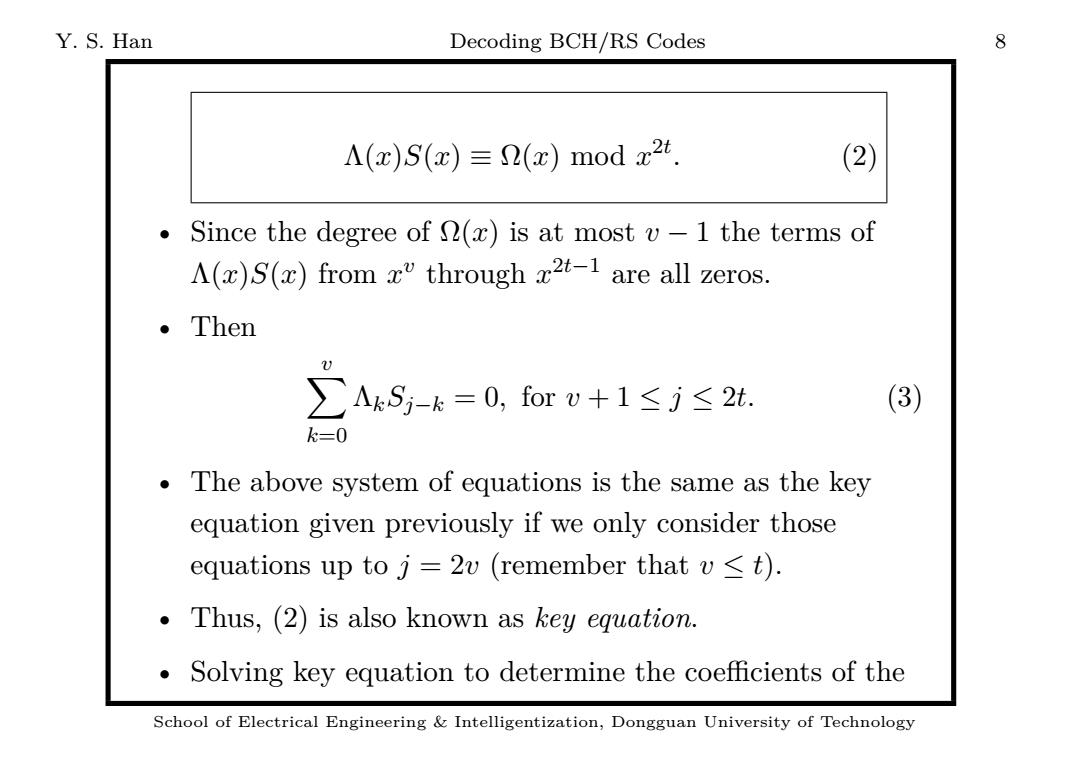
Y.S.Han Decoding BCH/RS Codes 8 A(x)S(x)=2(x)mod x24. (2) Since the degree of (x)is at most v-1 the terms of A()S(a)from through a2-1 are all zeros. 。Then ∑AkS)-k=0,foru+1≤j≤2t. (3) k=0 The above system of equations is the same as the key equation given previously if we only consider those equations up to j=2v(remember that v<t). Thus,(2)is also known as key equation. Solving key equation to determine the coefficients of the School of Electrical Engineering Intelligentization,Dongguan University of Technology
Y. S. Han Decoding BCH/RS Codes 8 Λ(x)S(x) ≡ Ω(x) mod x 2t . (2) • Since the degree of Ω(x) is at most v − 1 the terms of Λ(x)S(x) from x v through x 2t−1 are all zeros. • Then ∑ v k=0 Λk Sj−k = 0, for v + 1 ≤ j ≤ 2t. (3) • The above system of equations is the same as the key equation given previously if we only consider those equations up to j = 2v (remember that v ≤ t). • Thus, (2) is also known as key equation. • Solving key equation to determine the coefficients of the School of Electrical Engineering & Intelligentization, Dongguan University of Technology

Y.S.Han Decoding BCH/RS Codes 9 error-locator polynomial is a hard problem and it will be mentioned later. The key equation becomes A()(1+S(2))=2(2)mod 224+1 (4) if we define the infinite degree syndrome equation as (5) j=1 School of Electrical Engineering Intelligentization,Dongguan University of Technology
Y. S. Han Decoding BCH/RS Codes 9 error-locator polynomial is a hard problem and it will be mentioned later. • The key equation becomes Λ(x)(1 + S(x)) ≡ Ω(x) mod x 2t+1 (4) if we define the infinite degree syndrome equation as S(x) = ∑ ∞ j=1 Sj x j . (5) School of Electrical Engineering & Intelligentization, Dongguan University of Technology