
38 Mechanics of Materials 2 $2.5 The value of this expression will vary with the type of end condition;as an example,low carbon steel struts with pinned ends give L/k80. 2.5.Rankine or Rankine-Gordon formula As stated above,the Rankine formula is a combination of the Euler and crushing loads for a strut 111 PR=P。+P For very short struts Pe is very large;1/Pe can therefore be neglected and PR=Pe.For very long struts Pe is very small and 1/Pe is very large so that 1/Pc can be neglected.Thus PR=Pe. The Rankine formula is therefore valid for extreme values of L/k.It is also found to be fairly accurate for the intermediate values in the range under consideration.Thus,re-writing the formula in terms of stresses, 111 oA=aAayA ie. 1110e+0 一三一十一= 00y= Oy oe+oy [1+(oy/oe)] For a strut with both ends pinned π2E Ge= (L/k)2 Oy 0= Oy 1+2E Oy i.e.Rankine stress OR=1+a(L/k) (2.11) where a =ov/E,theoretically,but having a value normally found by experiment for various materials.This will take into account other types of end condition. Therefore Rankine load OyA PR=1+a(L/k) (2.12) Typical values of a for use in the Rankine formula are given in Table 2.3. However,since the values of a are not exactly equal to the theoretical values,the Rankine loads for long struts will not be identical to those estimated by the Euler theory as suggested earlier
38 Mechanics of Materials 2 $2.5 L - = JC?) k The value of this expression will vary with the type of end condition; as an example, low carbon steel struts with pinned ends give L/k fi 80. 2.5. Rankine or Rankine-Gordon formula As stated above, the Rankine formula is a combination of the Euler and crushing loads for a strut 111 +- PR Pe P, For very short struts P, is very large; 1/P, can therefore be neglected and PR = P,. For very long struts P, is very small and 1/P, is very large so that l/Pc can be neglected. Thus The Rankine formula is therefore valid for extreme values of L/k. It is also found to be fairly accurate for the intermediate values in the range under consideration. Thus, re-writing the formula in terms of stresses, _-_ PR = P,. 1 1 =-+- cA oeA a,A I - i.e. For a strut with both ends pinned (2.1 1) UY 1 + a(L/kI2 i.e. Rankine stress UR = where a = a,/n2E, theoretically, but having a value normally found by experiment for various materials. This will take into account other types of end condition. Therefore Rankine load (2.12) Typical values of a for use in the Rankine formula are given in Table 2.3. However, since the values of LI are not exactly equal to the theoretical values, the Rankine loads for long struts will not be identical to those estimated by the Euler theory as suggested earlier
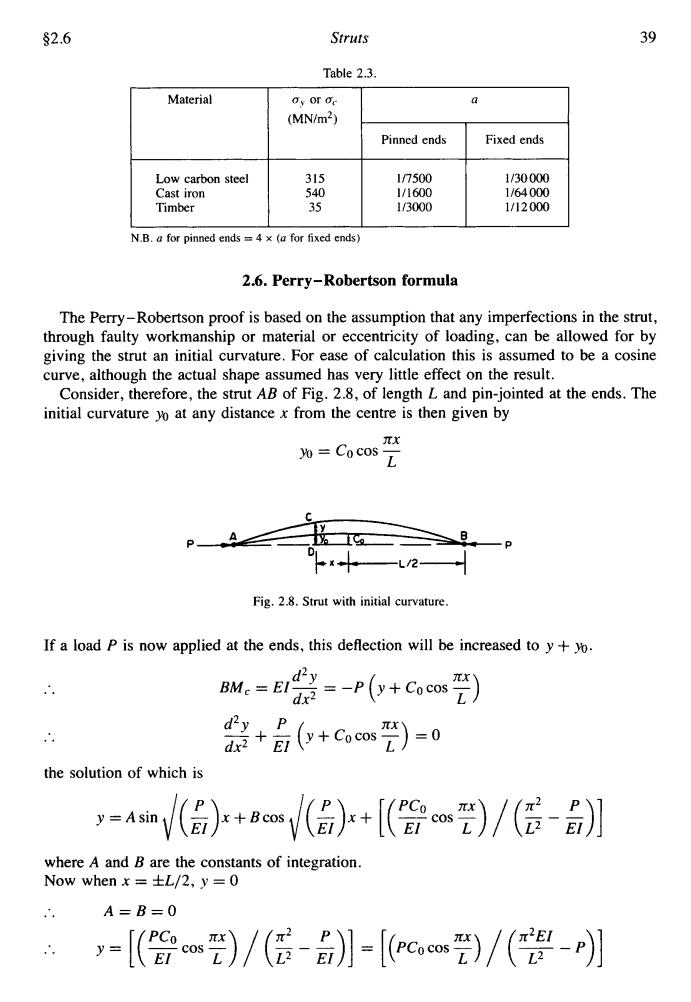
§2.6 Struts 39 Table 2.3. Material Oy or o a (MN/m2) Pinned ends Fixed ends Low carbon steel 315 17500 1/30000 Cast iron 540 1/1600 1/64000 Timber 35 1/3000 1/12000 N.B.a for pinned ends =4 x (a for fixed ends) 2.6.Perry-Robertson formula The Perry-Robertson proof is based on the assumption that any imperfections in the strut, through faulty workmanship or material or eccentricity of loading,can be allowed for by giving the strut an initial curvature.For ease of calculation this is assumed to be a cosine curve,although the actual shape assumed has very little effect on the result. Consider,therefore,the strut AB of Fig.2.8,of length L and pin-jointed at the ends.The initial curvature yo at any distance x from the centre is then given by πx yo=Co cos L -P -L2 Fig.2.8.Strut with initial curvature. If a load P is now applied at the ends,this deflection will be increased to y+yo. 8u:=狼=-P6+om) 2+后(+Gs咒)=0 the solution of which is y=Asin ()+a()+[(会)/(信-】 where A and B are the constants of integration. Now when x=±L/2,y=0 A=B=0 y=[(会o)/(信-司】-[ecw)/(提-P刃
$2.6 Material Low carbon steel Cast iron Timber Struts t~? or a,. a (MN/m2) Pinned ends Fixed ends 315 117500 1/3OOOO 540 1/1600 1 /64 000 35 1/3000 1/12OOO 39 2.6. Perry-Robertson formula The Perry-Robertson proof is based on the assumption that any imperfections in the strut, through faulty workmanship or material or eccentricity of loading, can be allowed for by giving the strut an initial curvature. For ease of calculation this is assumed to be a cosine curve, although the actual shape assumed has very little effect on the result. Consider, therefore, the strut AB of Fig. 2.8, of length L and pin-jointed at the ends. The initial curvature yo at any distance x from the centre is then given by TX yo = cocos - L Fig. 2.8. Strut with initial curvature. If a load P is now applied at the ends, this deflection will be increased to y + yo. .. "1 BMc=EI- d2 Y = -P(y+cocos- - + - (y + cocos "") L = 0 dx2 L d2y P dx2 EI the solution of which is y=Asin,/(&)x+Bcos,/(&)x+ [(%cos?) / (5 - k)] where A and B are the constants of integration. Now when x = fL/2, y = 0 .. A=B=O .. y= [($cos7)/($-;)] = [(Pcocos~)/(F-P)] T~EI