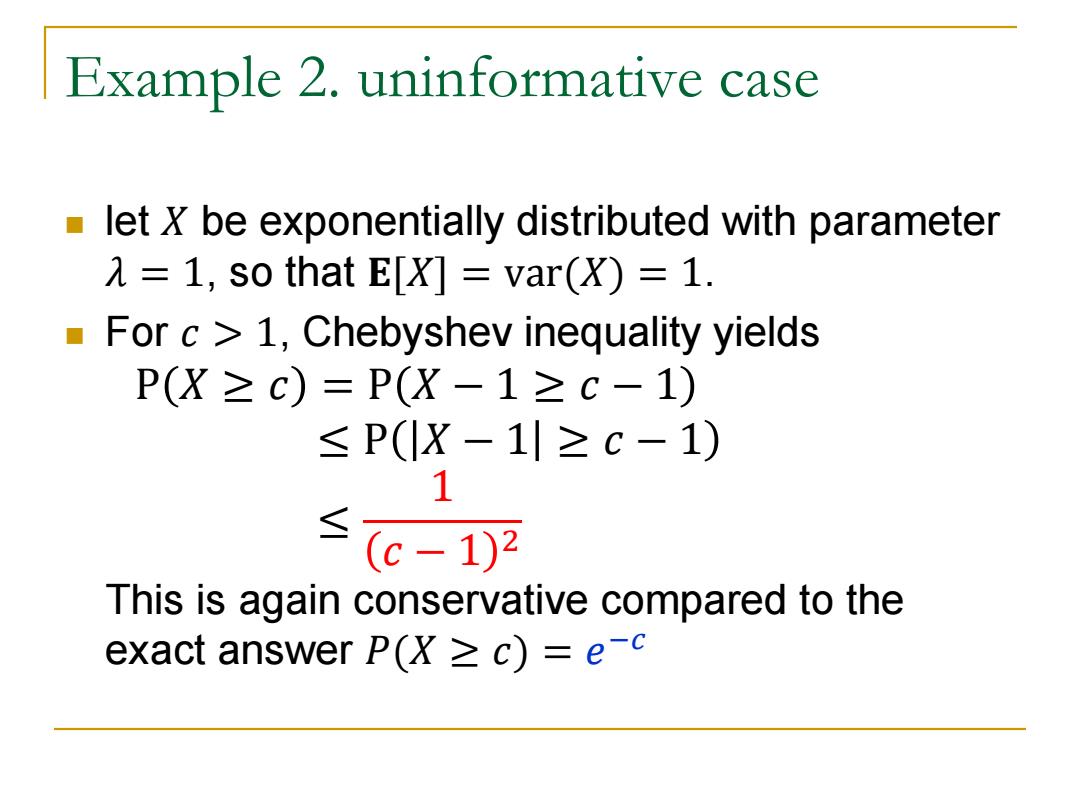
Example 2.uninformative case let X be exponentially distributed with parameter λ=1,so that E[X]=var(X)=1. For c 1,Chebyshev inequality yields P(X≥c)=P(X-1≥c-1) ≤P(X-1≥c-1) 1 (c-1)2 This is again conservative compared to the exact answer P(>c)=ec
Example 2. uninformative case let 𝑋 be exponentially distributed with parameter 𝜆 = 1, so that 𝐄[𝑋] = var(𝑋) = 1. For 𝑐 > 1, Chebyshev inequality yields P 𝑋 ≥ 𝑐 = P 𝑋 − 1 ≥ 𝑐 − 1 ≤ P 𝑋 − 1 ≥ 𝑐 − 1 ≤ 1 𝑐 − 1 2 This is again conservative compared to the exact answer 𝑃(𝑋 ≥ 𝑐) = 𝑒 −𝑐

Example 3.Upper Bounds -When X is known to take values in a range [a,b],we claim that o2 (b-a)2/4. If o2 is unknown,we may use the bound (b-a)2/4 in the Chebyshev inequality, (b-a)2 P(IX-川≥c)≤ for all c 0 4c2
Example 3. Upper Bounds When 𝑋 is known to take values in a range [𝑎, 𝑏], we claim that 𝜎 2 ≤ 𝑏 − 𝑎 2 /4. If 𝜎 2 is unknown, we may use the bound 𝑏 − 𝑎 2 /4 in the Chebyshev inequality, P 𝑋 − 𝜇 ≥ 𝑐 ≤ 𝑏 − 𝑎 2 4𝑐2 , for all 𝑐 > 0
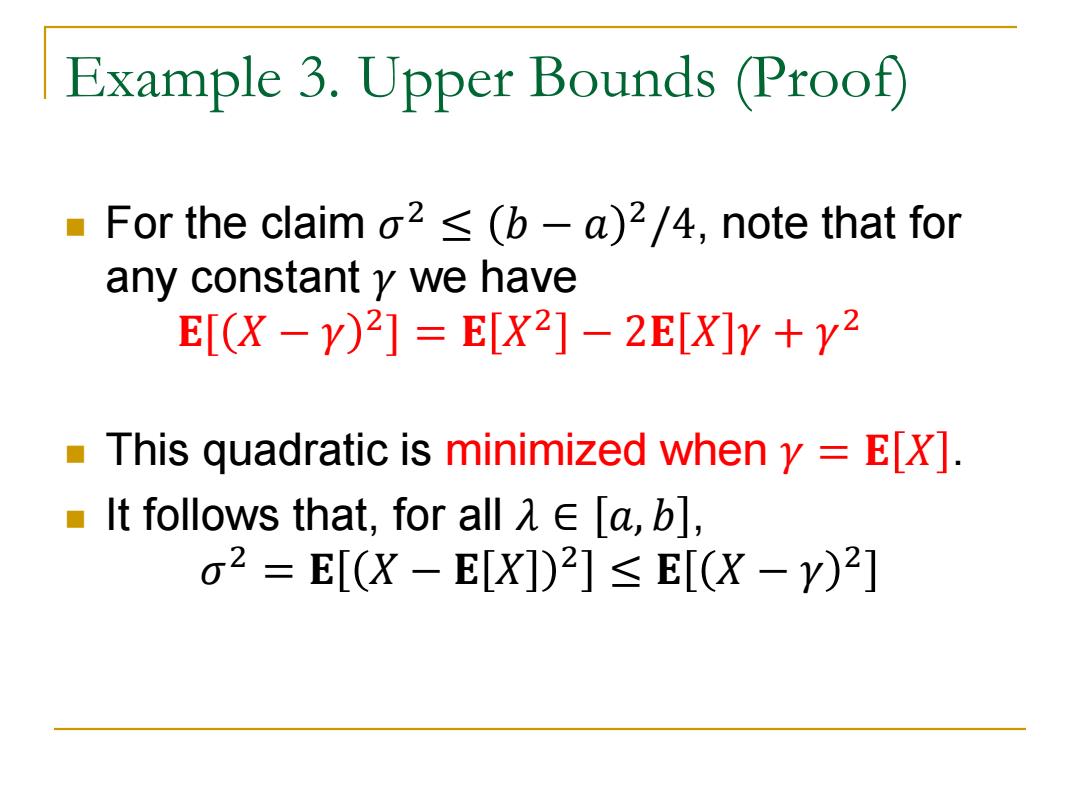
Example 3.Upper Bounds (Proof) For the claim o2 <(b-a)2/4,note that for any constant y we have E[(X-Y)2]=EX2]-2E[X]y+Y2 This quadratic is minimized when y E[X]. It follows that,.for allλ∈[a,b], o2=E[(X-EX])2]≤E[(X-Y)2]
Example 3. Upper Bounds (Proof) For the claim 𝜎 2 ≤ 𝑏 − 𝑎 2 /4, note that for any constant 𝛾 we have 𝐄[ 𝑋 − 𝛾 2 ] = 𝐄 𝑋 2 − 2𝐄 𝑋 𝛾 + 𝛾 2 This quadratic is minimized when 𝛾 = 𝐄 𝑋 . It follows that, for all 𝜆 ∈ 𝑎, 𝑏 , 𝜎 2 = 𝐄 𝑋 − 𝐄 𝑋 2 ≤ 𝐄 𝑋 − 𝛾 2

Example 3.Upper Bounds (Proof) By letting y=(a +b)/2,we obtain 2≤(x-2)] =E[(X-a0X-b】+b-a2≤-a2 4 4 The equality above is verified by calculation, The last inequality follows from the fact (x-a)(x-b)≤0 Vx∈[a,b]
Example 3. Upper Bounds (Proof) By letting 𝛾 = (𝑎 + 𝑏)/2, we obtain 𝜎 2 ≤ 𝐄 𝑋 − 𝑎+𝑏 2 2 = 𝐄 𝑋 − 𝑎 𝑋 − 𝑏 + 𝑏−𝑎 2 4 ≤ 𝑏−𝑎 2 4 The equality above is verified by calculation, The last inequality follows from the fact 𝑥 − 𝑎 𝑥 − 𝑏 ≤ 0 ∀𝑥 ∈ 𝑎, 𝑏
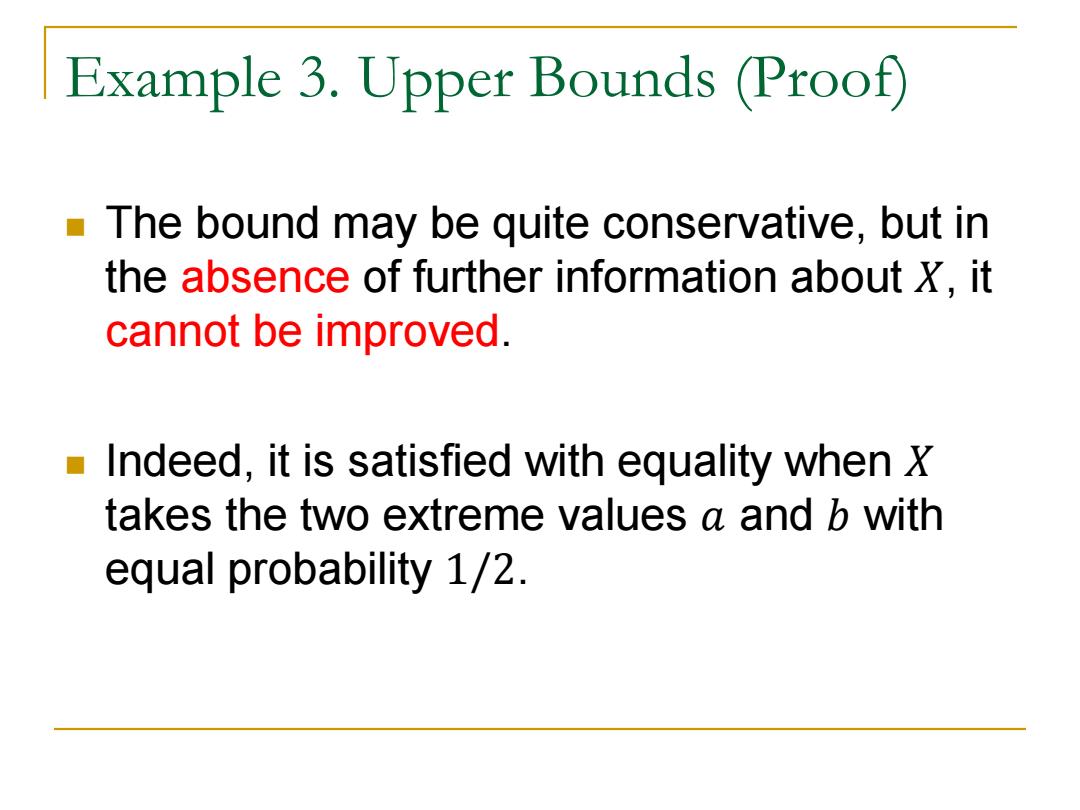
Example 3.Upper Bounds (Proof) The bound may be quite conservative,but in the absence of further information about X.it cannot be improved. Indeed,it is satisfied with equality when X takes the two extreme values a and b with equal probability 1/2
Example 3. Upper Bounds (Proof) The bound may be quite conservative, but in the absence of further information about 𝑋, it cannot be improved. Indeed, it is satisfied with equality when 𝑋 takes the two extreme values 𝑎 and 𝑏 with equal probability 1/2