
ENGG430Protaistics onnrs Chapter 5:Limit Theorems Instructor: Shengyu Zhang
Instructor: Shengyu Zhang

Content Markov and Chebyshev Inequalities The Weak Law of Large Numbers ■( Convergence in Probability The Central Limit Theorem The Strong Law of Large Numbers
Content ◼ Markov and Chebyshev Inequalities ◼ The Weak Law of Large Numbers ◼ Convergence in Probability ◼ The Central Limit Theorem ◼ The Strong Law of Large Numbers
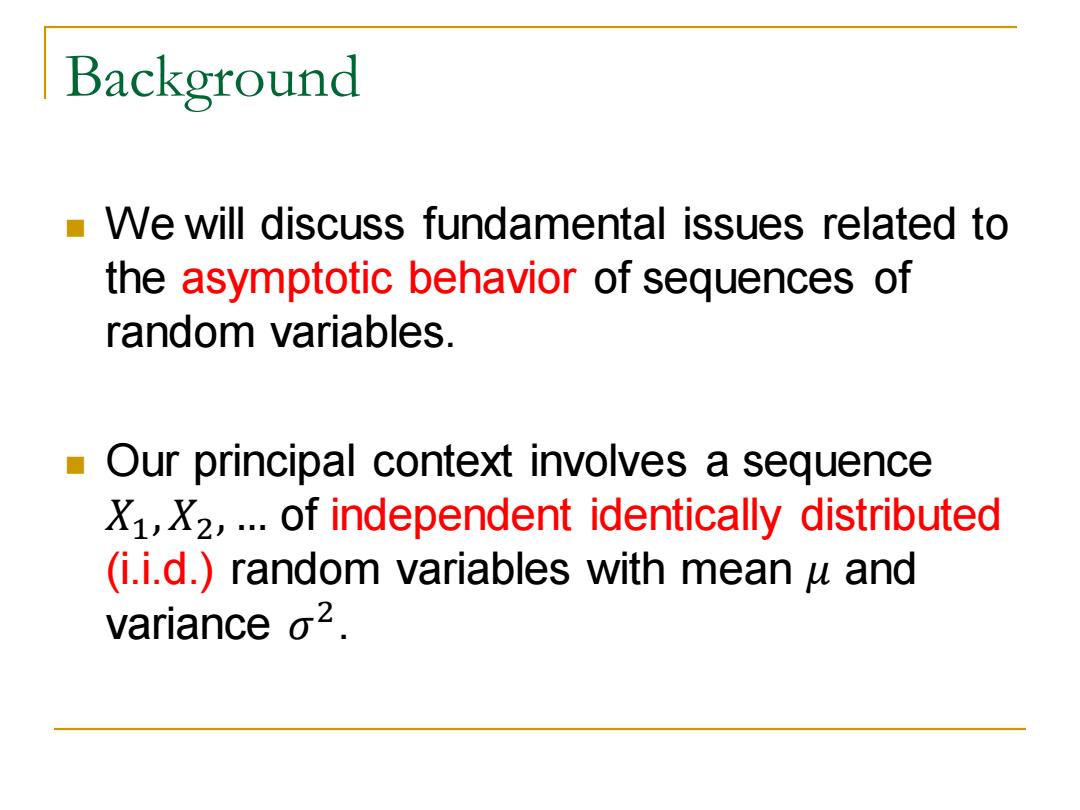
Background We will discuss fundamental issues related to the asymptotic behavior of sequences of random variables. Our principal context involves a sequence X1,X2,...of independent identically distributed (i.i.d.)random variables with mean w and variance o2
Background ◼ We will discuss fundamental issues related to the asymptotic behavior of sequences of random variables. ◼ Our principal context involves a sequence 𝑋1,𝑋2, … of independent identically distributed (i.i.d.) random variables with mean 𝜇 and variance 𝜎 2
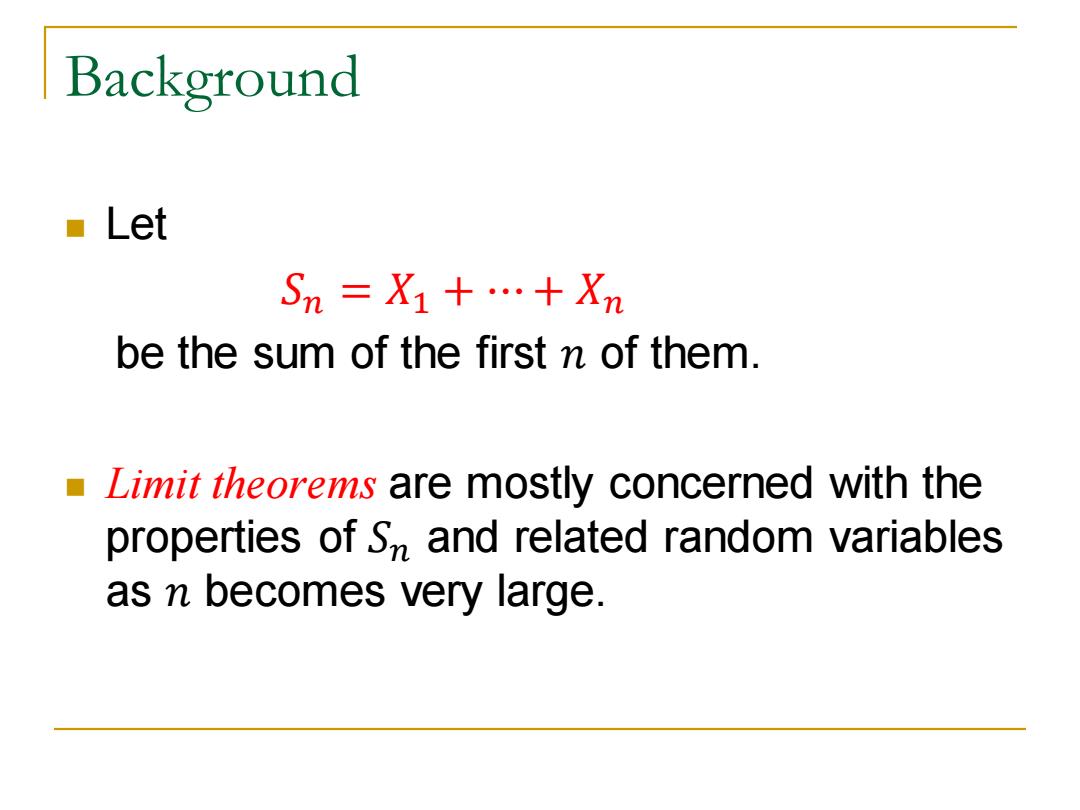
Background Let Sn=X1+…+Xn be the sum of the first n of them. Limit theorems are mostly concerned with the properties of S and related random variables as n becomes very large
Background ◼ Let 𝑆𝑛 = 𝑋1 + ⋯ + 𝑋𝑛 be the sum of the first 𝑛 of them. ◼ Limit theorems are mostly concerned with the properties of 𝑆𝑛 and related random variables as 𝑛 becomes very large
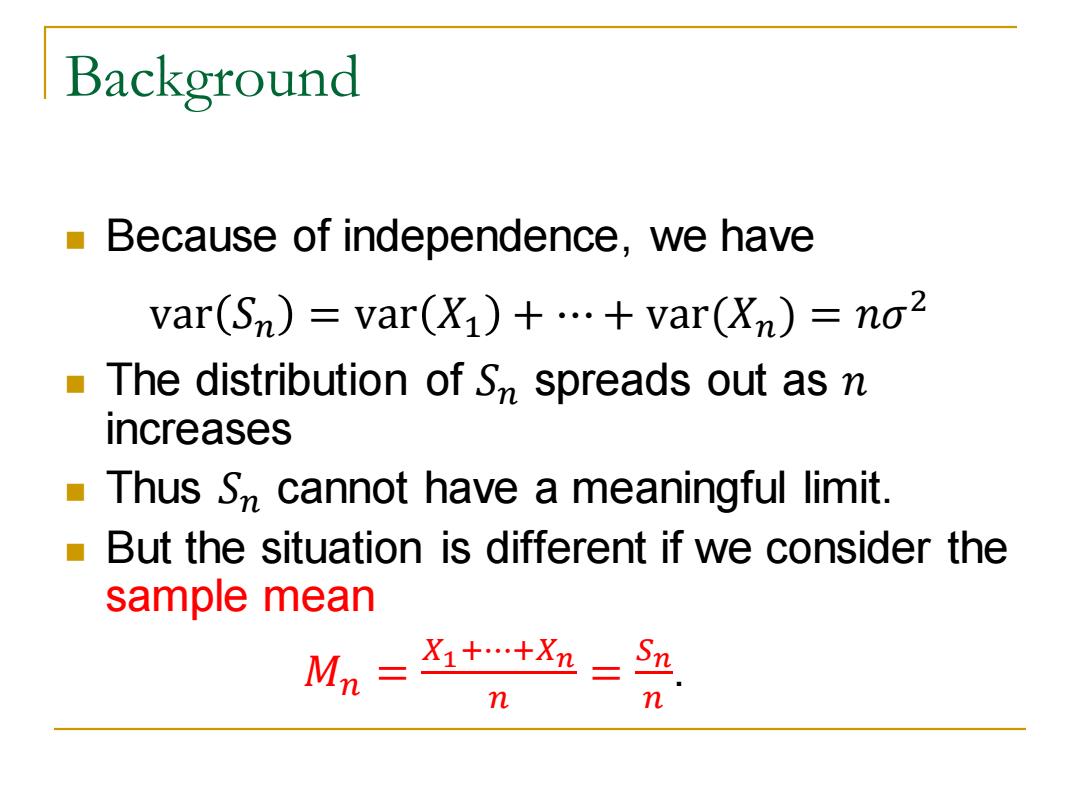
Background Because of independence,we have var(Sn)=var(X1)+.+var(Xn)=no2 The distribution of S spreads out as n increases Thus S cannot have a meaningful limit. But the situation is different if we consider the sample mean Mn= X1++Xn Sn n n
Background ◼ Because of independence, we have var 𝑆𝑛 = var 𝑋1 + ⋯ + var(𝑋𝑛) = 𝑛𝜎 2 ◼ The distribution of 𝑆𝑛 spreads out as 𝑛 increases ◼ Thus 𝑆𝑛 cannot have a meaningful limit. ◼ But the situation is different if we consider the sample mean 𝑀𝑛 = 𝑋1+⋯+𝑋𝑛 𝑛 = 𝑆𝑛 𝑛