上海交通大学：《材料加工原理 Principles of Materials Processing》教学资源（课程讲稿）03. Technology of plasticity——Elements of plastic theory

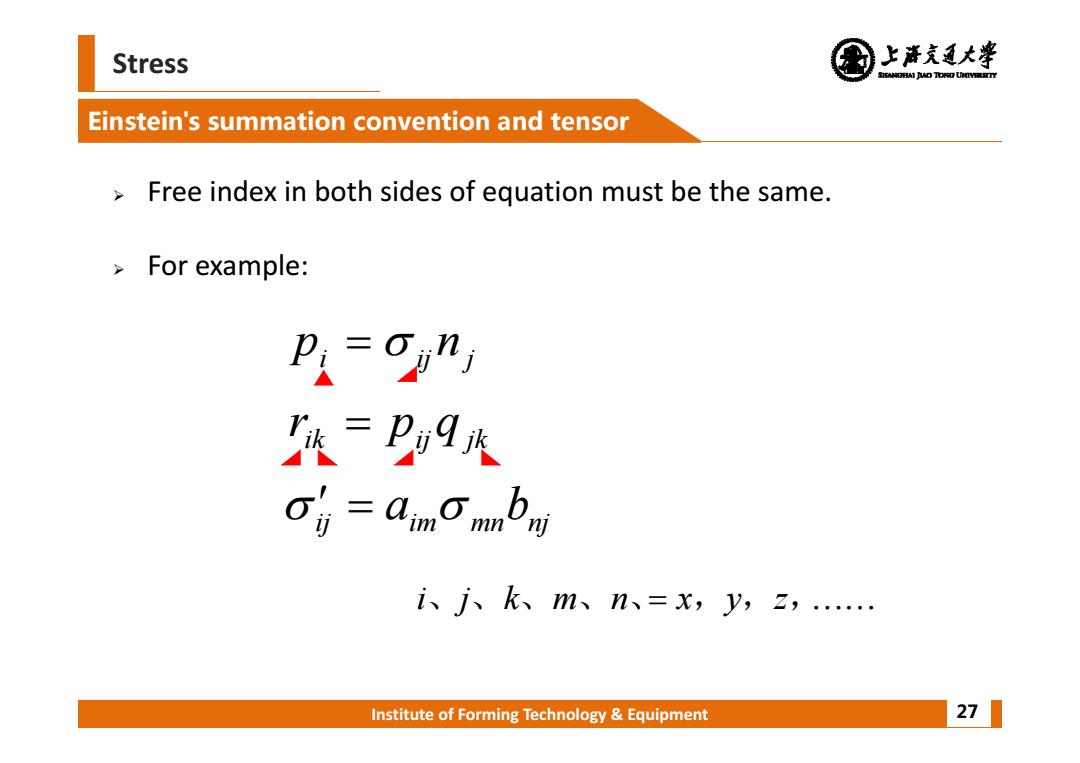
Stress 上清充通大￥ SHEAMGHAI DUD TONO UHTVEREETTY Einstein's summation convention and tensor Free index in both sides of equation must be the same. For example: k=卫9及 i、j、k、m、n、=x,y,z,. Institute of Forming Technology Equipment 27

Institute of Forming Technology & Equipment Stress Einstein's summation convention and tensor 27  Free index in both sides of equation must be the same.  For example: i ij j p   n ik ij jk r  p q ij im mn nj   a  b i、j、k、m、n、 x，y，z，

Stress 国上清文通大￥ SANCEAI JUO TO阳UY Einstein's summation convention and tensor Exercise:formula expansion o月=anm0mnb、六m、n、=l,2,3) 012=a11O1b12+412021b2+a13031b2 +41O12b22+a12022b22+a13032b22 +a411O13b32+412023b32+a13O33b32 023=？ 8,=a+u) where Oui Wi,j ai ,take derivative Institute of Forming Technology Equipment 28

Institute of Forming Technology & Equipment Stress Einstein's summation convention and tensor 28  Exercise: formula expansion  ij  aim mnbnj  (i、j、m、n、1，2，3) 12  a1111b12   a12 21b12 13 31 12  a  b  a1112b22  a12 22b22 13 32 22  a  b 11 13 32  a  b 12 23 32  a  b 13 33 32  a  b ?  23    ij ui, j u j,i 21    ju u i i j  where ,  ,: take derivative

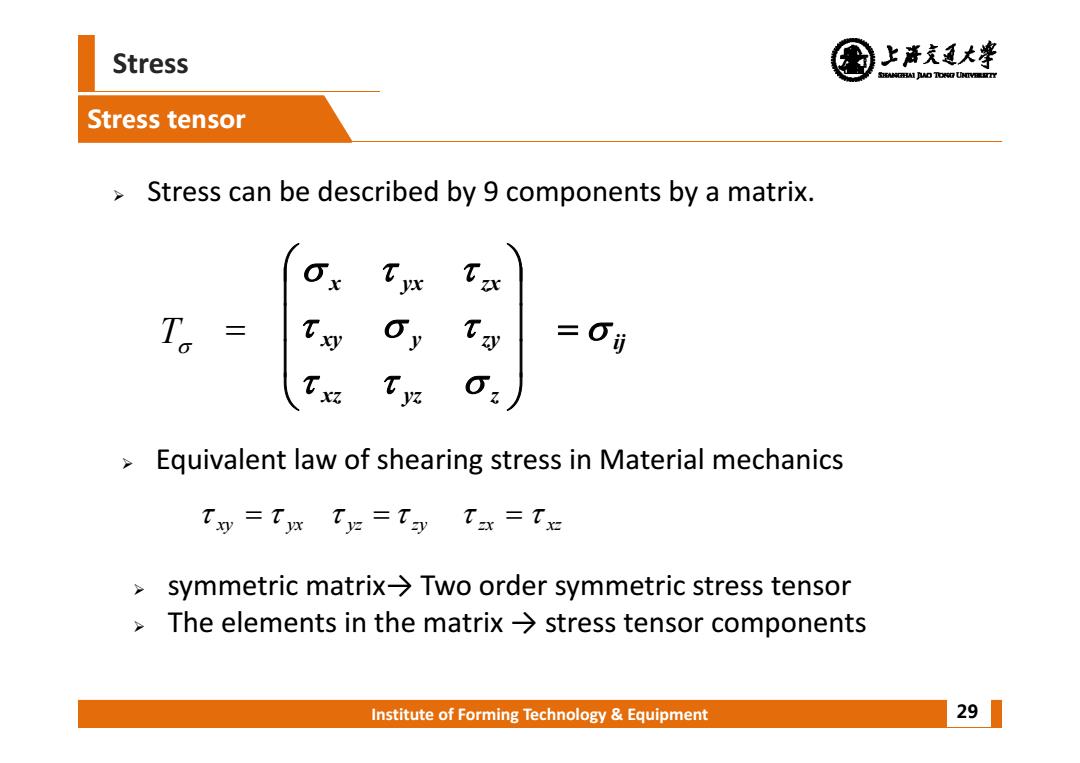
Stress 国上清充大￥ SHANCBHAI JUAO TONO UNTVEEETTY Stress tensor > Stress can be described by 9 components by a matrix. Ta Equivalent law of shearing stress in Material mechanics Ty=Tx Ty=ty Tx=Tx symmetric matrix Two order symmetric stress tensor The elements in the matrix>stress tensor components Institute of Forming Technology Equipment 29

Institute of Forming Technology & Equipment Stress Stress tensor 29  Stress can be described by 9 components by a matrix.   xz yz z xy y zy x yx zx          T    ij xy yx    yz zy   zx xz     Equivalent law of shearing stress in Material mechanics  symmetric matrix→ Two order symmetric stress tensor  The elements in the matrix → stress tensor components

Stress 上产克大睾 SHANCBHAI JUAO TONO UNTVEEETTY Stress tensor A scalar>by the magnitude of the number (Temperature,mass and energy) A vector>the magnitude/size and direction of the number (force,velocity) a directed line segment A tensor>the magnitude,direction,and orientation of the number (stress tensor and strain rate tensor) Scalar:in the axis;positive and negative;no coordinate transformation;Zero order tensor. Vector:decomposed;With the selection of the coordinate system->the component changes;coordinate transformation;first order tensor. Tensor:a set of vectors;magnitude,direction and the relationship between vectors;related to the selection of coordinate system; coordinate transformation Institute of Forming Technology Equipment 30

Institute of Forming Technology & Equipment Stress Stress tensor 30  A scalar → by the magnitude of the number (Temperature, mass and energy)  A vector → the magnitude/size and direction of the number (force, velocity) a directed line segment  A tensor → the magnitude, direction, and orientation of the number (stress tensor and strain rate tensor)  Scalar: in the axis; positive and negative; no coordinate transformation; Zero order tensor.  Vector: decomposed; With the selection of the coordinate system→ the component changes; coordinate transformation; first order tensor.  Tensor: a set of vectors; magnitude, direction and the relationship between vectors; related to the selection of coordinate system; coordinate transformation

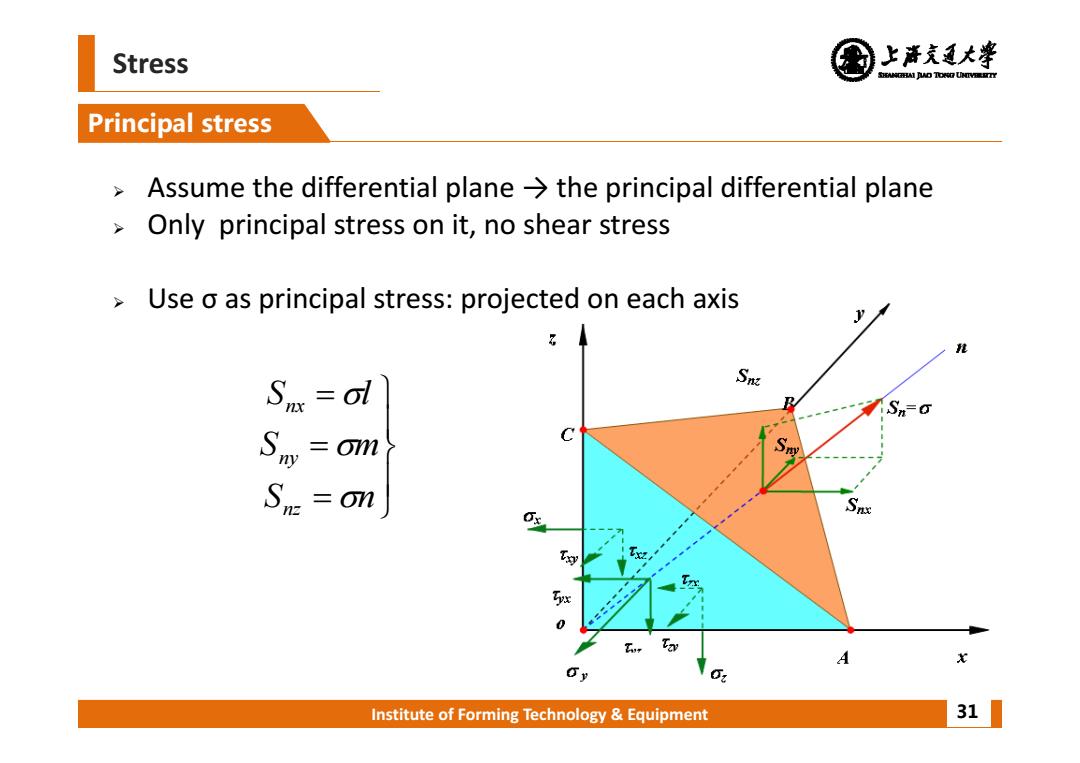
Stress 上清充通大￥ SHEAMGHAI DUD TONO UHTVEREETTY Principal stress Assume the differential plane>the principal differential plane Only principal stress on it,no shear stress Use o as principal stress:projected on each axis S R =om S=on x 0 A 6元 Institute of Forming Technology Equipment 31

Institute of Forming Technology & Equipment Stress Principal stress 31      S n S m S l nz ny nx     Assume the differential plane → the principal differential plane  Only principal stress on it, no shear stress  Use σ as principal stress: projected on each axis