上海交通大学：《现代控制理论》课程教学资源（讲稿）Chapter 3 Linear algebra

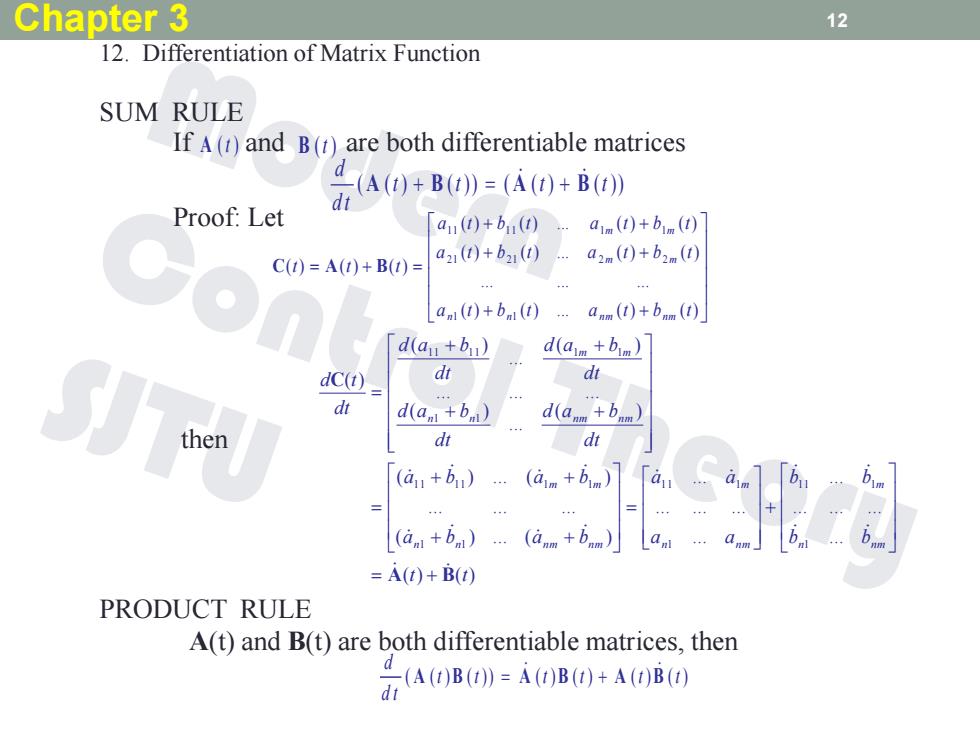
Chapter 3 12 12.Differentiation of Matrix Function SUM RULE If A()and B(t)are both differentiable matrices A(0)+B)=(A()+B(》 di Proof:Let a0)+b1(0… aim(1)+bim(t) C(t)=A()+B(t)= a21(t)+b21() a2m()+b2m() an(1)+b(t).. anm(I)+bnm(t)） d(an+bu) d(aim+bim) dC①= dr dt dt d(an+b) d(abm) then di dt (a1+b)… (am+bm) = (an+bn）… (ann+bnm)】 an =A(t)+B(t) PRODUCT RULE A(t)and B(t)are both differentiable matrices,then AOB》=A)B)+AB d

12. Differentiation of Matrix Function SUM RULE If and are both differentiable matrices A (t ) B (t ) ( (t ) (t ) ) ( (t ) (t ) ) d t d A B A B    Proof: Let                      ( ) ( ) ... ( ) ( ) ... ... ... ( ) ( ) ... ( ) ( ) ( ) ( ) ... ( ) ( ) ( ) ( ) ( ) 1 1 2 1 2 1 2 2 1 1 1 1 1 1 a t b t a t b t a t b t a t b t a t b t a t b t t t t n n n m n m m m m m C A B then ( ) ( ) ... ... ... ... ... ... ... ... ... ... ( ) ... ( ) ... ... ... ( ) ... ( ) ( ) ... ( ) ... ... ... ( ) ... ( ) ( ) 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 1 t t b b b b a a a a a b a b a b a b dt d a b dt d a b dt d a b dt d a b dt d t n n m m n n m m n n n m n m m m n n n m n m m m A B C                                                                           PRODUCT RULE A(t) and B(t) are both differentiable matrices, then ( (t ) (t ) ) (t ) (t ) (t ) (t ) d t d A B A B A B     Chapter 3 12

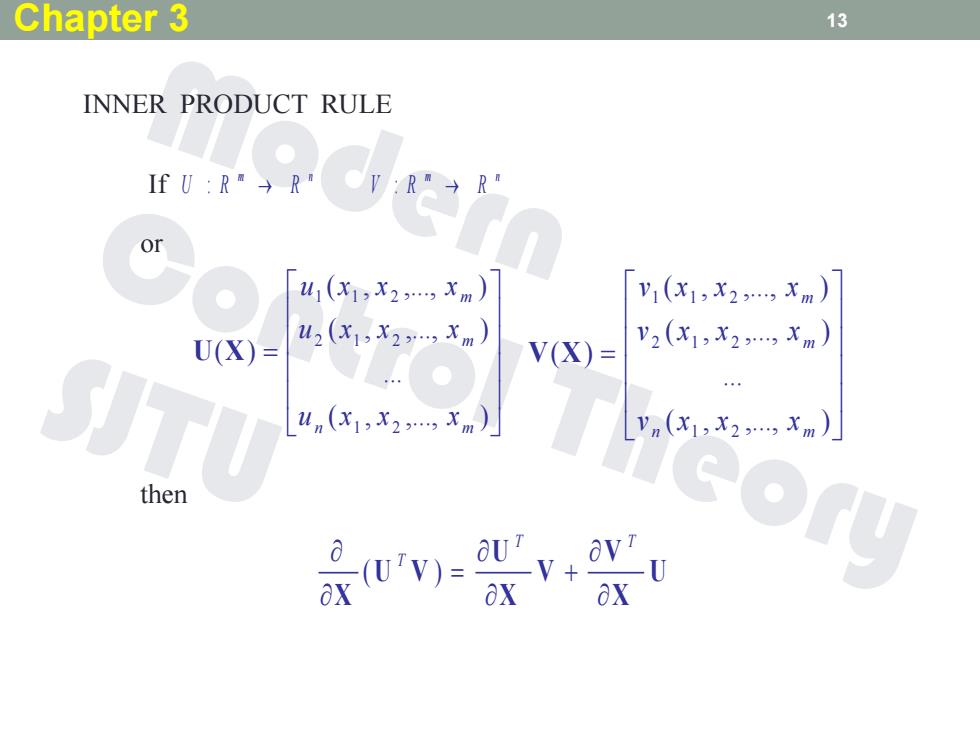
Chapter 3 13 INNER PRODUCT RULE IfU:R"→R' V RR or 41(X1,x2,Xm) V(1,x22..xm) U(X)= 2(X1,X2,Xm） V(X)= V2(x1,X2,Xm） un(X1,x2>xm) Vn(X1,2,Xm)】 then ax (U'v)= eory -V+ U OX

INNER PRODUCT RULE If m n m n U : R  R V : R  R or              ( , ,..., ) ... ( , ,..., ) ( , ,..., ) ( ) 1 2 2 1 2 1 1 2 n m m m u x x x u x x x u x x x U X              ( , ,..., ) ... ( , ,..., ) ( , ,..., ) ( ) 1 2 2 1 2 1 1 2 n m m m v x x x v x x x v x x x V X then U X V V X U U V X         T T T ( ) Chapter 3 13

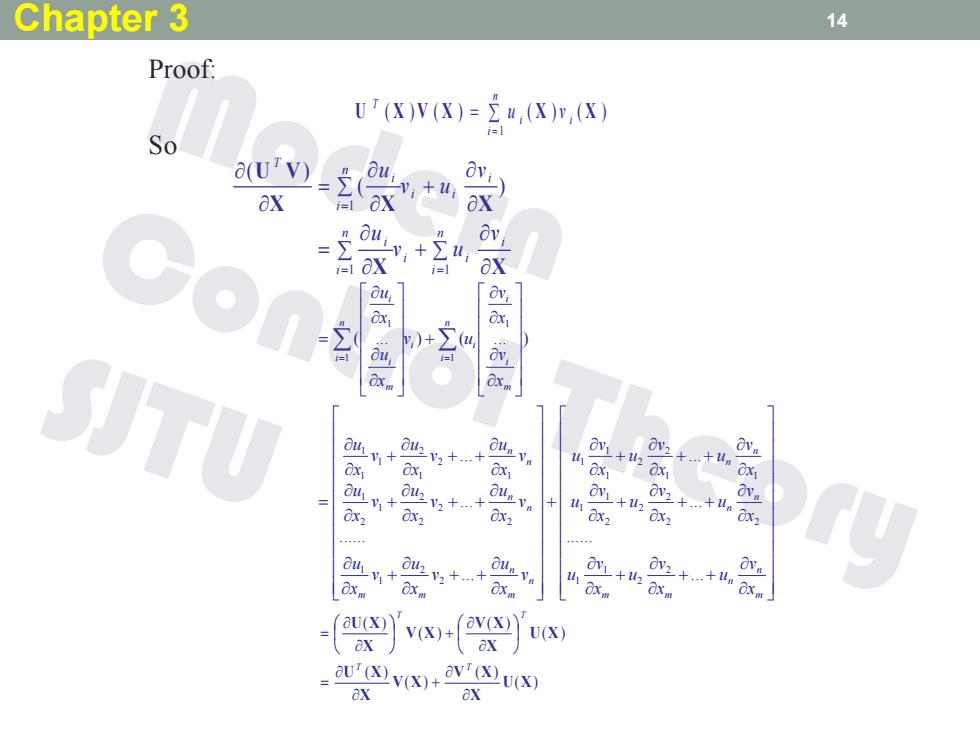
Chapter 3 14 Proof: UT(X)V(X)=u,(X)",(X) So a(U'v) OX aX Bv OX Ov 武 )+2 x1 SJTU 0xm ay2++ Oun 02 0 Ox1 山0x +…+ln OV' a + 0x7 0x2 0,++ OV+uz OVa 41 X xm axm】 aU(x) v8)+ax】 av(x) U(X) -0U70XV(X)V(X)U(X) ax X

So Proof:  ni i i T u v 1 U ( X ) V ( X ) ( X ) ( X )                 n i i i i n i i i i i n i i T v v u u v v u u 1 1 1 ( ) ( ) X X X X X U V                                                    mn n m m n n n n n mn m m n n n nmii i ni i ni mii xv u xv u xv u xv u xv u xv u xv u xv u xv u v xu v xu v xu v xu v xu v xu v xu v xu v xu xvxv v u xuxu ... ...... ... ... ... ...... ... ... ( ... ) ( ... ) 2 2 1 1 2 2 2 2 21 1 1 1 2 2 11 1 2 2 1 1 2 2 22 1 21 1 2 12 1 11 1 1 1 1 ( ) ( ) ( ) ( ) ( ) ( ) ( ) ( ) U X X V X V X X U X U X XV X V X XU X                  T T T T Chapter 3 14