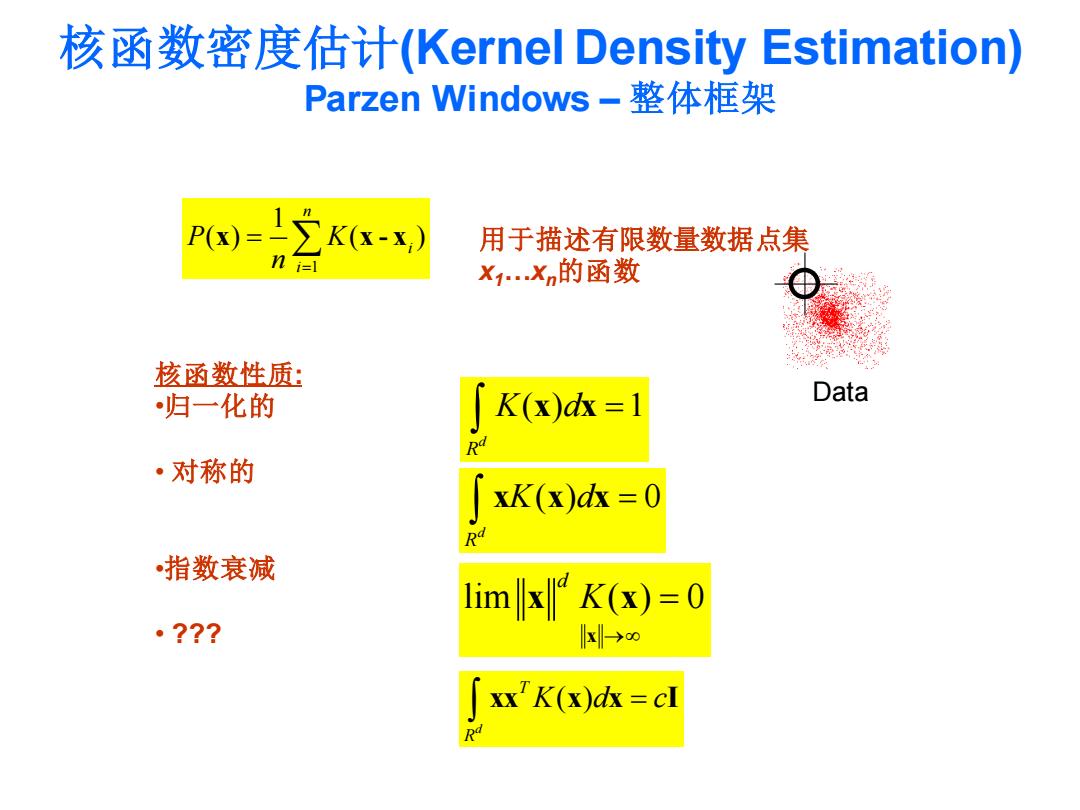
核函数密度估计(Kernel Density Estimation) Parzen Windows-整体框架 P)=月∑K(-) 用于描述有限数量数据点集 X1.…Xn的函数 核函数性质: 归一化的 K(x)dx =1 Data ·对称的 xK(x)dx =0 指数衰减 limK(x)=0 。?? x→o xx'K(x)dx =cI
核函数密度估计(Kernel Density Estimation) Parzen Windows – 整体框架 1 1 ( ) ( ) n i i P K n = x x - x = 核函数性质: •归一化的 • 对称的 •指数衰减 • ??? ( ) 1 d R K d = x x ( ) 0 d R K d = x x x lim ( ) 0 d K → = x x x ( ) d T R K d c = xx x x I 用于描述有限数量数据点集 x1…xn的函数 Data
f'(x) m(xg) f(x) K(x) Xi G(x) Xk x

Example:Given a set of five data points x1 =2,x2 =2.5,x3 =3,x4 1 and x5=6,find Parzen probability density function(pdf)estimates at x =3,using the Gaussian function with o 1 as window function. Solution: ( 0.3989 =云0(2)-02420 0.0540 0.0044 so 20(25p)-03521 p(x=3)=(0.2420+0.3521+0.3989 +0.0540+0.0044)/5=0.2103
Example: Given a set of five data points x1 = 2, x2 = 2.5, x3 = 3, x4 = 1 and x5 = 6, find Parzen probability density function (pdf) estimates at x = 3, using the Gaussian function with σ = 1 as window function

0.6 0.5 人 0.4 0.3 0. 0.1 -0.1 -0.2 -2 -1 0 2

核函数密度估计(Kernel Density Estimation Parzen Windows-函数形式 P)=∑K(-) 用于描述有限数量数据点集 ni=】 X1Xn的函数 Data 在实际应用时经常使用的函数形式为: K(x)=ck(x)or K(x)=ck(x) 在各个数据纬度上使用相同的核函数 建立关于向量长度的核函数
1 1 ( ) ( ) n i i P K n = x x - x = Data 在实际应用时经常使用的函数形式为: 1 ( ) ( ) d i i K c k x = x = or K ck ( ) x x = ( ) 在各个数据纬度上使用相同的核函数 建立关于向量长度的核函数 核函数密度估计(Kernel Density Estimation) Parzen Windows – 函数形式 用于描述有限数量数据点集 x1…xn的函数