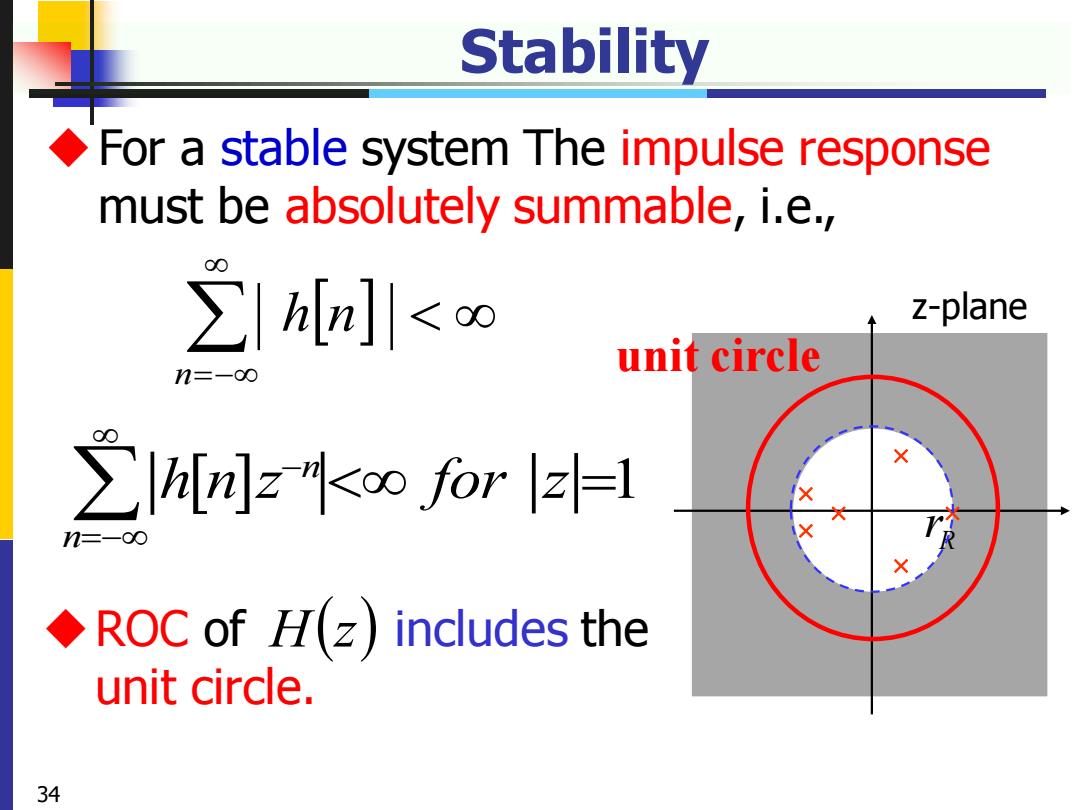
Stability For a stable system The impulse response must be absolutely summable,i.e., ]< z-plane unit circle n=-00 ∑刊z<oo for IzH1 ROC of H(z)includes the unit circle. 34
Stability n=− h n 1 n n h n z for z − =− = ◆ROC of includes the unit circle. H(z) ◆For a stable system The impulse response must be absolutely summable, i.e., 34 z-plane R r unit circle
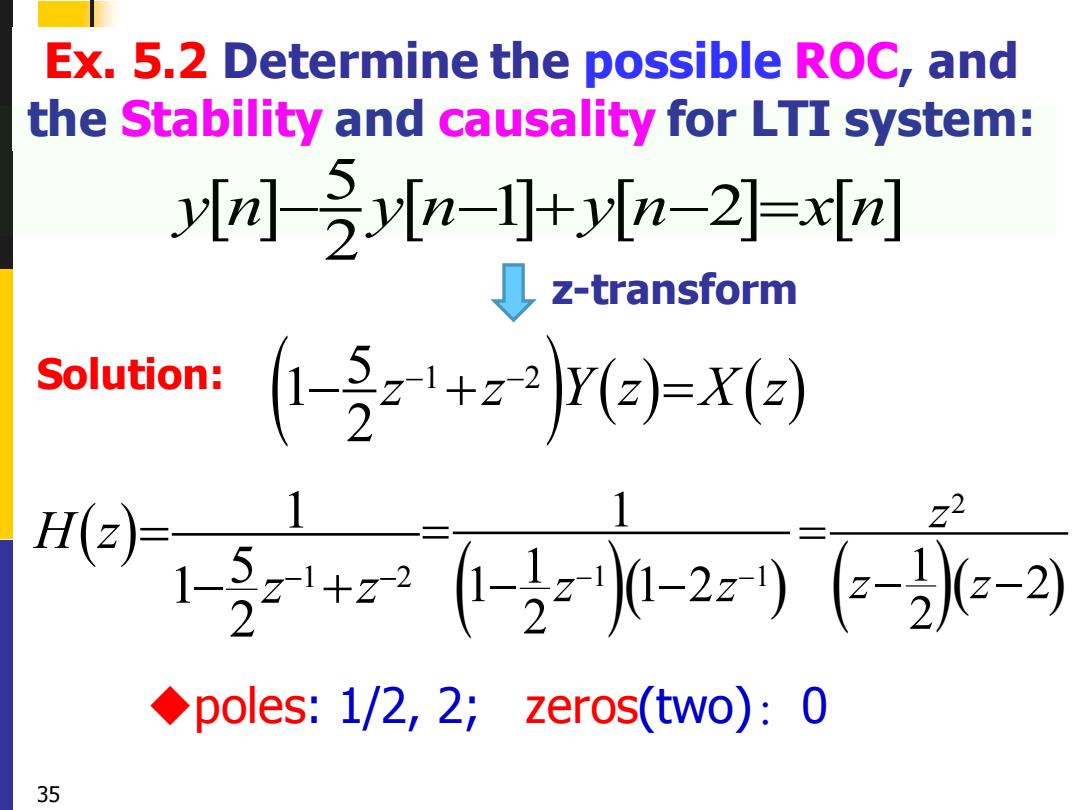
Ex.5.2 Determine the possible ROC,and the Stability and causality for LTI system: [闪号n-+yn-2]=xn ↓z-transform Solution: ((2)-X() H2= 之2 13+x2(-20-2x)-2e-2) poles:1/2,2;zeros(two):0 35
Ex. 5.2 Determine the possible ROC, and the Stability and causality for LTI system: 5 1 2 2 y n y n y n x n − − + − = ◆poles: 1/2, 2; zeros(two):0 Solution: 35 ( ) ( ) ( ) 5 1 2 1 2 z z Y z X z − − − + = ( ) 1 2 1 5 1 2 H z z z − − = − + ( )( ) 1 1 1 1 1 1 2 2 z z − − = − − z-transform ( )( ) 2 1 2 2 z z z = − −
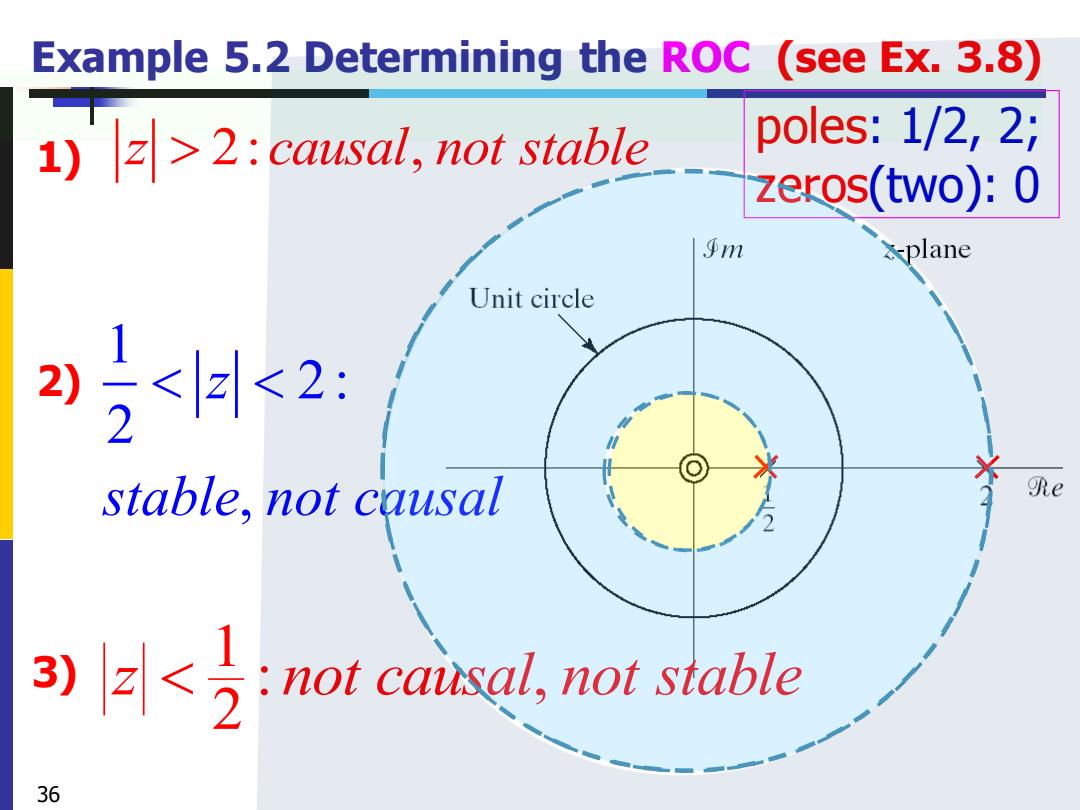
Example 5.2 Determining the ROC (see Ex.3.8) 1)>2:causal,not stable poles:1/2,2; zeros(two):0 乎m <plane Unit circle 2) 2 stable,not causal 欧e not caial,not stable 36
Example 5.2 Determining the ROC (see Ex. 3.8) z causal not stable 2: , 1 2 : 2 , z stable not causal 1 : , 2 z not causal not stable 1) 2) 3) 36 poles: 1/2, 2; zeros(two): 0
Causal and Stable system Causal:ROC must be outside the outermost pole. Stable:ROC includes the unit circle. Causal and stable:all the poles of the system function are inside the unit circle; ROC is outside the outermost pole,and includes the unit circle. 37
Causal and Stable system ◆Causal: ROC must be outside the outermost pole. ◆Stable: ROC includes the unit circle. ◆Causal and stable: all the poles of the system function are inside the unit circle; ROC is outside the outermost pole, and includes the unit circle. 37
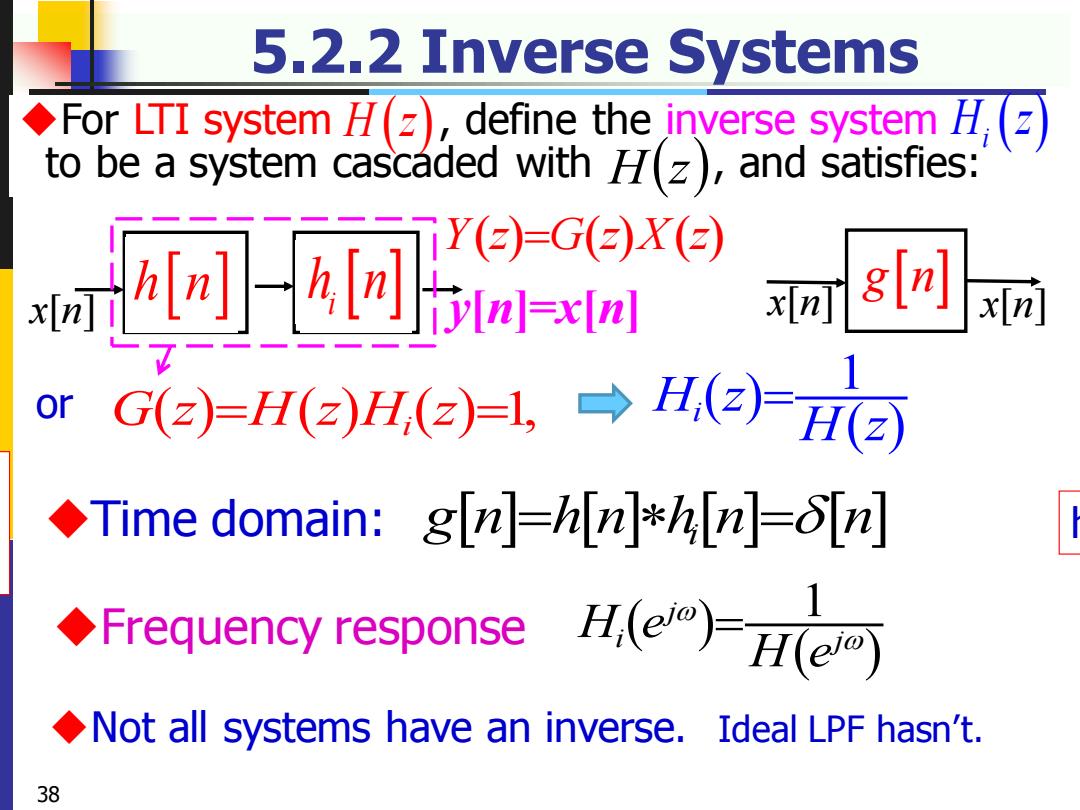
5.2.2 Inverse Systems For LTI system H(),define the inverse system H ( to be a system cascaded with H(z),a and satisfies: Y(e=G(a)X(e) nxinl xn] or G(2=H(H,()=1, →H(2)FHa ◆Time domain::g[n]=h[n]*h[n]=δ[n] ◆Frequency response' ee可 Not all systems have an inverse.Ideal LPF hasn't. 38
5.2.2 Inverse Systems ( ) ( ) ( ) 1, G z H z H z = = i ( ) ( ) 1 H zi H z = ◆Time domain: g n h n h n n = = i ◆Not all systems have an inverse. Ideal LPF hasn’t. ◆For LTI system , define the inverse system to be a system cascaded with , and satisfies: H z( ) ( ) H z i H(z) ( ) ( ) j 1 H ei H ej ◆Frequency response = x[n] y[n]=x[n] H z( ) ( ) H z i x[n] x[n] h n G z( ) i h n g n 38 or Y z G z X z ( )= ( ) ( ) ( ) 1, | | 0, | | j c lp c H e = has