Chapter 8 The Discrete Fourier Transform ◆8.0 Introduction 8.1 Representation of Periodic Sequence:the Discrete Fourier Series(DFS) 8.2 Properties of the DFS 8.3 The Fourier Transform of Periodic Signal 8.4 Sampling the Fourier Transform(DTFT) 8.5 Fourier Representation of Finite-Duration Sequence:the Discrete Fourier Transform(DFT) 8.6 Properties of the DFT 8.7 Linear Convolution using the DFT 8.8 the discrete cosine transform (DCT
2 Chapter 8 The Discrete Fourier Transform ◆8.0 Introduction ◆8.1 Representation of Periodic Sequence: the Discrete Fourier Series (DFS) ◆8.2 Properties of the DFS ◆8.3 The Fourier Transform of Periodic Signal ◆8.4 Sampling the Fourier Transform(DTFT) ◆8.5 Fourier Representation of Finite-Duration Sequence: the Discrete Fourier Transform(DFT) ◆8.6 Properties of the DFT ◆8.7 Linear Convolution using the DFT ◆8.8 the discrete cosine transform (DCT)
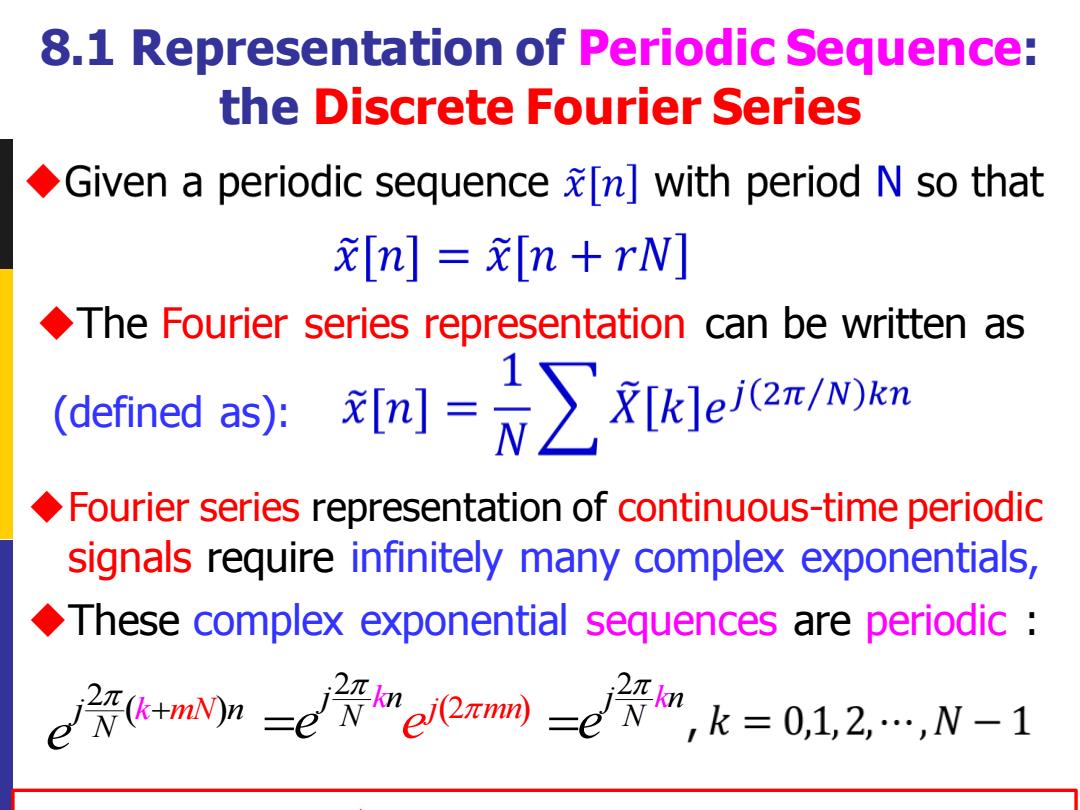
8.1 Representation of Periodic Sequence: the Discrete Fourier Series Given a periodic sequence [n]with period N so that [n]=n +rN] The Fourier series representation can be written as (defined as): 网=表∑]e2wm Fourier series representation of continuous-time periodic signals require infinitely many complex exponentials, These complex exponential sequences are periodic g2k+m0n=e8e2m=e/3,k=01,2,N-1
6 ◆Fourier series representation of continuous-time periodic signals require infinitely many complex exponentials, ◆These complex exponential sequences are periodic : 8.1 Representation of Periodic Sequence: the Discrete Fourier Series ( ) 2 j k N N m n e + ◆The Fourier series representation can be written as 2 j n N k e = ( ) 2 2 j n N k j mn e e = (defined as): (2 / ) ( ) ( ) , j t k T k k x t x t a T e + =− + = = 1 (2 / ) ( ) jk T t k T T a x t e dt − =

8.1 Representation of Periodic Sequence: the Discrete Fourier Series )m) 2元 JN ,k=0,1,2,…,W-1 Due to periodicity,we only need N complex exponentials for Fourier series representation of [n]: 网∑[e祭n =之[]e: The Fourier series representation of periodic sequence [n]
7 8.1 Representation of Periodic Sequence: the Discrete Fourier Series ◆Due to periodicity,we only need N complex exponentials 1 0 2 1 [ ] j N N k kn x n X k e N − = = 2 1 [ ] N k j kn x n X k e N = , 0,1,2, , 1 k N = − ( ) 2 j k N N m n e + 2 j n N k e = ( ) 2 2 j n j m k N n e e =
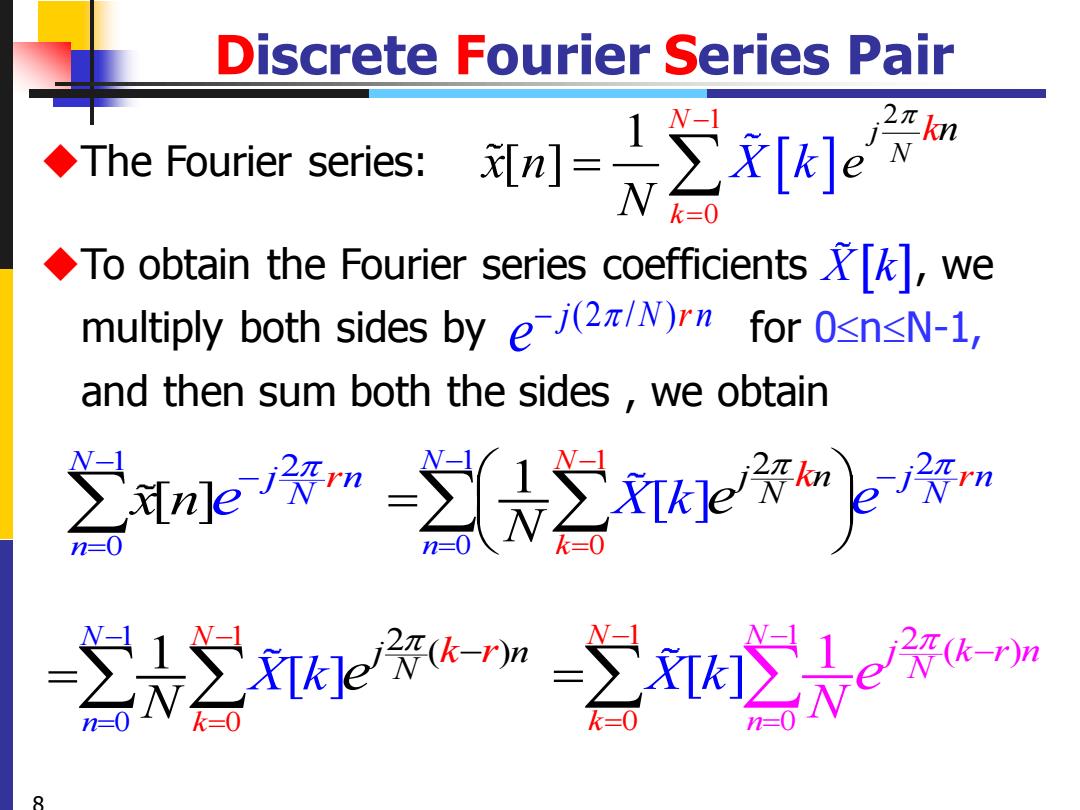
Discrete Fourier Series Pair 测=太2[] 27 kn ◆The Fourier series: N k二0 To obtain the Fourier series coefficients,we multiply both sides by e-j(2/N)rn f for0sn≤N-1, and then sum both the sides we obtain e废2ee n=0 -2p4"方k位动e
8 Discrete Fourier Series Pair ◆The Fourier series: 1 0 2 [ ] N n j n N r x n e − = − 0 1 1 0 2 ( ) [ ] 1 N n N k j k r n N N X k e − − = = − = ◆To obtain the Fourier series coefficients , we multiply both sides by for 0nN-1, and then sum both the sides , we obtain j n (2 / ) N r e − 1 1 0 0 2 ( ) [ ] 1 n N k N j n N k r N X k e − − = = − = 1 0 2 1 [ ] j N N k kn x n e X k N − = = 1 0 1 0 2 2 [ 1 ] N k N n j n j nr N N k X k N e e − − = = − = X k
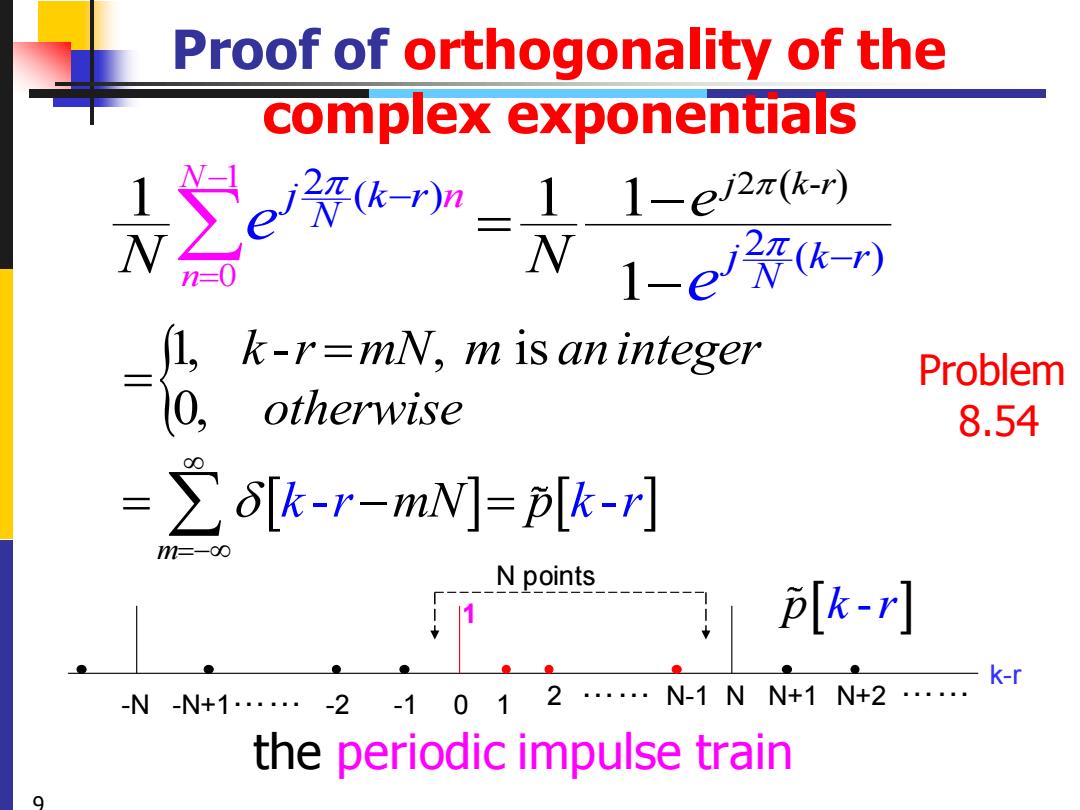
Proof of orthogonality of the complex exponentials ”=1 1-e2π(k-r) N 1-e() 1, k-r=mN,m is an integer Problem 0, otherwise 8.54 =∑6[k-r-mW]=p[k-r N points k-小 K-r -N-N+1·-2-101 2 N-1NN+1N+2. the periodic impulse train
9 - - m k r r k mN p =− = − = 1 2 ( ) 0 2 - ( ) 2 ( ) 1 1 1 1 N j k n r j k r N j k n r N e N N e e − = − − − = − Proof of orthogonality of the complex exponentials 1, - , is 0, k r mN m aninteger otherwise = = k-r 0 1 -N -N+1…… -2 -1 2 …… N-1 N N+1 N+2 …… N points 1 pk -r the periodic impulse train Problem 8.54