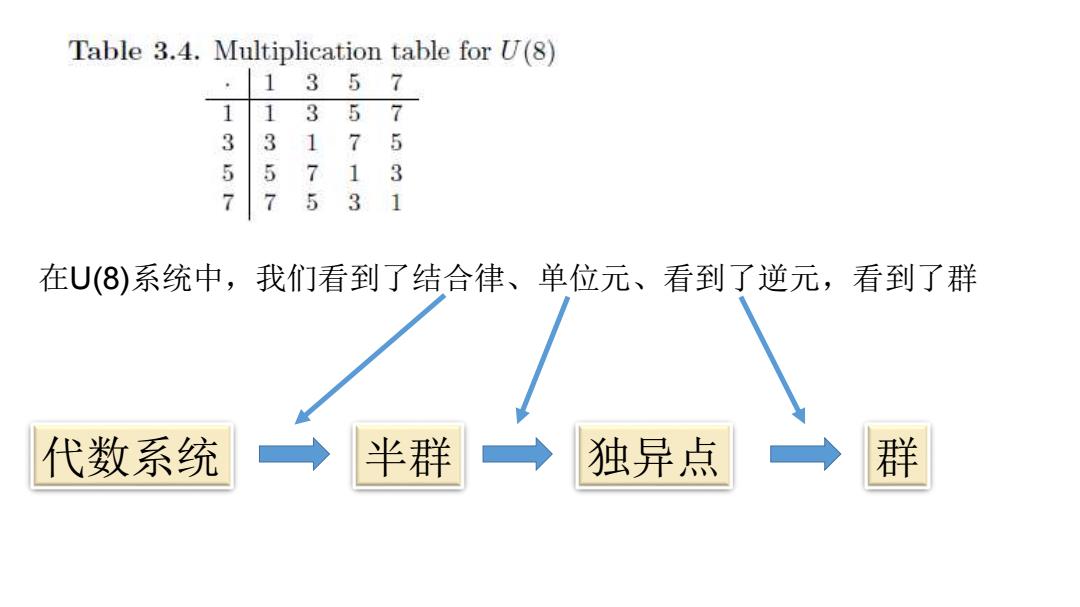
Table 3.4.Multiplication table for U(8) 3 1 5 7 1 1 3 57 3 3 175 5 5 7 1 3 7 7 5 3 1 在U(⑧)系统中,我们看到了结合律、单位元、看到了逆元,看到了群 代数系统 半群 独异点 群
在U(8)系统中,我们看到了结合律、单位元、看到了逆元,看到了群 代数系统 半群 独异点 群

Table 3.4.Multiplication table for U(8) 3 1 5 7 1 1 3 57 3 3 1 75 5 7 13 7 7 53 1 在U(8)系统中,我们看到了群,看到了这样的结构: 5 3
在U(8)系统中,我们看到了群,看到了这样的结构: 1 3 5 7
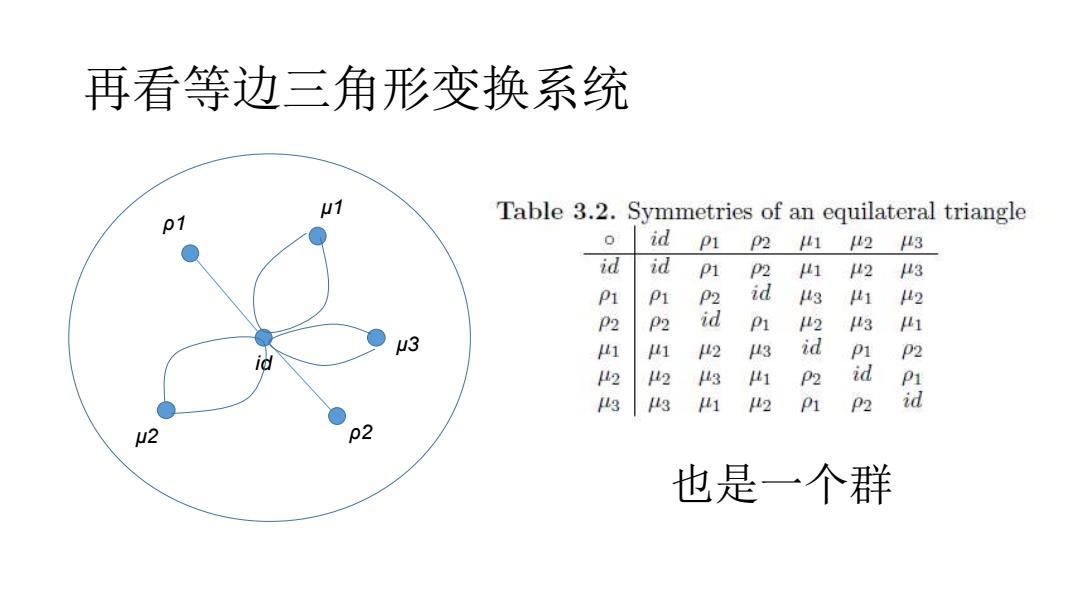
再看等边三角形变换系统 01 Table 3.2.Symmetries of an equilateral triangle id P1 P2 41 2 3 id P1 1 2 μ3 P1 id 3 1 2 P2 d P 2 3 1 U3 p 3 id P2 2 吗 a 3 a 2 也是一个群
再看等边三角形变换系统 id ρ1 ρ2 μ1 μ2 μ3 也是一个群

群-一种“公理化”定义的代数系统 The law of composition is associative.That is, 问题2:结合律 (aob)oc=ao(boc) 为什么会被放进 fora,b,c∈G. 群公理中? There exists an element e G,called the identity element,such that for any element a EG eo a aoe a. For each element a G,there exists an inverse element in G, denoted by a-1,such that aoa-1=a-loa=e
群 – 一种“公理化”定义的代数系统 问题2:结合律 为什么会被放进 群公理中?

结合律的作用 Proposition 3.19.Let G be a group.If a,bEG,then (ab)-1=b-1a-1. PROOF.Leta,b∈G.The abb-la-1 aca-1 aa-1=e.Similarly,b-la-lab =e.But by the previous proposition,inverses are unique;hence,(ab)-1=b-la-1. 口 问题3:如果这 里的运算实例化 为加法,这个计 算过程如何重写?
结合律的作用 问题3:如果这 里的运算实例化 为加法,这个计 算过程如何重写?