
定理4.4设A∈Cm"是Hermite.正定矩阵,则存在下 三角矩阵L∈Cx",使得A=LL,称为A的Cholesky分解。 以n=3为例 [a1a2 937 d 2 a23 121 l22 as a32 13112 1 k 42 11 =42141h2+1zf 1☑21l1+12l2 g141g1+122h+h2+ha月 This documentis prduced byria version ofPrnhVisit www.prinashcfor more informion
This document is produced by trial version of Print2Flash. Visit www.print2flash.com for more information

Ax=b→L(UX)=b三 Ly=b Ux=y 例.试用Doolittle分解求解方程组 2 -6 10 13 -19 19 -6 -3 -6Lx3] -30 2 5 -6 「1 0 04 42 413 3 -19 0 V22 2423 -6 -3 6 12 133」 This documentis produced bytril versinofPrVisit www.prinashmformore infomio
This document is produced by trial version of Print2Flash. Visit www.print2flash.com for more information
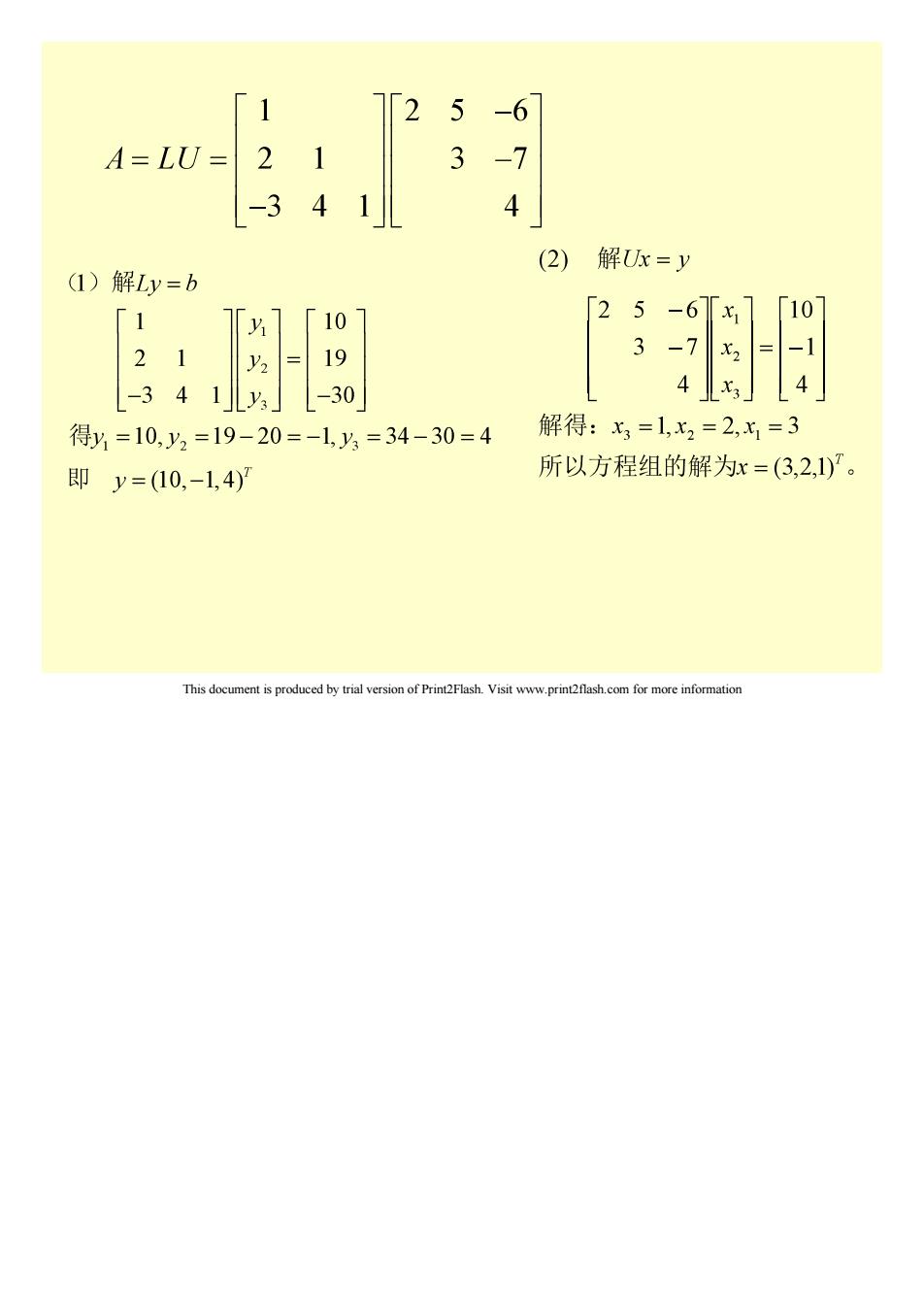
7「2 -6 A=LU= 21 3-7 -341 4 (2)解Ux=y (1)解Ly=b 1 7y1「10 21 y2 19 1-341y」-30」 得y=10,y2=19-20=-1,y=34-30=4 解得:x3=1,x2=2,x=3 即y=10,-1,4) 所以方程组的解为x=(3,2,1)。 This documentis prduced byria version ofPrnhVisit www.prinashcfor more informion
This document is produced by trial version of Print2Flash. Visit www.print2flash.com for more information
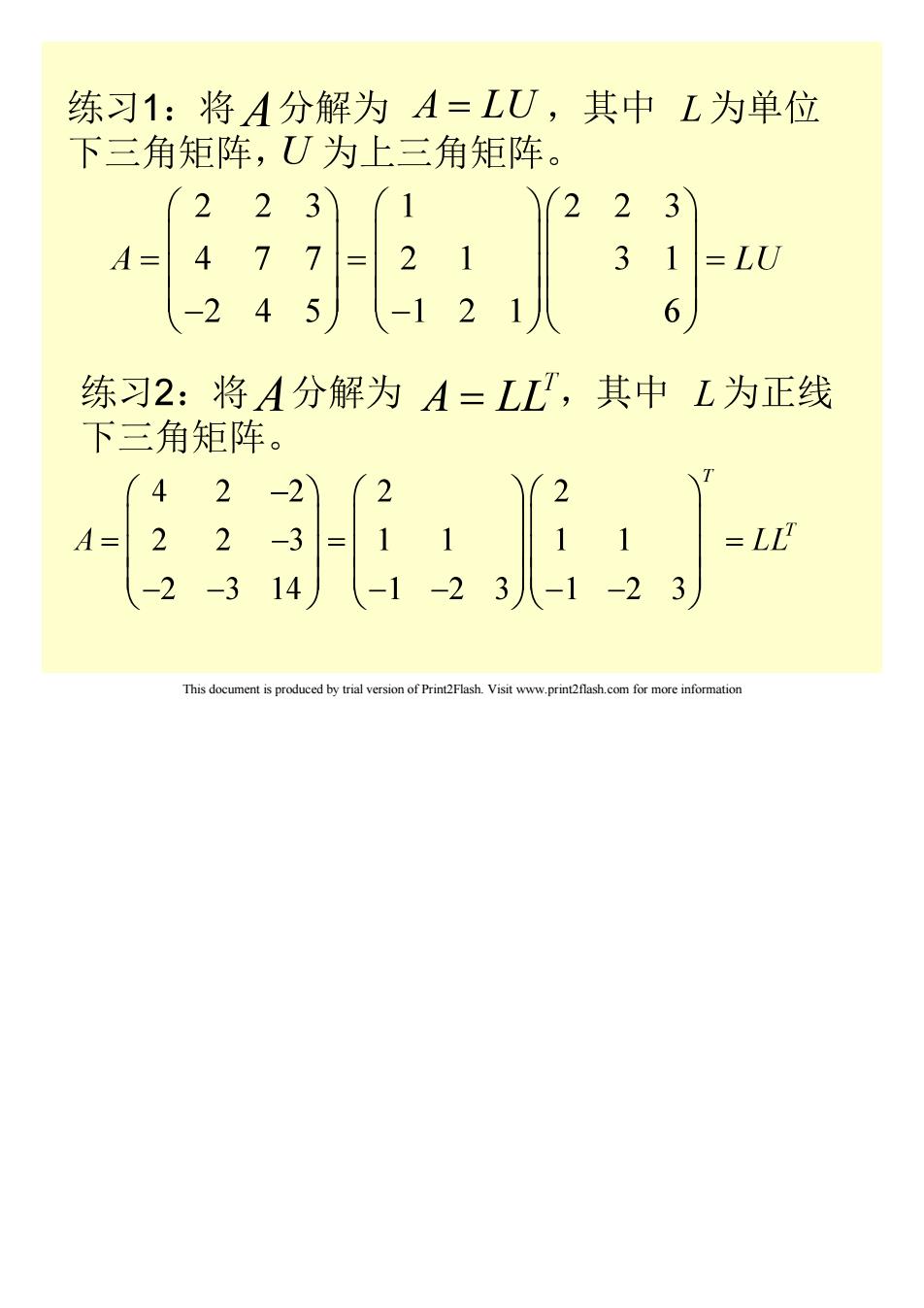
练习1:将A分解为A=LU,其中L为单位 下三角矩阵,U为上三角矩阵: 22 23 4= =LU 6 练习2:将A分解为A=L,其中L为正线 下三角矩阵。 This document is produced by trial version of Print2Flash Visit www.print2flash.com for more informatio
This document is produced by trial version of Print2Flash. Visit www.print2flash.com for more information

4.2矩阵的QR分解 定义:设A∈Cx”,如果存在n阶酉矩阵Q 和n阶上三角矩阵R,使得A=OR 则称之为A的QR分解或酉-三角分解。当 A∈Rx”时,称为A的正交三角分解。 定理4.5任意A∈Cmx”都可以作QR分解。 定理4.6设A∈C”,则A可唯一分解为 A=OR 其中Q是n阶酉矩阵,R∈C"是具有正对角元的 上三角矩阵
This document is produced by trial version of Print2Flash. Visit www.print2flash.com for more information