
§4.2 The Frequency Response H(ei),in general,is a complex function of o with a period2π It can be expressed in terms of its real and imaginary parts H(e)=Hre(ej)+j Him(ei) or,in terms of its magnitude and phase, H(eio)=H(eio)川e(o) where θ(o)=argH(ejo)
§4.2 The Frequency Response • H(ejω), in general, is a complex function of ω with a period 2π • It can be expressed in terms of its real and imaginary parts H(ejω)= Hre(ejω) +j Him(ejω) or, in terms of its magnitude and phase, H(ejω)=|H(ejω)| eθ(ω) where θ(ω)=argH(ejω)

§4.2 The Frequency Response The function H(ei)is called the magnitude response and the function 0(@)is called the phase response of the LTI discrete-time system Design specifications for the LTI discrete-time system,in many applications,are given in terms of the magnitude response or the phase response or both
§4.2 The Frequency Response • The function | H(ejω) | is called the magnitude response and the function θ(ω) is called the phase response of the LTI discrete-time system • Design specifications for the LTI discrete-time system, in many applications, are given in terms of the magnitude response or the phase response or both
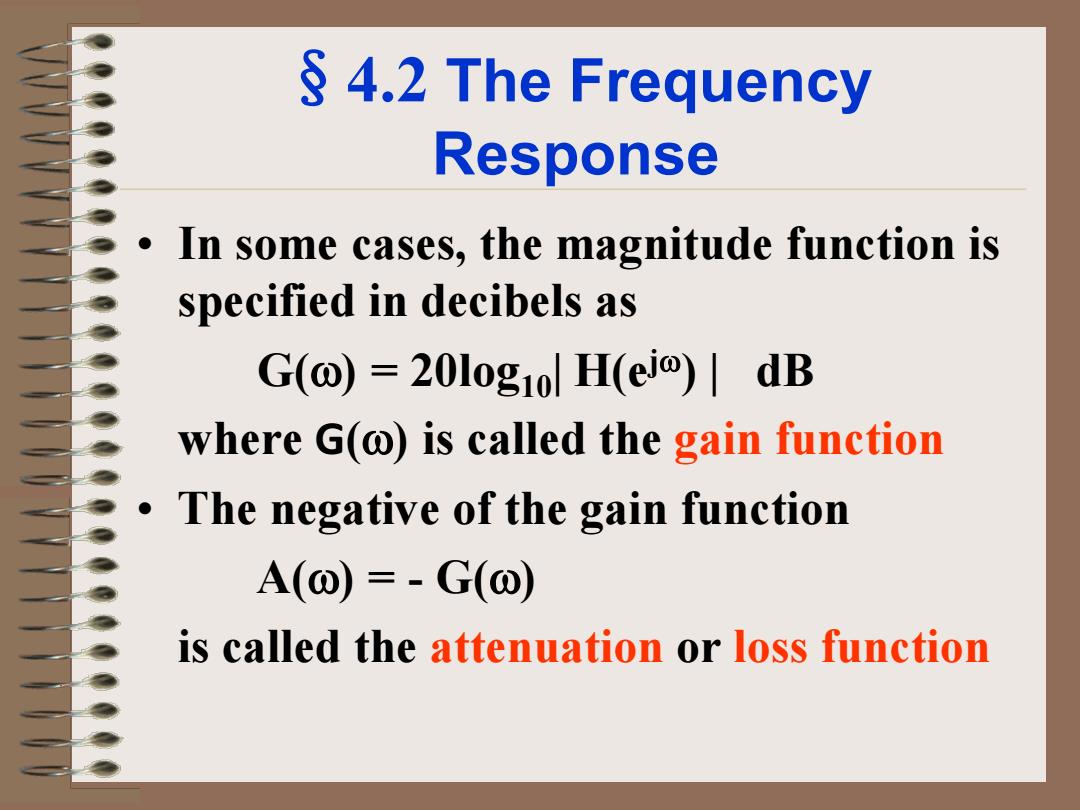
§4.2 The Frequency Response n some cases,the magnitude function is specified in decibels as G(@)=20l0g10 H(ei)dB where G(@)is called the gain function The negative of the gain function A(O)=-G(0) is called the attenuation or loss function
§4.2 The Frequency Response • In some cases, the magnitude function is specified in decibels as G(ω) = 20log10| H(ejω) | dB where G(ω) is called the gain function • The negative of the gain function A(ω) = - G(ω) is called the attenuation or loss function
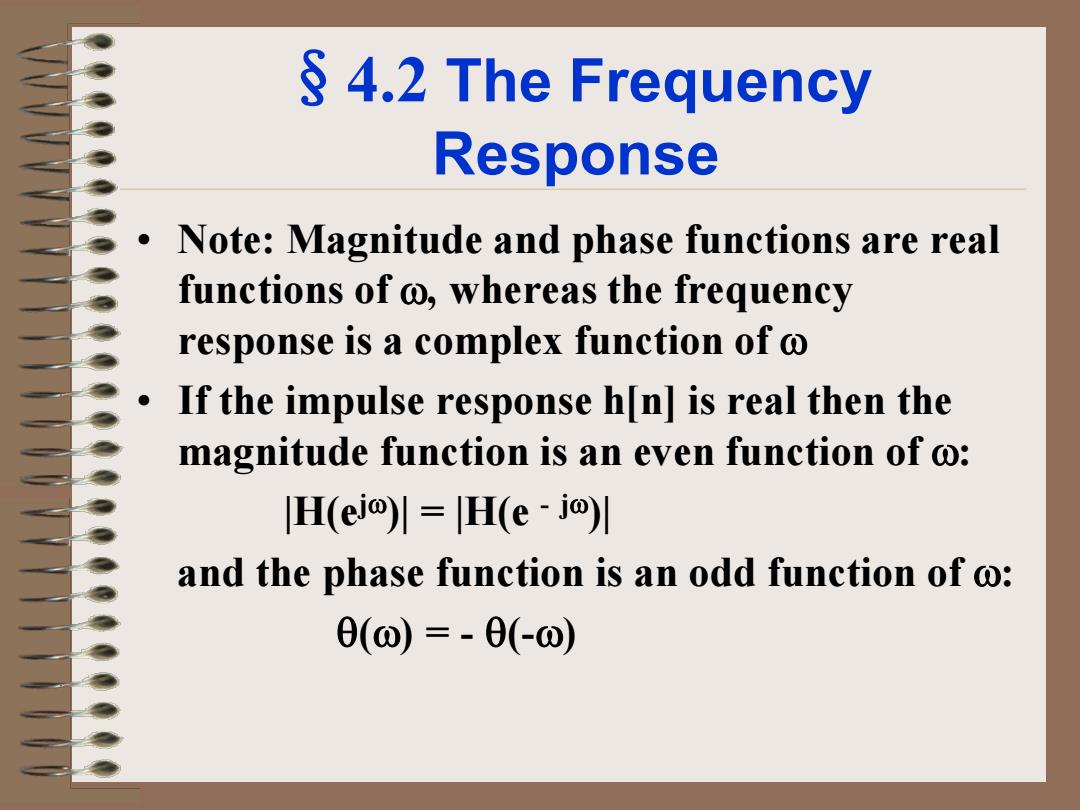
§4.2 The Frequency Response Note:Magnitude and phase functions are real functions of o,whereas the frequency response is a complex function of o If the impulse response h[n]is real then the magnitude function is an even function of o: H(eio)川=IH(e-jo)川 and the phase function is an odd function of o: 0(@)=-0(-0)
§4.2 The Frequency Response • Note: Magnitude and phase functions are real functions of ω, whereas the frequency response is a complex function of ω • If the impulse response h[n] is real then the magnitude function is an even function of ω: |H(ejω)| = |H(e - jω)| and the phase function is an odd function of ω: θ(ω) = - θ(-ω)