
=n29r-21=ew29 (4.17) We decide D,if ep(2,≥4 (4.18) e(y)is log-likelihood ratio e(y)=In L(y) (4.19) We decide D if 2bo2In4 (4.20) UESTC 11
11 UESTC 2 2 2 2 2 ( ) ( ) 2 ( ) exp[ ] exp( ) (4.17) 2 2 y b y b yb L y + − = − = 2 2 exp( ) 4 (4.18) yb 2 2 ln 4 (4.20) yb ( ) ln ( ) (4.19) y L y = (y) is log-likelihood ratio We decide D1 if We decide D1 , if

呀 D1_2 y In4 (4.21) 2b Ifπo=兀1=0.5,then In=0 Then,the ML criterion decision D > y 0 < UESTC Do 12
12 UESTC 1 0 2 ln 4 (4.21) 2 D D y b If , then 0.5 0 = 1 = ln 0 ML = Then, the ML criterion decision 1 0 0 D D y

36 p(yIHo) p(ylH) -b b y Figure 4.1.Probability density functions for Example 2.1 UESTC 13
13 UESTC
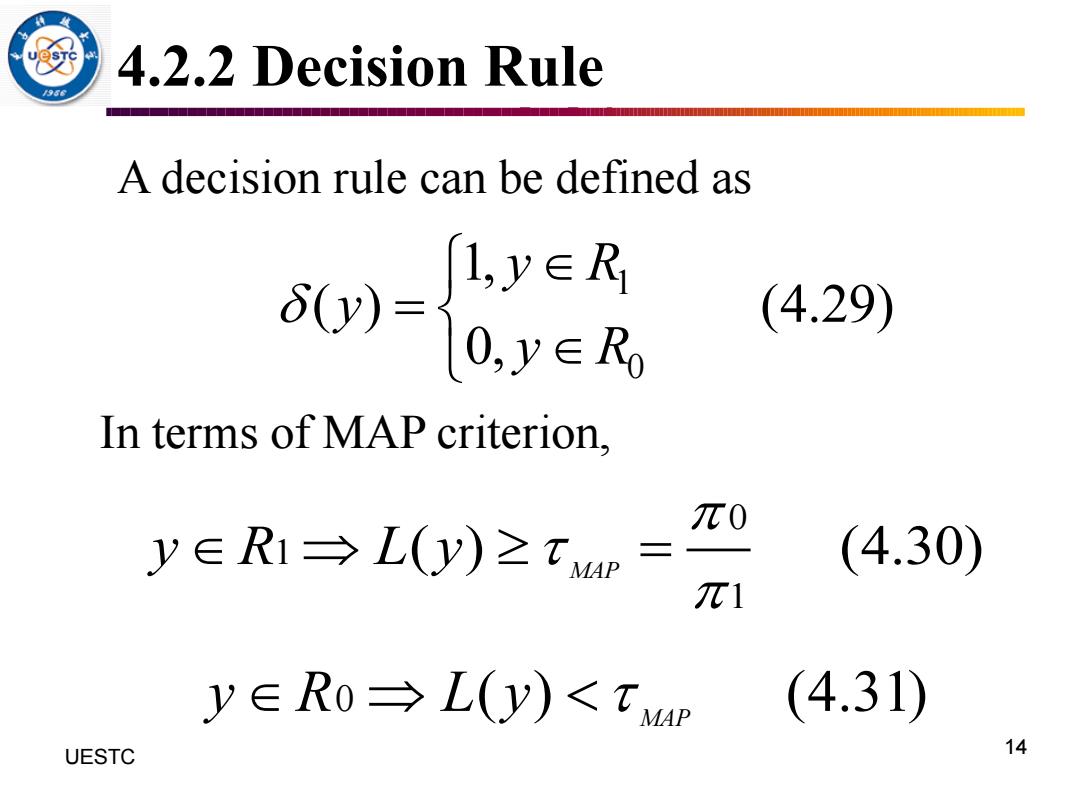
4.2.2 Decision Rule A decision rule can be defined as -及 1,y∈R (4.29) In terms of MaP criterion, 元0 y∈R1→L(y)≥TMP= (4.30) π1 y∈RO→L(y)<tMP (4.31) UESTC 14
14 UESTC 1 0 1, ( ) (4.29) 0, y R y y R = 0 1 1 ( ) (4.30) MAP y R L y = 0 ( ) (4.31) MAP y R L y A decision rule can be defined as In terms of MAP criterion, 4.2.2 Decision Rule

It follows that the MaP decision rule is 1,yeL(y)≥ 0 δMar(y)= 兀1 (4.32) 0,Vvei()< 元0 元1 UESTC 15
15 UESTC It follows that the MAP decision rule is ( ) ( ) ( ) 0 1 0 1 1, (4.32) 0, MAP y L y y y L y =