CH4 LMS Algorithm Remarkably simple A simple one line updating algorithm Not more than roughly 2N Multiply-Add per time update May be derived from the RLS algorithm Remarkably complex Convergence/stability analysis
CH4 LMS Algorithm Remarkably simple A simple one line updating algorithm Not more than roughly 2N Multiply-Add per time update May be derived from the RLS algorithm Remarkably complex Convergence/stability analysis

Content o Steepest-descent algorithm o LMS algorithm o LMS for time-varying set-ups o LMS variants o Normalized LMS (NLMS) o LMS versus RLS convergence 2020-01-18 2
2020-01-18 2 Content Steepest-descent algorithm LMS algorithm LMS for time-varying set-ups LMS variants Normalized LMS (NLMS) LMS versus RLS convergence
S1.Steepest-descent algorithm o Update formula o Convergence condition o Transient behavion ●The learning curve WC 2020-01-18
2020-01-18 3 S1. Steepest-descent algorithm Update formula Convergence condition Transient behavior The learning curve

Solving the Wiener-Hopf equations o Solving the Wiener-Hopf equations .Direct method (e.g.,Gauss elimination) Iterative method:Avoid matrix inverse and find optimal solution recursively maw0生2e Jw-w(n) 2020-01-18 4
2020-01-18 4 Solving the Wiener-Hopf equations Solving the Wiener-Hopf equations Direct method (e.g., Gauss elimination) Iterative method: Avoid matrix inverse and find optimal solution recursively ( ) ( ) ( 1) ( ) 2 MSE n J n n w w w w w w
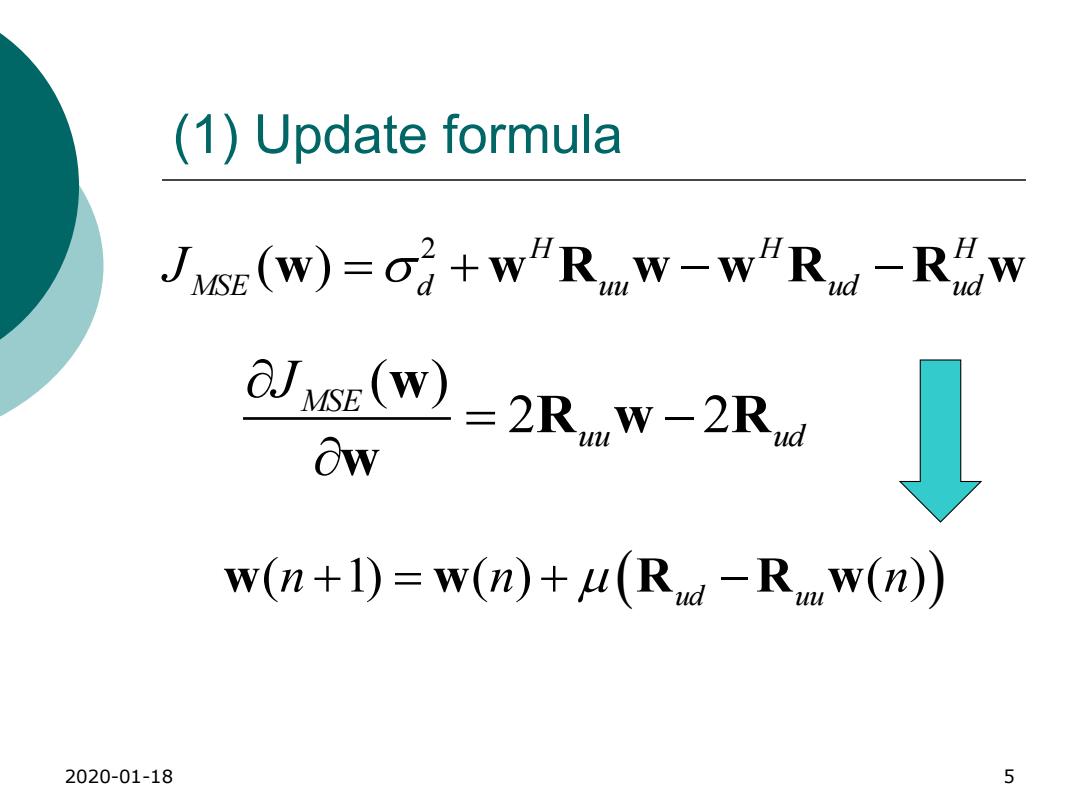
(1)Update formula JuSE(w)=od+w"RW-wRd-Rw Jssw)=2Rmw-2Rd Ow w(n+1)=w(n)+u(Rd-RmW(n)) 2020-01-18 5
2020-01-18 5 (1) Update formula ( ) 2 2 MSE uu ud J w R w R w 2 ( ) H H H MSE d uu ud ud J w w R w w R R w w w R R w ( 1) ( ) ( ) n n n ud uu