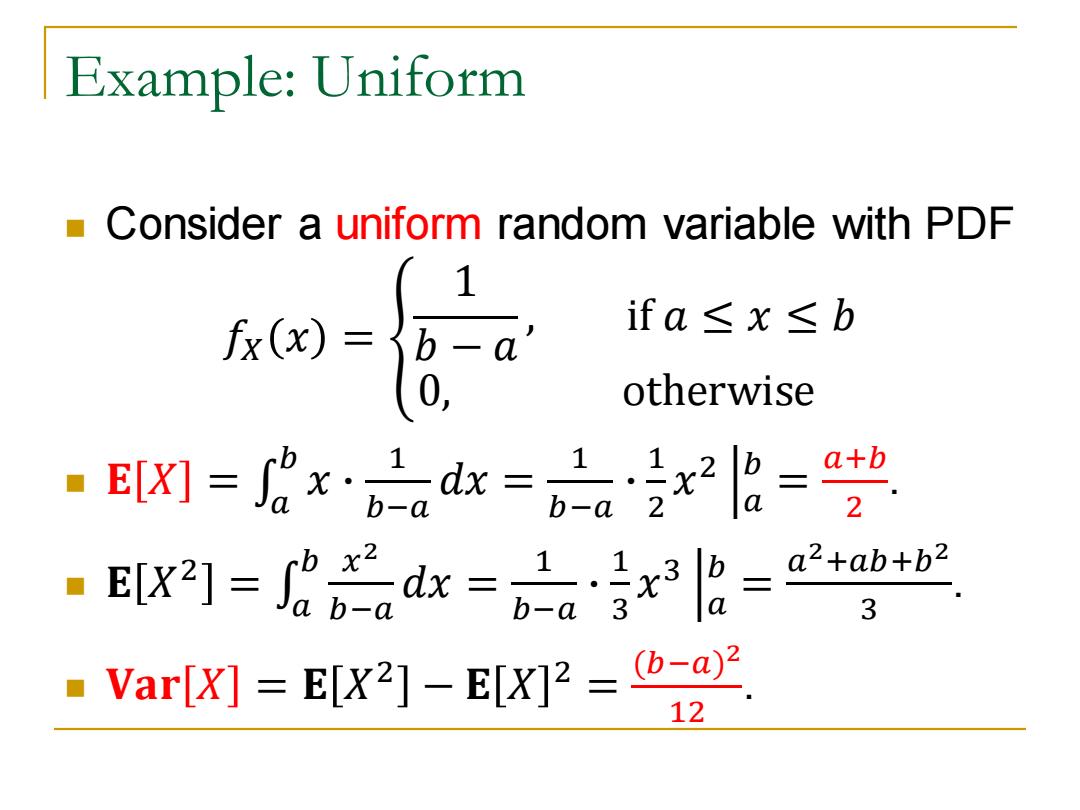
Example:Uniform Consider a uniform random variable with PDF 1 (x) ifa≤x≤b otherwise E[☒=必xa=ga2l收= 2 EIX4]=dx a2+ab+b2 3 Var[X]E[X2]-E[X]2 =(b-a)2 12
Example: Uniform ◼ Consider a uniform random variable with PDF 𝑓𝑋 𝑥 = ቐ 1 𝑏 − 𝑎 , if 𝑎 ≤ 𝑥 ≤ 𝑏 0, otherwise �� = �� �� ◼ 𝑏 𝑥 ∙ 1 𝑏−𝑎 𝑑𝑥 = 1 𝑏−𝑎 ∙ 1 2 𝑥 2 ቚ 𝑏 𝑎 = 𝑎+𝑏 2 . ◼ 𝐄 𝑋 �� = 2 𝑏 𝑥 2 𝑏−𝑎 𝑑𝑥 = 1 𝑏−𝑎 ∙ 1 3 𝑥 3 ቚ 𝑏 𝑎 = 𝑎 2+𝑎𝑏+𝑏 2 3 . ◼ 𝐕𝐚𝐫 𝑋 = 𝐄 𝑋 2 − 𝐄 𝑋 2 = (𝑏−𝑎) 2 12
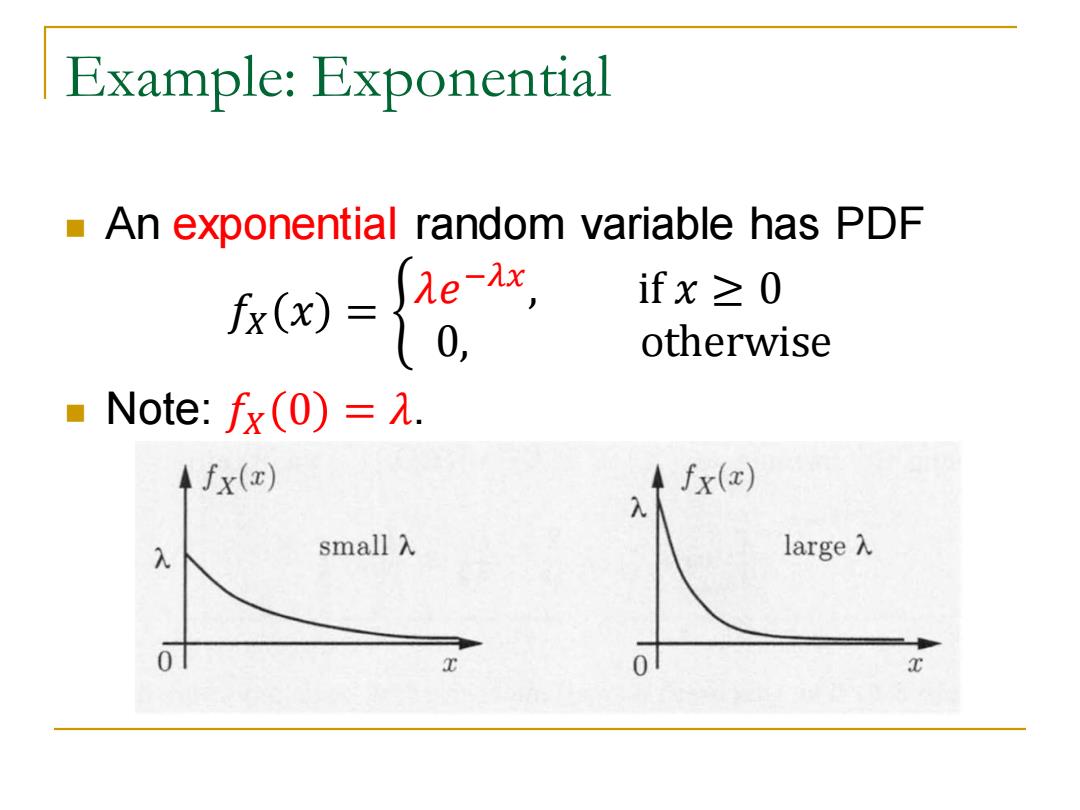
Example:Exponential An exponential random variable has PDF -你 ifx≥0 otherwise ■Note:fx(0)=λ. A fx(x) fx(x) 入 入 small入 large入 0 0 x
Example: Exponential ◼ An exponential random variable has PDF 𝑓𝑋 𝑥 = ቊ 𝜆𝑒 −𝜆𝑥 , if 𝑥 ≥ 0 0, otherwise ◼ Note: 𝑓𝑋 0 = 𝜆
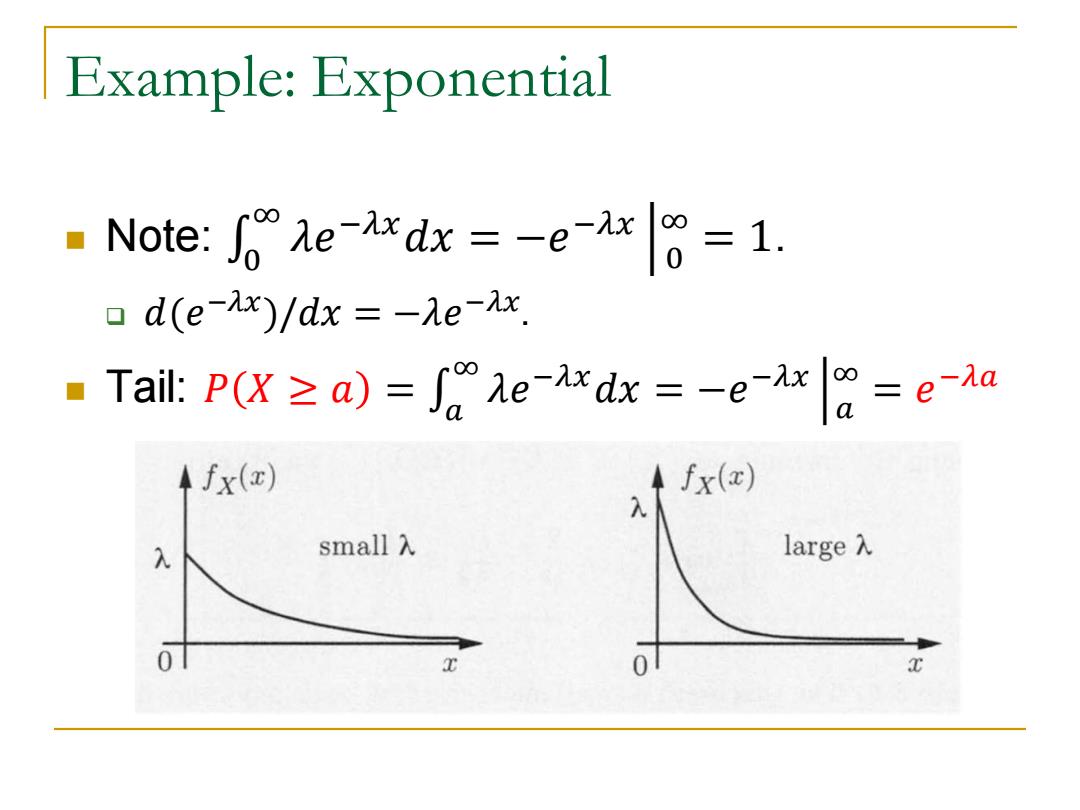
Example:Exponential Note:fo e-ixdx=-e-Ax=1. 口d(e-x)/dx=-λe-x. Tail:P(X z a)S e-xdx =-e-ix a e-la Afx(x) Afx(a) 入 small入 large入 0 x 0 x
Example: Exponential ◼ Note: 0 ∞ 𝜆𝑒 −𝜆𝑥𝑑𝑥 = −𝑒 −𝜆𝑥 ቚ ∞ 0 = 1. ❑ 𝑑(𝑒 −𝜆𝑥)/𝑑𝑥 = −𝜆𝑒 −𝜆𝑥 . �� = �� ≤ �� �� :Tail◼ ∞ 𝜆𝑒 −𝜆𝑥𝑑𝑥 = −𝑒 −𝜆𝑥 ቚ ∞ 𝑎 = 𝑒 −𝜆𝑎
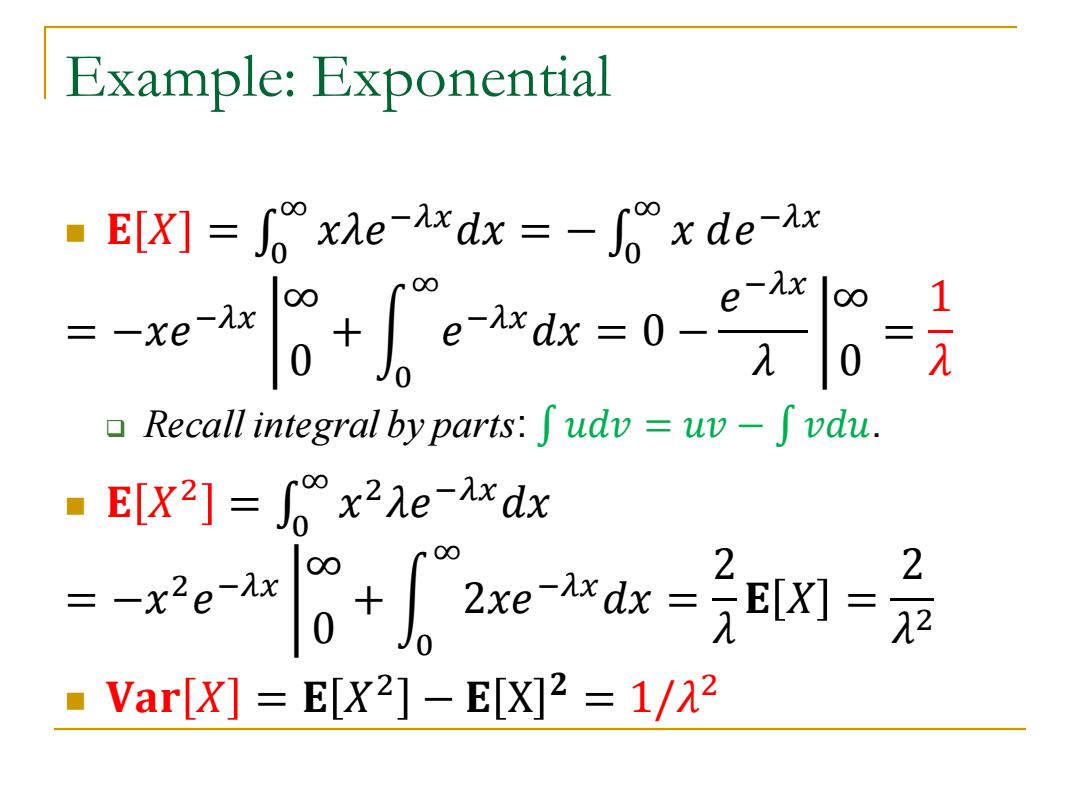
Example:Exponential E[X]=fo xe-ixdx=-fx de-ix e-Ax 1 =-xe-1x 0 ▣Recall integral by parts:∫udv=uw-∫vdu. ■E[X2]=Jx2ne-rdx ●义 00 2 =-x2e-Ax 2xe-xdx=E[X刈 2 22 ·Var[X]=E[X2]-E[X]2=1/2
Example: Exponential 0 = �� �� ◼ ∞ 𝑥𝜆𝑒 0 − = �𝑑𝑥𝜆�− ∞ 𝑥 𝑑𝑒 −𝜆𝑥 = −𝑥𝑒 −𝜆𝑥 ቤ ∞ 0 + න 0 ∞ 𝑒 −𝜆𝑥𝑑𝑥 = 0 − 𝑒 −𝜆𝑥 𝜆 ቤ ∞ 0 = 1 𝜆 ❑ Recall integral by parts: − �𝑢� = �𝑑𝑢� .�𝑑𝑣� ◼ 𝐄 𝑋 2 = 0 ∞ 𝑥 2𝜆𝑒 −𝜆𝑥𝑑𝑥 = −𝑥 2 𝑒 −𝜆𝑥 ቤ ∞ 0 + න 0 ∞ 2𝑥𝑒−𝜆𝑥𝑑𝑥 = 2 𝜆 𝐄 𝑋 = 2 𝜆 2 ◼ 𝐕𝐚𝐫 𝑋 = 𝐄 𝑋 2 − 𝐄 X 𝟐 = 1/𝜆 2

Example Time X of a meteorite first lands in Sahara An exponential r.v.with mean of 10 days. Since E[X]1/1,we have 1=1/10. Question:What's the probability of it first lands in 6am-6pm of the first day? ▣P(任≤X≤到=P(X≥)-P(x≥) 1 3 =e40-e40=0.0476
Example ◼ Time 𝑋 of a meteorite first lands in Sahara. ◼ An exponential r.v. with mean of 10 days. ◼ Since 𝐄 𝑋 = 1/𝜆, we have 𝜆 = 1Τ10. ◼ Question: What’s the probability of it first lands in 6am – 6pm of the first day? ◼ 𝑃 1 4 ≤ 𝑋 ≤ 3 4 = 𝑃 𝑋 ≥ 1 4 − 𝑃 𝑋 ≥ 3 4 = 𝑒 − 1 40 − 𝑒 − 3 40 = 0.0476