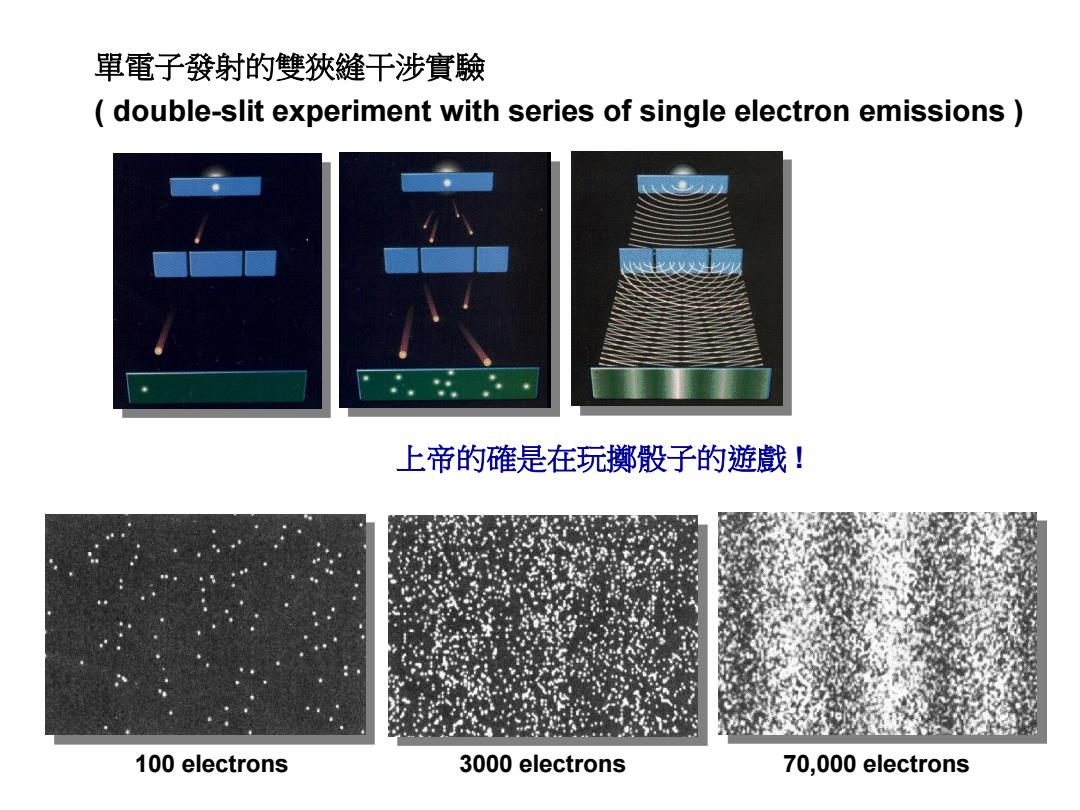
單電子發射的雙狹縫干涉實驗 double-slit experiment with series of single electron emissions 上帝的確是在玩擲骰子的遊戲! 100 electrons 3000 electrons 70,000 electrons
單電子發射的雙狹縫干涉實驗 ( double-slit experiment with series of single electron emissions ) 上帝的確是在玩擲骰子的遊戲 ! 100 electrons 3000 electrons 70,000 electrons

A PICCAID E HENPOT'P.SHRENFEST Id.HERZEN TA.DE DONDER ESCHRCOINGER EVERSCIAFELT W.PAUU W.HISENBERG BH.FOWLER L.BRILOUIN P.DESYE M.KNLOSEN W.L.BRAGG HA.KRAMERS PAM DIRAC A.H.COMPTON L.d BROGLIE N BOHR (29 attendees, M PLANCK Mme CURIE P.LANGEVIN Ch.E.CUYE C.T..WISON OW.RICHARDION 17 Nobel prize winners) 1927 Fifth Solvay International Conference on Electrons and Photons. Einstein "God does not play dice". Bohr replied "Einstein,stop telling God what to do
1927 Fifth Solvay International Conference on Electrons and Photons. Einstein : "God does not play dice". Bohr replied : "Einstein, stop telling God what to do". (29 attendees, 17 Nobel prize winners)

Bohr and Heisenberg COPENHAGEN
Bohr and Heisenberg

let the particle wave be represented by P(r,t) Yr,t):wave function(波函數),r:position The probability that the particle will be found in volume dV: P(r,t dv=2dv P(r,t )=2:probability density 2dV=1 normalization ·量子力學主要在幹什麼? →尋找或計算一個物理系統(單粒子或多粒子系統)的波函數
• 量子力學主要在幹什麼 ? 尋找或計算一個物理系統 ( 單粒子或多粒子系統 ) 的波函數 • let the particle wave be represented by Y( r, t ) Y( r,t ) : wave function ( 波函數 ) , r : position • The probability that the particle will be found in volume dV : P( r,t ) dV = Y 2 dV , P( r,t ) = Y 2 : probability density Y 2 dV = 1 ( normalization ) –

·例如:一個在一維空間中以等速率運動的粒子: 其波函數可以Ψ(xt)=Asin(kx-ot),k=2/入,o=2πU代表 (1)same amplitude A at all points (2)well-defined入=2元/k and u=wl2πat all points (3)travels in“+x”direction,v=λ·u=o/k →this particle: (1)has a well-defined p=h/ (2)P(x,t dx =2 dx A2dx at any point Particle can be found with equal probability at any point (因為粒子以等速率自∞移動至+∞處,因此在各點出現及停留的 時間都一樣)
• 例如 : 一個在一維空間中以等速率運動的粒子 : 其波函數可以 Y( x,t ) = A sin( kx – wt ) , k = 2p/l , w = 2p u 代表 (1) same amplitude A at all points (2) well-defined l = 2p/k and u = w/2p at all points (3) travels in “+x” direction , v = l • u = w / k this particle : (1) has a well-defined p= h/l (2) P( x,t ) dx = Y 2 dx = A2dx at any point Particle can be found with equal probability at any point ( 因為粒子以等速率自– 移動至 + 處 , 因此在各點出現及停留的 時間都一樣 )