
版权所有:华东理工大学物理化学教研室 6 In Path 2, the initial and final states are the same but in which the expansion is not adiabatic. In this path an energy q' enters the system as heat and the work w' is not the same as w . T h e w o r k a n d the heat are path functions. 1). Exact and inexact differential ∫ = f i q q ,path d 3.1 State functions
版权所有:华东理工大学物理化学教研室 6 In Path 2, the initial and final states are the same but in which the expansion is not adiabatic. In this path an energy q' enters the system as heat and the work w' is not the same as w . T h e w o r k a n d the heat are path functions. 1). Exact and inexact differential ∫ = f i q q ,path d 3.1 State functions
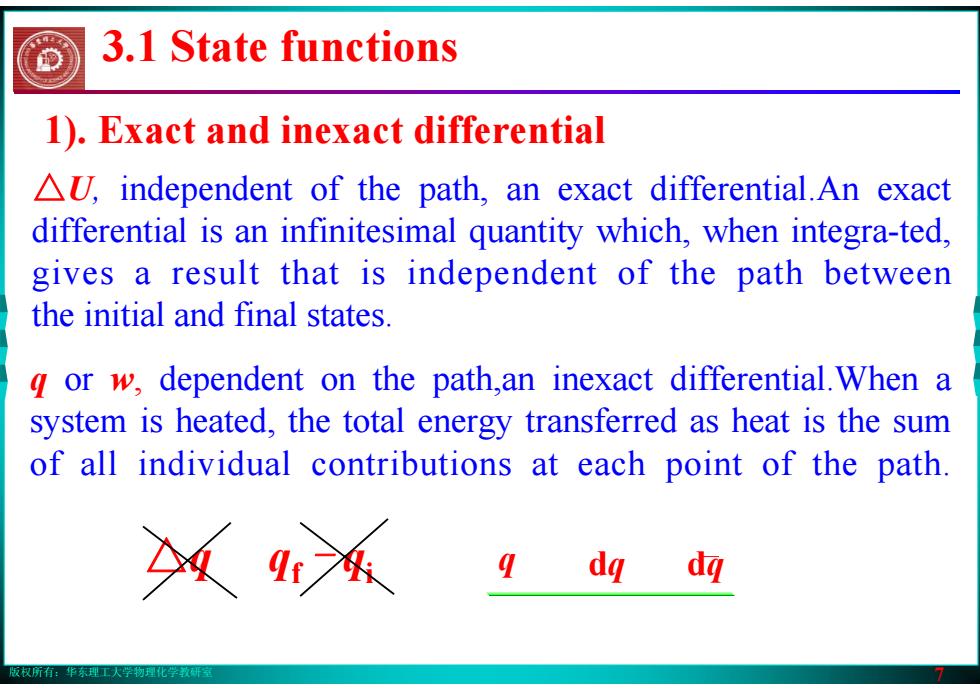
版权所有:华东理工大学物理化学教研室 7 △U, independent of the path, an exact differential.An exact differential is an infinitesimal quantity which, when integra-ted, gives a result that is independent of the path between the initial and final states. q or w, dependent on the path,an inexact differential.When a system is heated, the total energy transferred as heat is the sum of all individual contributions at each point of the path. 1). Exact and inexact differential △q qf - qi q dq dq 3.1 State functions
版权所有:华东理工大学物理化学教研室 7 △U, independent of the path, an exact differential.An exact differential is an infinitesimal quantity which, when integra-ted, gives a result that is independent of the path between the initial and final states. q or w, dependent on the path,an inexact differential.When a system is heated, the total energy transferred as heat is the sum of all individual contributions at each point of the path. 1). Exact and inexact differential △q qf - qi q dq dq 3.1 State functions
版权所有:华东理工大学物理化学教研室 8 Consider a perfect gas inside a cylinder fitted with a piston. Let the initial state be T, Vi and the final state be T, Vf . The change of state can be brought about in many ways, of which the two simplest are following: Path 1, in which there is free expansion against zero external pressure; Path 2, in which there is reversible, isothermal expansion. Calculate w , q , and Δ U f o r e a c h process. Example -Calculating work, heat, and internal energy
版权所有:华东理工大学物理化学教研室 8 Consider a perfect gas inside a cylinder fitted with a piston. Let the initial state be T, Vi and the final state be T, Vf . The change of state can be brought about in many ways, of which the two simplest are following: Path 1, in which there is free expansion against zero external pressure; Path 2, in which there is reversible, isothermal expansion. Calculate w , q , and Δ U f o r e a c h process. Example -Calculating work, heat, and internal energy

版权所有:华东理工大学物理化学教研室 9 Method: To find a starting point for a calculation in thermodynamics, it is often a good idea to go back to first principles, and to look for a way of expressing the quantity we are asked to calculate in terms of other quantities that are easier to calculate. Because the internal energy of a perfect gas arises only from the kinetic energy of its molecules, it is independent of volume; therefore, for any i s o t h e r m a l c h a n g e , i n general ΔU = q+w. Example -Calculating work, heat, and internal energy
版权所有:华东理工大学物理化学教研室 9 Method: To find a starting point for a calculation in thermodynamics, it is often a good idea to go back to first principles, and to look for a way of expressing the quantity we are asked to calculate in terms of other quantities that are easier to calculate. Because the internal energy of a perfect gas arises only from the kinetic energy of its molecules, it is independent of volume; therefore, for any i s o t h e r m a l c h a n g e , i n general ΔU = q+w. Example -Calculating work, heat, and internal energy

版权所有:华东理工大学物理化学教研室 10 Answer: Since ΔU = 0 for both paths and ΔU = q+w. The work of free expansion is zero, in Path 1, w = 0 and q = 0; for Path 2, the work is given by so w = - nRT ln (Vf /Vi), and q = nRT ln (Vf /Vi). i f ln f d i V V nRT V V w nRT V V = − = − ∫ Example -Calculating work, heat, and internal energy
版权所有:华东理工大学物理化学教研室 10 Answer: Since ΔU = 0 for both paths and ΔU = q+w. The work of free expansion is zero, in Path 1, w = 0 and q = 0; for Path 2, the work is given by so w = - nRT ln (Vf /Vi), and q = nRT ln (Vf /Vi). i f ln f d i V V nRT V V w nRT V V = − = − ∫ Example -Calculating work, heat, and internal energy