
Chapter 7 Economic Growth Capital and Population
⚫Chapter 7 Economic Growth : ⚫Capital and Population

The lessons ofgrowth theory .can make a positive difference in the lives of hundreds of millions of people. These lessons help us understand why poor countries are poor design policies that can help them grow learn how our own growth rate is affected by shocks and our government's policies
The lessons of growth theory .can make a positive difference in the lives of hundreds of millions of people. These lessons help us • understand why poor countries are poor • design policies that can help them grow • learn how our own growth rate is affected by shocks and our government’s policies

Learning objectives Learn the closed economy Solow model See how a country's standard of living depends on its saving and population growth rates 。Learn how to use the“Golden Rule'” to find the optimal savings rate and capital stock
Learning objectives • Learn the closed economy Solow model • See how a country’s standard of living depends on its saving and population growth rates • Learn how to use the “Golden Rule” to find the optimal savings rate and capital stock

Content 1.Capital Accumulation 1.1 Production Function in Per Worker Term 1.2 Capital Growth and Steady State 1.3 Moving toward the steady state .1.4 Impact of Saving Rate 2.Golden Rule Level of Capital 2.1 Golden Rule Capital Stock 2.2 The transition to the Golden Rule Steady State ·3.Population Growth ·4.Chapter Summary
Content • 1. Capital Accumulation • 1.1 Production Function in Per Worker Term • 1.2 Capital Growth and Steady State • 1.3 Moving toward the steady state • 1.4 Impact of Saving Rate • 2. Golden Rule Level of Capital • 2.1 Golden Rule Capital Stock • 2.2 The transition to the Golden Rule Steady State • 3. Population Growth • 4. Chapter Summary
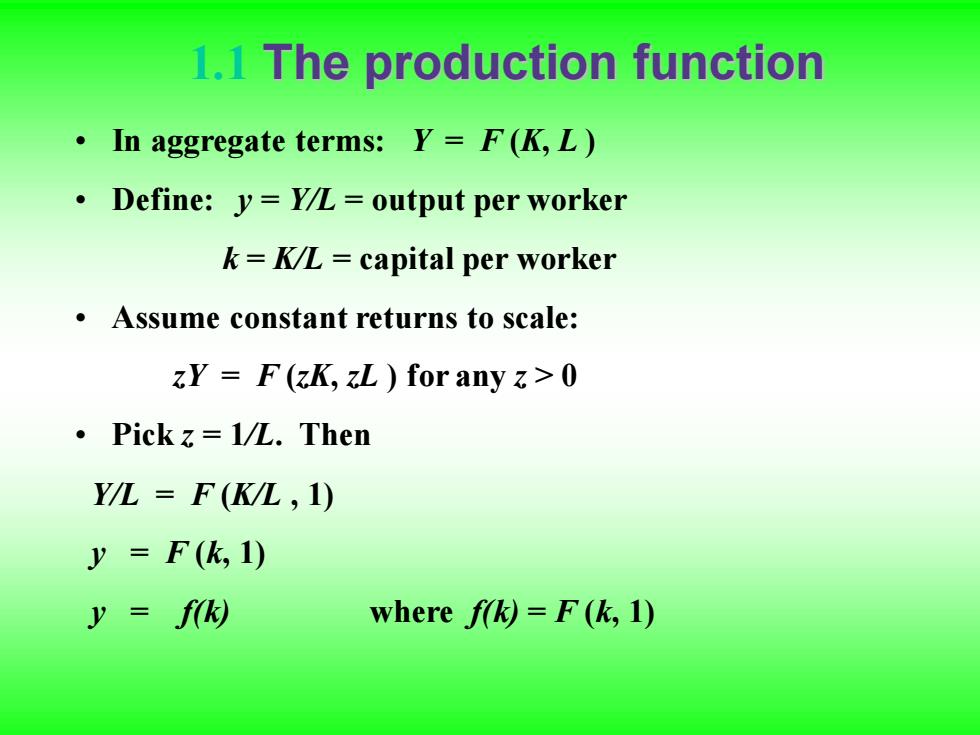
1.1 The production function In aggregate terms:Y=F(K,L) Define:y=YL=output per worker k=KL capital per worker Assume constant returns to scale: Y=F(zK,zL)for any >0 ·Pick=1L.Then Y/L F(K/L,1) y=F(k,1) y=f(k) where fk)=F(k,1)
1.1 The production function • In aggregate terms: Y = F (K, L ) • Define: y = Y/L = output per worker k = K/L = capital per worker • Assume constant returns to scale: zY = F (zK, zL ) for any z > 0 • Pick z = 1/L. Then Y/L = F (K/L , 1) y = F (k, 1) y = f(k) where f(k) = F (k, 1)