
Boundary Layer Similarity Parameters The boundary layer equations(velocity,mass, energy continuity)represent low speed,forced convection flow. Advection terms on the left side and diffusion terms on the right side of each equation, such as: Advection TT Diffusion W- Ox dy 0y2 Non-dimensionalize the equations by setting ond y where L is characteristic length of the surface and v-y where V is the freestream velocity (U) and T-T and P=p/pv2 T-T
Boundary Layer Similarity Parameters • The boundary layer equations (velocity, mass, energy continuity) represent low speed, forced convection flow. • Advection terms on the left side and diffusion terms on the right side of each equation, such as: Advection Diffusion • Non-dimensionalize the equations by setting: * s * 2 and / T T -T and where V is the freestream velocity ( ) where L is characteristic length of the surface P p V T T U V v and v V u u L y and y L x x s 2 2 y T y T v x T u

Boundary Layer Similarity Parameters(Cont'd) The boundary layer equations can be rewritten in terms of the non-dimensional variables Continuity ou,0* * =0 x-momentum aP O2u' +v Ox" ov" Ox L *2 a energy tv'o *dT* o2T u 元0y2 With boundary conditions Wall u(x,y=0)=0;v*(x,y=0)=0; T(x,y=0)=0 Frcestream:)U)if-U] T(x,y*=o)=1
Boundary Layer Similarity Parameters (Cont’d) • The boundary layer equations can be rewritten in terms of the non-dimensional variables Continuity x-momentum energy • With boundary conditions 0 * * * * y v x u 2 * 2 * * * * * * * * * y u x VL P y u v x u u 2 * 2 * * * * * * * y T y VL T v x T u ( , ) 1 [ 1if ] ( ) Freestream : ( , ) ( , 0) 0 Wall : ( , 0) 0 ; ( , 0) 0 ; * * * * * * * * * * * * * * * * T x y V U V U x u x y T x y u x y v x y

Boundary Layer Similarity Parameters(Cont'd) From the non-dimensionalized boundary layer equations,dimensionless groups can be seen Reynolds Prandtl Substituting gives the boundary layer equations: Continuity: ou'Ov" =0 * ou'ou ap* 1a2u* x-momentum: u +v Ox a" ReL oy Energy: aT,*∂T*1 02T dy" ReLPry2
Boundary Layer Similarity Parameters (Cont’d) • From the non-dimensionalized boundary layer equations, dimensionless groups can be seen Reynolds # Prandtl # Substituting gives the boundary layer equations: VL Re L 0 * * * * y v x u 2 * 2 * * * * * * * * * Re 1 y u x P y u v x u u L Re Pr 1 2 * 2 * * * * * * * y T y T v x T u L Continuity: x-momentum: Energy: P r
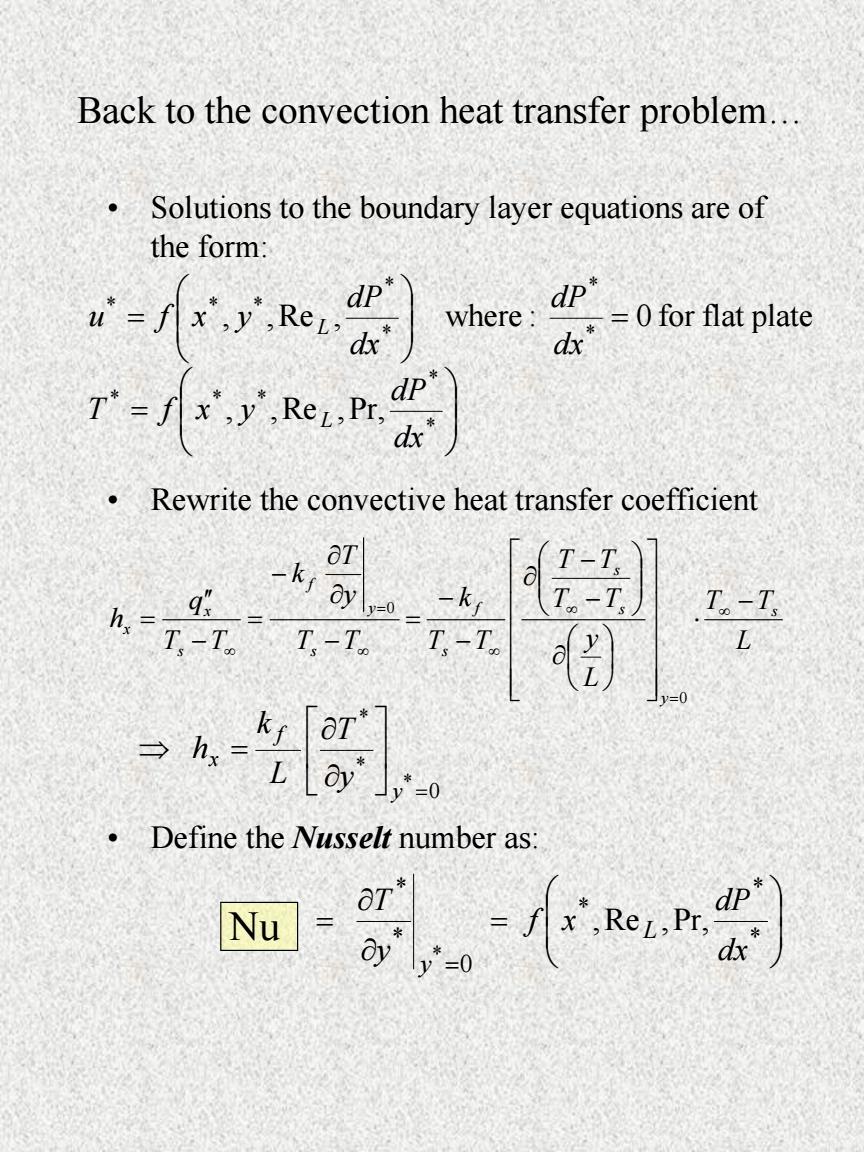
Back to the convection heat transfer problem... Solutions to the boundary layer equations are of the form: =,) dP" where: =0 for flat plate x* -小器 .Rewrite the convective heat transfer coefficient t 一k 6 T-T -ki h, 9 T。-I T,-T. T-T。 T-T. L kr 「a Define the Nusselt number as: Nu .o
Back to the convection heat transfer problem… • Solutions to the boundary layer equations are of the form: • Rewrite the convective heat transfer coefficient • Define the Nusselt number as: * * * * * * * * * * * * , ,Re ,Pr, , ,Re , where : 0 for flat plate dx dP T f x y dx dP dx dP u f x y L L L T T L y T T T T T T k T T y T k T T q h s y s s s f s y f s x x 0 0 0 * * * y f x y T L k h * * * 0 * * ,Re ,Pr, * dx dP f x y T L y Nu
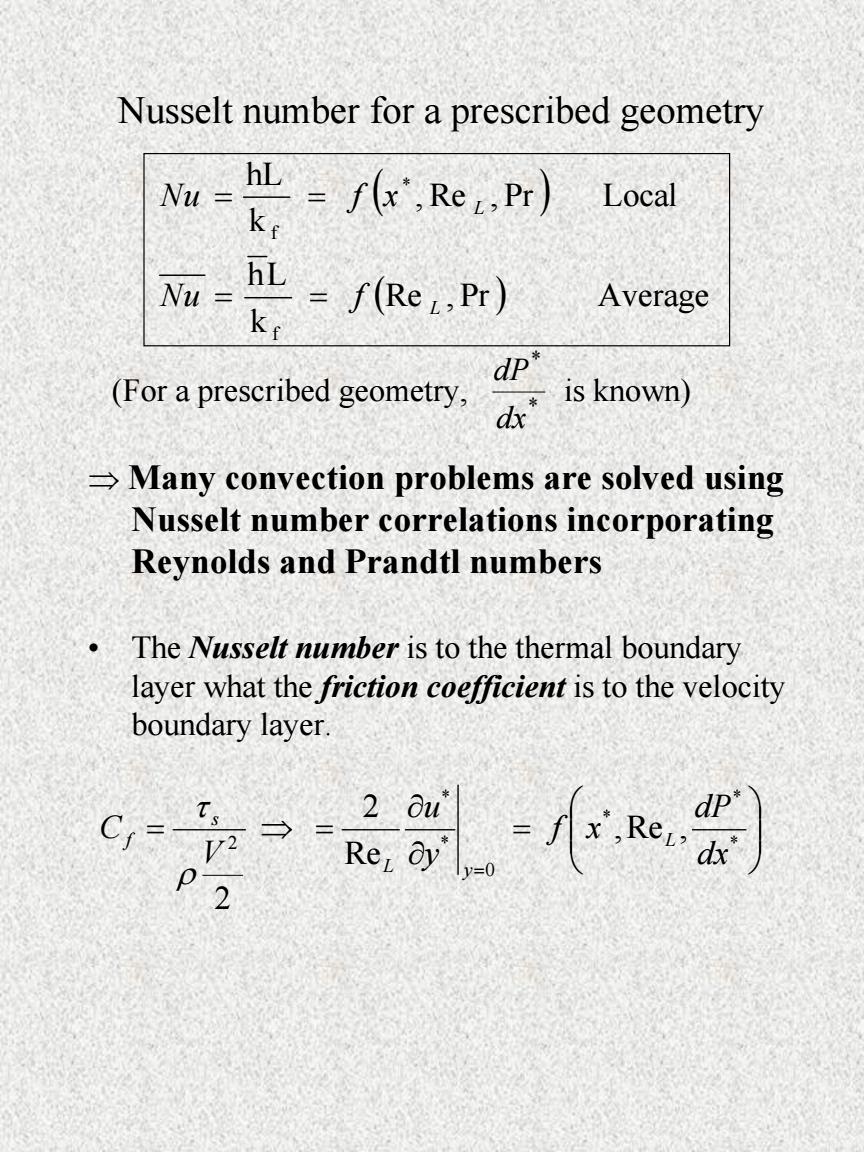
Nusselt number for a prescribed geometry Nu= -=f(x'.Rec.Pr) Local Nu= _=f(Re.Pr) Average dp" (For a prescribed geometry, is known) dx Many convection problems are solved using Nusselt number correlations incorporating Reynolds and Prandtl numbers The Nusselt number is to the thermal boundary layer what the friction coefficient is to the velocity boundary layer. CI= 2 ou v2 → Re,dy" =0 2
Nusselt number for a prescribed geometry (For a prescribed geometry, is known) Many convection problems are solved using Nusselt number correlations incorporating Reynolds and Prandtl numbers • The Nusselt number is to the thermal boundary layer what the friction coef icient is to the velocity boundary layer. Re , Pr Average k hL , Re , Pr Local k hL f * f L L Nu f Nu f x * * dx dP * * * 0 * * 2 ,Re , Re 2 2 dx dP f x y u V C L L y s f