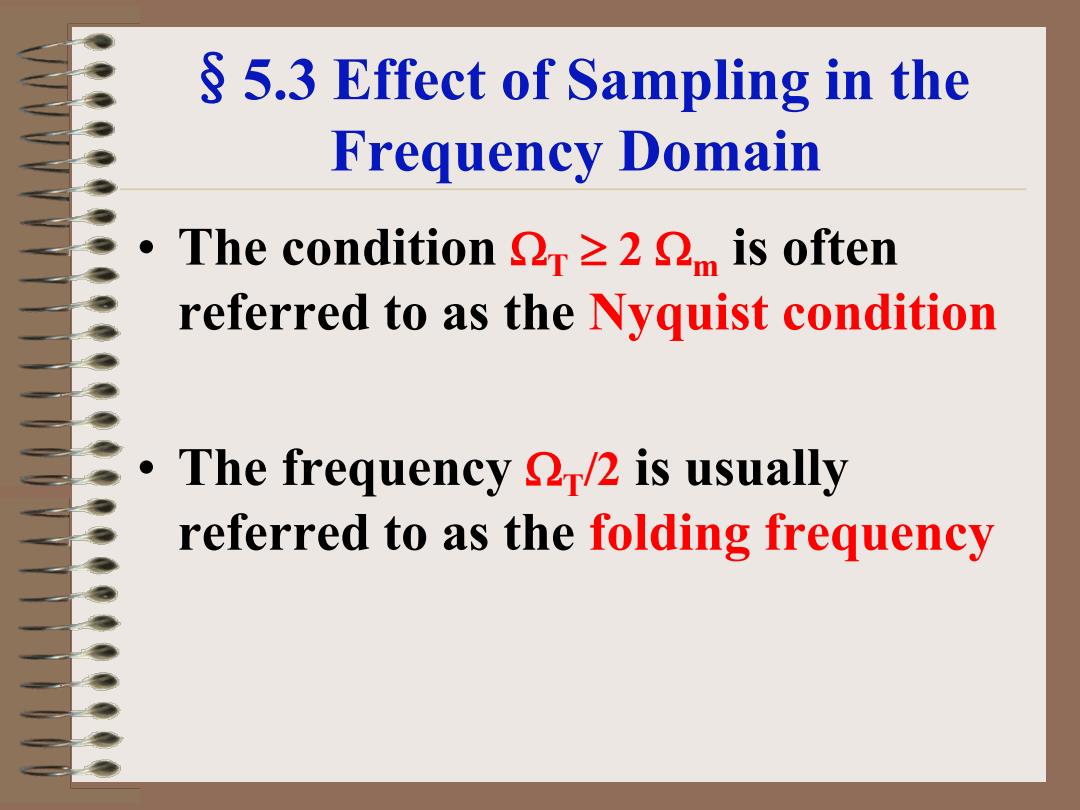
5.3 Effect of Sampling in the Frequency Domain The condition r>2 is often referred to as the Nyquist condition The frequency /2 is usually referred to as the folding frequency
§5.3 Effect of Sampling in the Frequency Domain • The condition ΩT ≥ 2 Ωm is often referred to as the Nyquist condition • The frequency ΩT/2 is usually referred to as the folding frequency
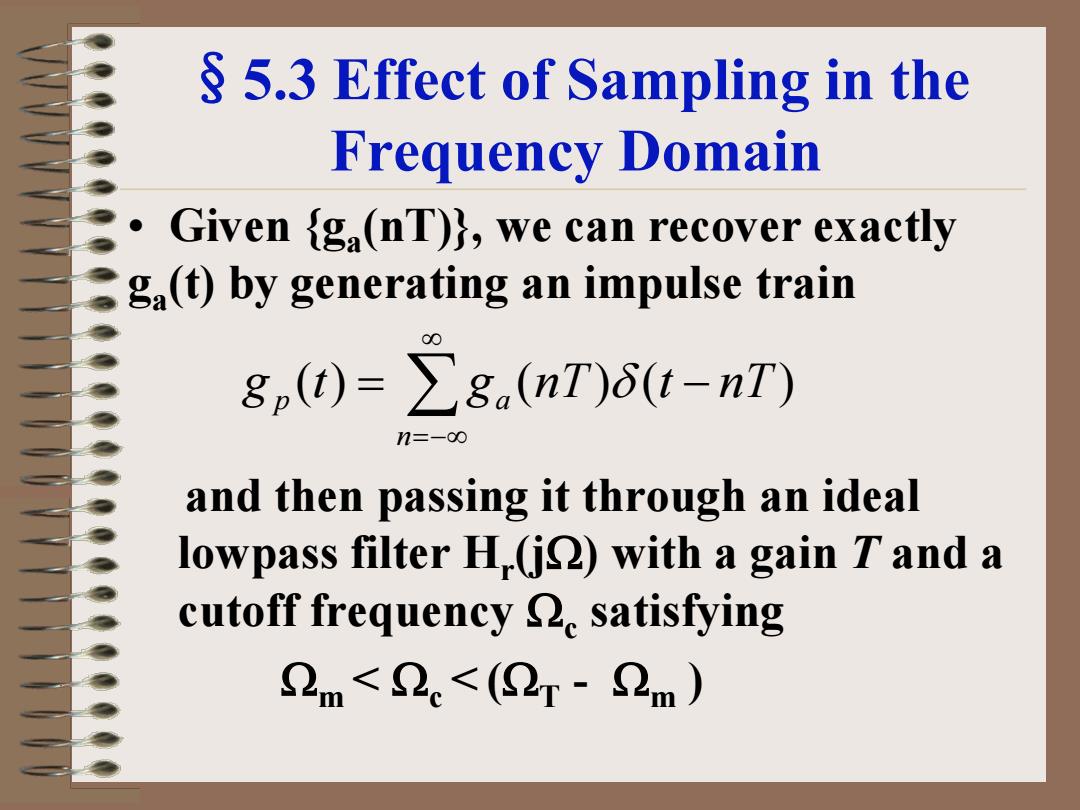
5.3 Effect of Sampling in the Frequency Domain Given {ga(nT)},we can recover exactly ga(t)by generating an impulse train 8,()=∑g(nT)6t-nT) n=-o0 and then passing it through an ideal lowpass filter H(j)with a gain T and a cutoff frequency satisfying 2m<2e<(2r-2m)
§5.3 Effect of Sampling in the Frequency Domain and then passing it through an ideal lowpass filter Hr(jΩ) with a gain T and a cutoff frequency Ωc satisfying Ωm < Ωc < (ΩT - Ωm ) ∑ ∞ =−∞ = − n g p (t) ga (nT)δ (t nT) • Given {ga(nT)}, we can recover exactly ga(t) by generating an impulse train
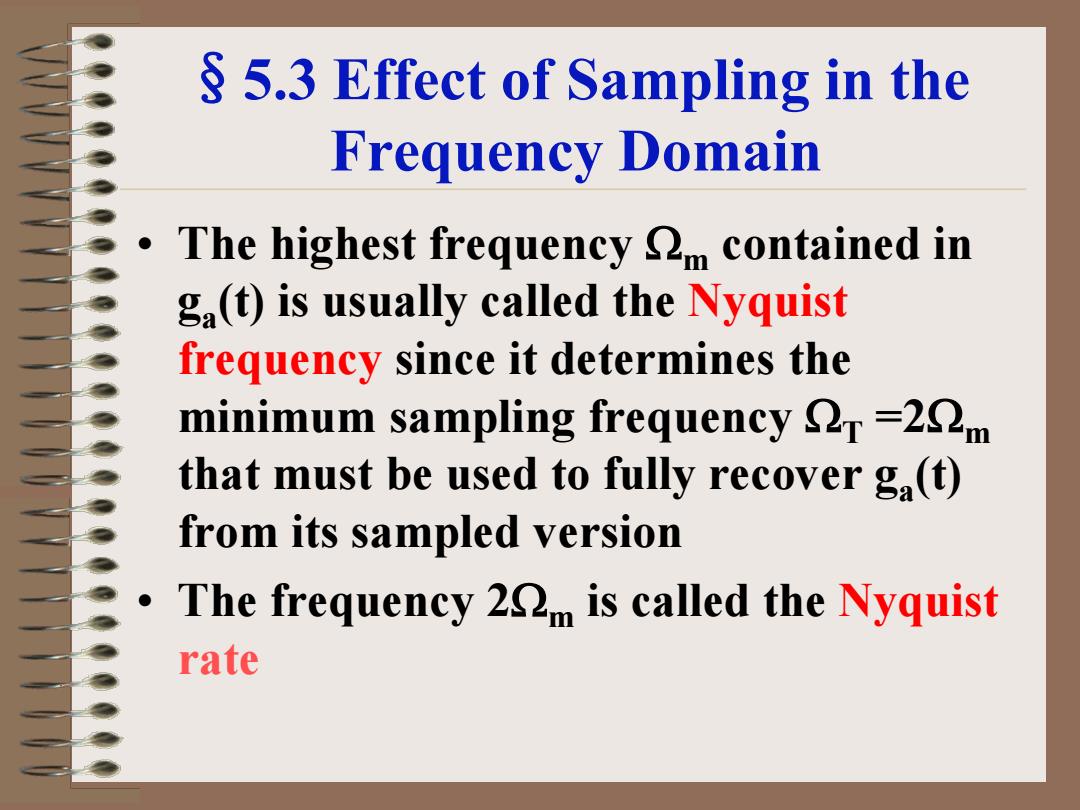
5.3 Effect of Sampling in the Frequency Domain The highest frequency m contained in g(t)is usually called the Nyquist frequency since it determines the minimum sampling frequency r =29m that must be used to fully recover ga(t) from its sampled version The frequency 21m is called the Nyquist rate
§5.3 Effect of Sampling in the Frequency Domain • The highest frequency Ωm contained in ga(t) is usually called the Nyquist frequency since it determines the minimum sampling frequency ΩT =2Ωm that must be used to fully recover ga(t) from its sampled version • The frequency 2Ωm is called the Nyquist rate