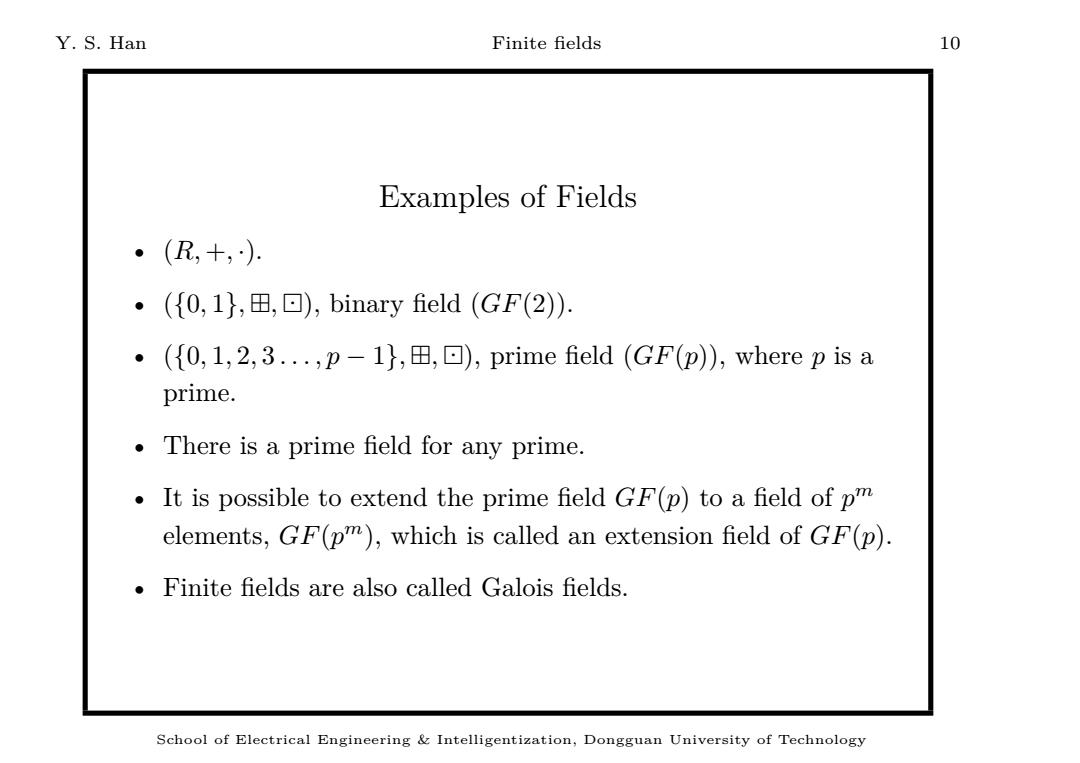
Y.S.Han Finite fields 10 Examples of Fields ·(R,+,) ·({0,1},田,回),binary field(GF(2). ·({0,l,2,3.,p-1},田,回),prime field(GF(p),where p is a prime. There is a prime field for any prime. It is possible to extend the prime field GF(p)to a field of pm elements,GF(pm),which is called an extension field of GF(p). Finite fields are also called Galois fields. School of Electrical Engineering Intelligentization,Dongguan University of Technology
Y. S. Han Finite fields 10 Examples of Fields • (R, +, ·). • ({0, 1}, ⊞, ⊡), binary field (GF(2)). • ({0, 1, 2, 3 . . . , p − 1}, ⊞, ⊡), prime field (GF(p)), where p is a prime. • There is a prime field for any prime. • It is possible to extend the prime field GF(p) to a field of p m elements, GF(p m), which is called an extension field of GF(p). • Finite fields are also called Galois fields. School of Electrical Engineering & Intelligentization, Dongguan University of Technology
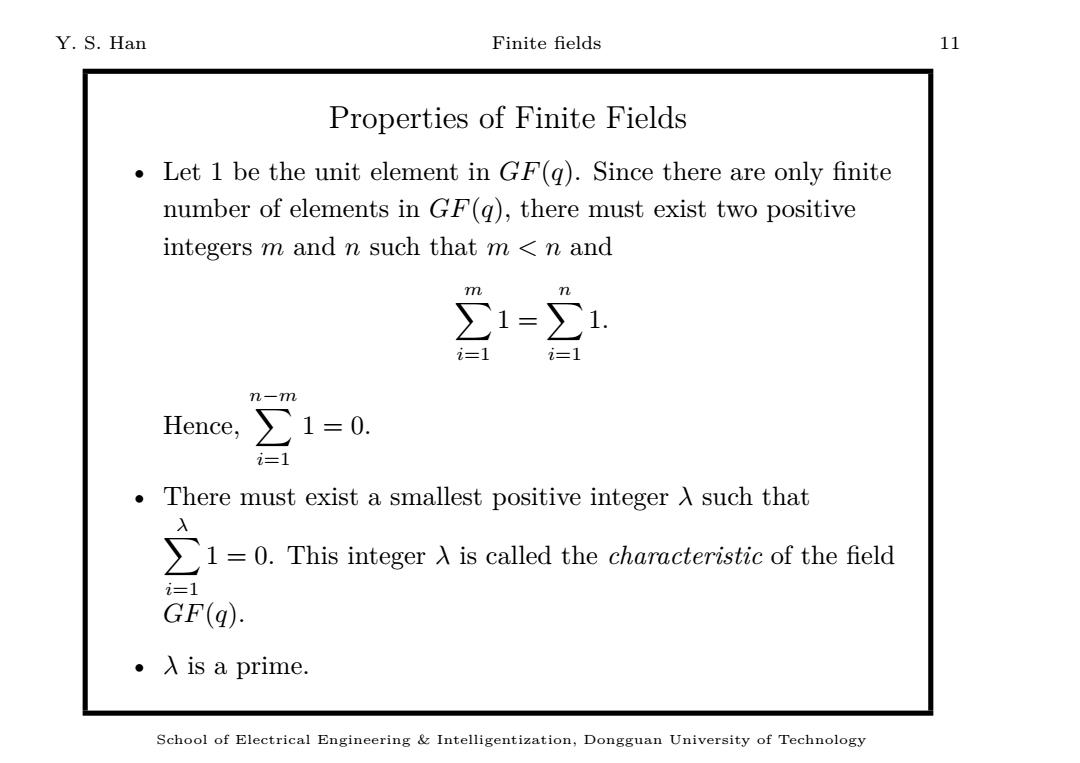
Y.S.Han Finite fields 11 Properties of Finite Fields Let 1 be the unit element in GF(g).Since there are only finite number of elements in GF(q),there must exist two positive integers m and n such that m <n and m n 21 n-m Hence, i-n There must exist a smallest positive integer A such that 10.This integeris called the cteristic of the field i=1 GF(q). 。λis a prime. School of Electrical Engineering Intelligentization,Dongguan University of Technology
Y. S. Han Finite fields 11 Properties of Finite Fields • Let 1 be the unit element in GF(q). Since there are only finite number of elements in GF(q), there must exist two positive integers m and n such that m < n and ∑ m i=1 1 = ∑ n i=1 1. Hence, n ∑−m i=1 1 = 0. • There must exist a smallest positive integer λ such that ∑ λ i=1 1 = 0. This integer λ is called the characteristic of the field GF(q). • λ is a prime. School of Electrical Engineering & Intelligentization, Dongguan University of Technology

Y.S.Han Finite fields 12 Proof:Assume that入=km,where1<k,m<入.Then ②)(②)-2= m n1=0or 1=0.Contradiction. Then i1 k m 1 2 λ-1 入 i=1i=1 =1=1 GF(q).It cab be proved that these A elements is a field, GF(A),under the addition and multiplication of GF(q). GF(A)is called a subfield of GF(q). 。Ifq≠入,then g is a power of入. School of Electrical Engineering Intelligentization,Dongguan University of Technology
Y. S. Han Finite fields 12 Proof: Assume that λ = km, where 1 < k, m < λ. Then ( ∑ k i=1 1 ) · ( ∑ m i=1 1 ) = ∑ km i=1 1 = 0. Then ∑ k i=1 1 = 0 or ∑ m i=1 1 = 0. Contradiction. • ∑ k i=1 1 ̸= ∑ m i=1 1 for any k, m < λ and k ̸= m. • 1 = ∑ 1 i=1 1, ∑ 2 i=1 1, . . . , λ ∑−1 i=1 1, ∑ λ i=1 1 = 0 are λ distinct elements in GF(q). It cab be proved that these λ elements is a field, GF(λ), under the addition and multiplication of GF(q). GF(λ) is called a subfield of GF(q). • If q ̸= λ, then q is a power of λ. School of Electrical Engineering & Intelligentization, Dongguan University of Technology
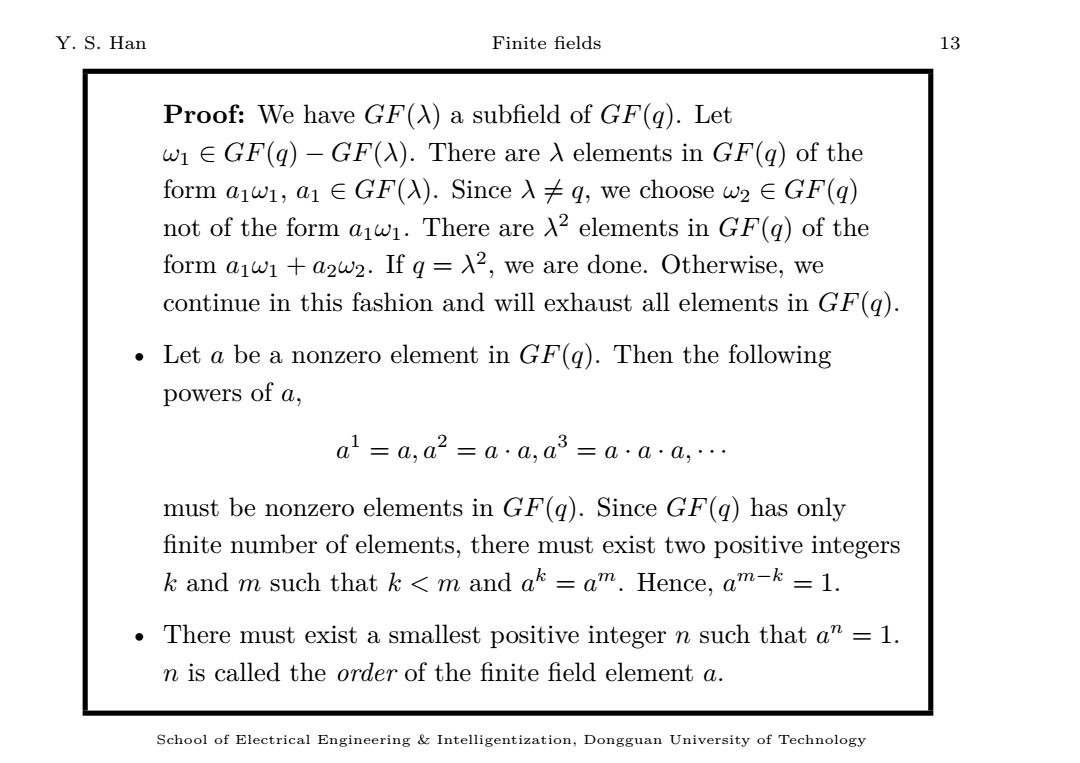
Y.S.Han Finite fields 13 Proof:We have GF(A)a subfield of GF(q).Let W1E GF(q)-GF(A).There are X elements in GF(q)of the form a1w1,a1∈GF().Since入≠g,we choose w2∈GF(q) not of the form awi.There are A2 elements in GF(q)of the form aw+a2w2.If q=A2,we are done.Otherwise,we continue in this fashion and will exhaust all elements in GF(q). Let a be a nonzero element in GF(q).Then the following powers of a, a1=a,a2=a…a,a3=a·aa, must be nonzero elements in GF(q).Since GF(q)has only finite number of elements,there must exist two positive integers k and m such that k <m and ak am.Hence,am-k =1. There must exist a smallest positive integer n such that a"=1. n is called the order of the finite field element a. School of Electrical Engineering Intelligentization,Dongguan University of Technology
Y. S. Han Finite fields 13 Proof: We have GF(λ) a subfield of GF(q). Let ω1 ∈ GF(q) − GF(λ). There are λ elements in GF(q) of the form a1 ω1, a1 ∈ GF(λ). Since λ ̸= q, we choose ω2 ∈ GF(q) not of the form a1 ω1. There are λ 2 elements in GF(q) of the form a1 ω1 + a2 ω2. If q = λ 2 , we are done. Otherwise, we continue in this fashion and will exhaust all elements in GF(q). • Let a be a nonzero element in GF(q). Then the following powers of a, a 1 = a, a2 = a · a, a3 = a · a · a, · · · must be nonzero elements in GF(q). Since GF(q) has only finite number of elements, there must exist two positive integers k and m such that k < m and a k = a m. Hence, a m−k = 1. • There must exist a smallest positive integer n such that a n = 1. n is called the order of the finite field element a. School of Electrical Engineering & Intelligentization, Dongguan University of Technology