
芝 force per unit length N. ·10 FI any kind. Illustration of decomposition of coupling effects in a general laminate loaded by a single leads to nonzero Bi. middle surface or in partitioned form as twisting and if A=0,pure stretching of Note:if Bu=B12=B16=0,no bending or middle surface Stretching of middle surface Shearing of : directions Bending along Ns=Alx+Aie,+A16Y+B1Kx+Biaky+Bickxy of xy plane Twisting behavior and the presence of 16 and 26 terms in the lamina stiffness or sarily related.Lamina shear coupling is a result of anisotropic material level and the laminate level,but the two types of coupling are not neces- It is now clear that there may be coupling effects at both the lamina would only include the fourth and fifth terms and no coupling terms of moment-curvature relationship for a homogeneous isotropic plate no coupling terms of any kind,whereas in figure 7.11,the corresponding homogeneous isotropic plate would only include the first two terms and figure 7.10 that the corresponding force-deformation relationship for a (7.41)is illustrated in figure 7.10 and figure 7.11,respectively.Note in The decomposition of typical force and moment terms in equations the condition that all By=0 and that asymmetry about the middle surface material property symmetry with respect to the middle surface leads to ing moment loading.It can be easily shown that laminate geometric and a laminate will also exhibit midplane stretching under bending and twist- laminate having nonzero B will bend or twist under in-plane loads.Such curvatures (K}and the moments [M]with the midplane strains (e).A coupling stiffness matrix [B]couples the in-plane forces (N)with the stiffness matrix [D]relates the moments (M)to the curvatures [k).The relates the in-plane forces (N)to the midplane strains (e),and the bending From equations(7.42),we can see that the extensional stiffness matrix [A] 平业 Principles of Composite Material Mechanics T) FIGURE 7.12 FIGURE 7.11 llustration of the difference between lamina level coupling and laminate level coupling in a general laminate loaded by a single force per unit length N. be summarized. (Q16=0 for 0or 90 lamina) coupling in off-axis lamina and Q is due to shear at lamina level since A16Y term due to coupling middle surface. arrangement of plies about due to nonsymmetrical present.By terms present as Q16 and (26 may not be lamina coupling terms such laminate level even though cause coupling at the B1K+B12K,terms Example:Expanding expression for N nature of the stiffness matrices for several special types of laminates will one specific laminate force-deformation equation.In the next section,the Figure 7.12 illustrates the two different types of coupling that appear in isotropic lamina properties lead to the condition A16=A26=D16=0. isotropic laminae if they are stacked in nonsymmetrical fashion,but the For example,it is possible for a laminate to have nonzero By even with laminate level is due to geometric and/or material property asymmetry with respect to the middle surface and is unrelated to material anisotropy. ina level also leads to coupling at the laminate level due to terms such as A16A26 Di6 and D26 On the other hand,the Birtype coupling at the compliance matrices(recall section 2.6).This type of coupling at the lam- Illustration of decomposition of coupling effects in a general laminate loaded by a single bending moment per unit length M Analysis of Laminates Conclusion:Major simplifications possible if By=0 shearing and if D6=0,pure bending Note:if Bi=B12=B16=0,no stretching or middle surface Stretching of middle surface Shearing of x and'y axes Bending along Mx=BIEx+B12+B16Y+Di1Kx+D12Ky+Di6K of xy plane Twisting
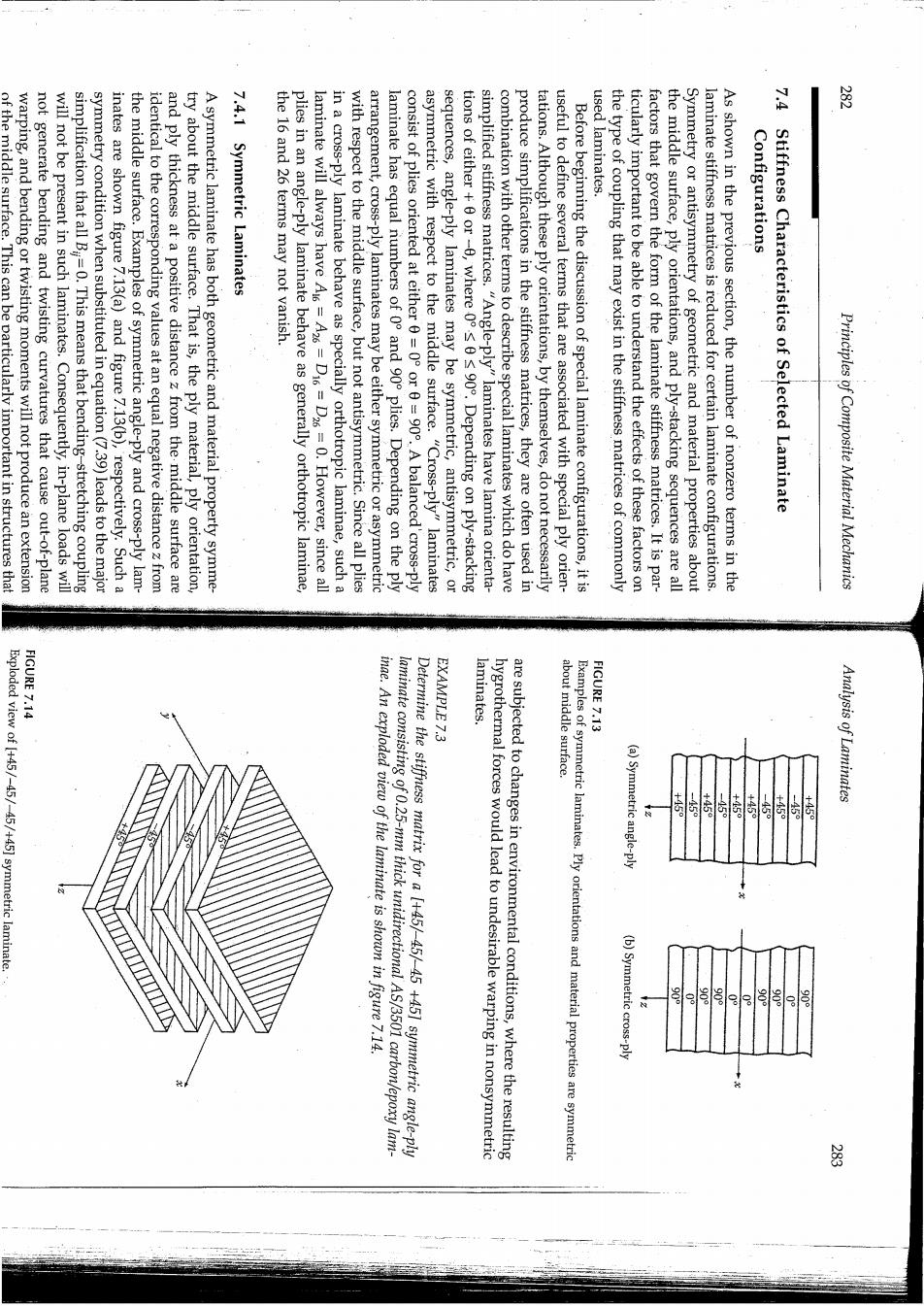
used laminates. 7.4 of the middle surface.This can be particularly important in structures that warping,and bending or twisting moments will not produce an extension not generate bending and twisting curvatures that cause out-of-plane will not be present in such laminates.Consequently,in-plane loads will simplification that all B=0.This means that bending-stretching coupling symmetry condition when substituted in equation(7.39)leads to the major inates are shown figure 7.13(a)and figure 7.13(b),respectively.Such a the middle surface.Examples of symmetric angle-ply and cross-ply lam- identical to the corresponding values at an equal negative distance z from and ply thickness at a positive distance z from the middle surface are try about the middle surface.That is,the ply material,ply orientation, A symmetric laminate has both geometric and material property symme- 7.4.1 Symmetric Laminates the 16 and 26 terms may not vanish. plies in an angle-ply laminate behave as generally orthotropic laminae, laminate will always have A=A26=Di6=D26=0.However,since all in a cross-ply laminate behave as specially orthotropic laminae,such a with respect to the middle surface,but not antisymmetric.Since all plies arrangement,cross-ply laminates may be either symmetric or asymmetric laminate has equal numbers of 0 and 90 plies.Depending on the ply consist of plies oriented at either 0=0 or 6=90.A balanced'cross-ply asymmetric with respect to the middle surface. "Cross-ply"laminates sequences,angle-ply laminates may be symmetric,antisymmetric,or tions of either+0 or-0,where 0s0s 90.Depending on ply-stacking simplified stiffness matrices."Angle-ply"laminates have lamina orienta- combination with other terms to describe special laminates which do have produce simplifications in the stiffness matrices,they are often used in tations.Although these ply orientations,by themselves,do not necessarily useful to define several terms that are associated with special ply orien- Before beginning the discussion of special laminate configurations,it is the type of coupling that may exist in the stiffness matrices of commonly ticularly important to be able to understand the effects of these factors on factors that govern the form of the laminate stiffness matrices.It is par- the middle surface,ply orientations,and ply-stacking sequences are all Symmetry or antisymmetry of geometric and material properties about laminate stiffness matrices is reduced for certain laminate configurations. As shown in the previous section,the number of nonzero terms in the Configurations Stiffness Characteristics of Selected Laminate Principles of Composite Material Mechanics FIGURE 7.14 laminates. Exploded view of +45/-45/-45/+45]symmetric laminate.. EXAMPLE 7.3 FIGURE 7.13 laminate consisting of0.25-mm thick unidirectional AS/3501 carbon/epoxy lam- inae.An exploded view of the laminate is showon in figure 7.14. Determine the stiffness matrix for a [+45/-45/-45 +45]symmetric angle-ply hygrothermal forces would lead to undesirable warping in nonsymmetric are subjected to changes in environmental conditions,where the resulting Examples of symmetric laminates.Ply orientations and material properties are symmetric about middle surface. (a)Symmetric angle-ply Analysis of Laminates +45 +458 46 (b)Symmetric cross-ply

M31.42 4523 452231.43 356 0 GPa-mm these distances along with the lamina stiffness in equations(7.38): For the-45 plies, =0.5 mm.The laminate extensional stiffnesses are then found by substituting The distances are z=-0.50 mm,=-0.25 mm,=0,=0.25 mm,and from the middle surface on the various ply interfaces according to figure 7.9. ply.Before calculating the laminate stiffnesses,we must determine distances the-45 ply have the opposite sign from the corresponding terms for+45 that the shear coupling terms(i.e.,the terms with subscripts 16 and 26)for Note that the only difference between the stiffness matrices for the plies is cipal material axes: [Q145*= -32.4 31.42 4522 4522 3143 060 138.82720 9 356 32.4432.44356 4522314282.44 equations (2.43)and equations(2.44).For the +45 plies, -32.4GPa 13生 31.42452232.44GPa then found by substituting the above stiffnesses in equations(2.36)or The transformed lamina stiffness matrices for the +45 and-45 plies are 12.729050lGa the components of the lamina stiffness matrix associated with the prin- Substitution of the above engineering constants in equations(2.27)yields E1=138 GPa E2=9 GPa G12=6.9GPa v12 =0.3 Solution.From table 2.2,the lamina engineering constants are Principles of Composite Material Mechanics FIGURE 7.15 Examples of antisymmetric angle-ply laminates.Although ply orientations are antisymmet- ric about middle surface,the material distribution is symmetric. 7.4.2 Antisymmetric Laminates Analysis of Laminates -5。 21032032.97 2.623772.03 877262209 100 000|GPa-mm2 00 曾 南 Bu=B12=B22=B66=0 for the antisymmetric angle-ply laminate. condition into equations(7.38)and equations(7.40),the coupling terms A1=A26=D16=D26=0.From equations (7.39),it can also be shown that has no meaning for a cross-ply laminate,which must be either symmetric or nonsymmetric.It can be shown that by substituting the antisymmetric an equal negative distance z is-0.Examples of antisymmetric angle-ply laminates are shown in figure 7.15.Note that the antisymmetric definition the ply orientation at a positive distance z is+0,if the ply orientation at orientations are antisymmetric with respect to the middle surface.That is, An antisymmetric laminate has plies of identical material and thickness at equal positive and negative distances from the middle surface,but the ply GPa-mm3 and the laminate-bending stiffnesses are found from equations(7.40): Similarly,the laminate-coupling stiffnesses are found from equation(7.39): 斜
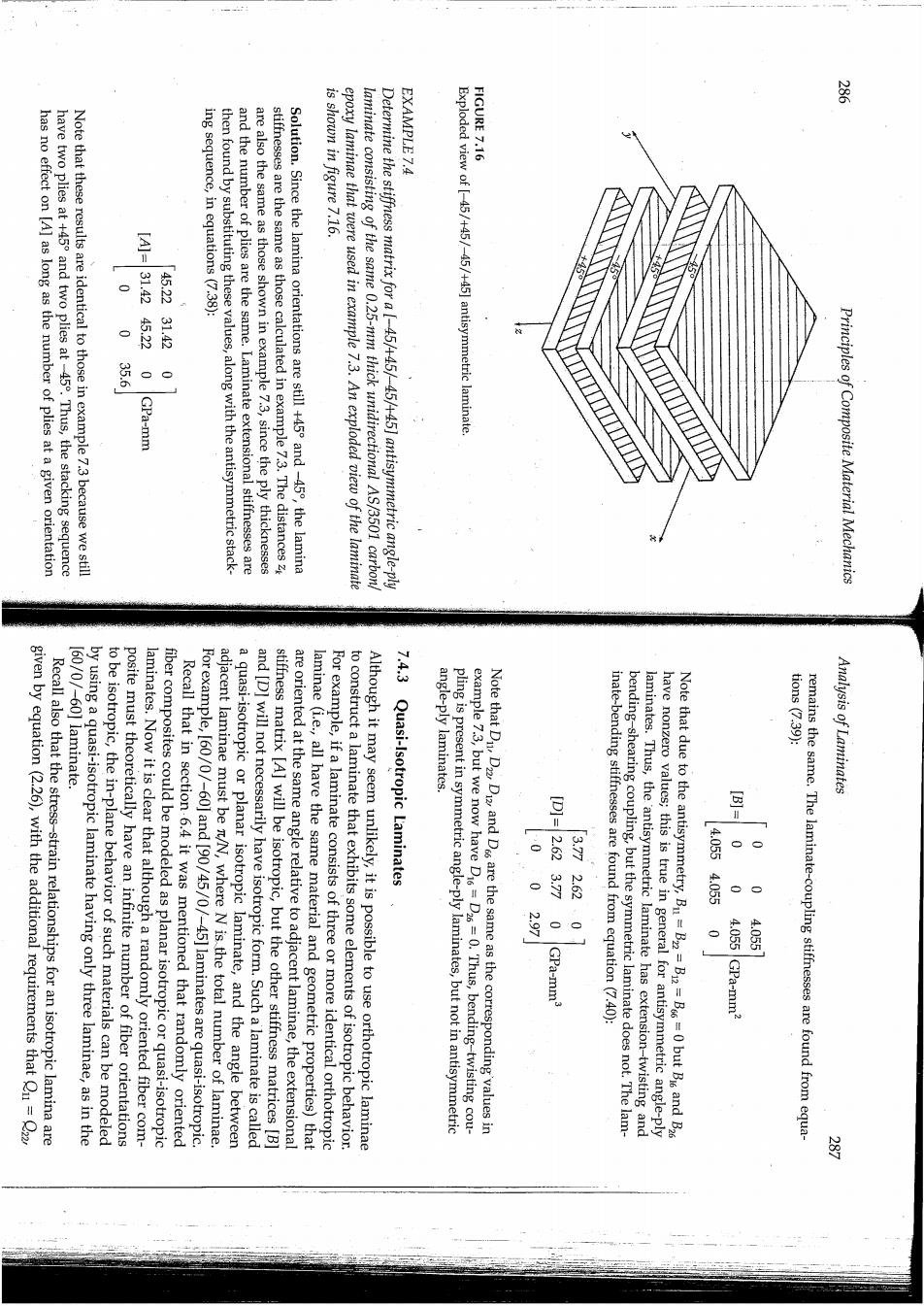
笑 ing sequence,in equations (7.38): is shown in figure 7.16. EXAMPLE 7.4 ·l6 F has no effect on [A]as long as the number of plies at a given orientation have two plies at+45 and two plies at-45.Thus,the stacking sequence Note that these results are identical to those in example 7.3 because we still [A]= 31.42 63 45223142 356 GPa-mm then found by substituting these values,along with the antisymmetric stack- and the number of plies are the same.Laminate extensional stiffnesses are are also the same as those shown in example 7.3,since the ply thicknesses stiffnesses are the same as those calculated in example 7.3.The distancesz Solution.Since the lamina orientations are still +45 and-45,the lamina epoxy laminae that were used in example 7.3.An exploded view of the laminate laminate consisting of the same 0.25-mm thick unidirectional AS/3501 carbon/ Determine the stiffness matrix for a [-45/445/45/45]antisymmetric angle-ply Exploded view of [-45/+45/-45/+45]antisymmetric laminate. Principles of Composite Material Mechanics 7.4.3 tions (7.39): given by equation(2.26),with the additional requirements that Qu=Q2z Recall also that the stress-strain relationships for an isotropic lamina are by using a quasi-isotropic laminate having only three laminae,as in the [60/0/-60]laminate to be isotropic,the in-plane behavior of such materials can be modeled posite must theoretically have an infinite number of fiber orientations fiber composites could be modeled as planar isotropic or quasi-isotropic laminates.Now it is clear that although a randomly oriented fiber com- adjacent laminae must be /N,where N is the total number of laminae. For example,[60/0/-60]and [90/45/0/-45]laminates are quasi-isotropic. Recall that in section 6.4 it was mentioned that randomly oriented a quasi-isotropic or planar isotropic laminate,and the angle between and [D]will not necessarily have isotropic form.Such a laminate is called are oriented at the same angle relative to adjacent laminae,the extensional stiffness matrix [A]will be isotropic,but the other stiffness matrices [B] laminae (i.e.,all have the same material and geometric properties)that For example,if a laminate consists of three or more identical orthotropic to construct a laminate that exhibits some elements of isotropic behavior. Although it may seem unlikely,it is possible to use orthotropic laminae Quasi-Isotropic Laminates pling is present in symmetric angle-ply laminates,but not in antisymmetric angle-ply laminates. example 7.3,but we now have Dis=D2s=0.Thus,bending-twisting cou- Note that Dn D Diz and D are the same as the corresponding'values in Analysis of Laminates 00 262377 3.77 2.62 2. inate-bending stiffnesses are found from equation(7.40): GPa-mm3 bending-shearing coupling,but the symmetric laminate does not.The lam- laminates.Thus,the antisymmetric laminate has extension-twisting and Note that due to the antisymmetry,Bu=B2=B12=B=0 but Bi and B26 have nonzero values;this is true in general for antisymmetric angle-ply 4:0554.055 B-0 4.055GPa-mm2 1055 remains the same.The laminate-coupling stiffnesses are found from equa- 苦

第 EXAMPLE 7.5 [A]= equations(7.38),we find that the laminate extensional stiffnesses are or equations(2.43)and equations (2.44).Substituting these stiffnesses in appropriate transformed stiffnesses for 60 and-60 from equations(2.36) Solution.The required lamina stiffnesses are the Qi in example 7.3 and the 7.3.Figure 7.17 shows an exploded view of the laminate. 160/0/-60]laminate consisting of the same laminae that were described in example Determine the stiffness matrices and engineering constants for a quasi-isotropic nates are given by equations(6.43). the effective extensional engineering constants for quasi-isotropic lami- Using developments similar to those in section 6.4,we can show that sional stiffnesses,we find that 12.8044.68 44.6812.80 15.94 i三Ar GPa-mm invariants from equations(2:43)in equations(7.38)for the laminate exten- laminates.For example,by substituting the lamina stiffnesses in terms of ite.Similarly,the invariants can be used in the study of quasi-isotropic neering constants of a planar isotropic,randomly oriented fiber compos- development of the stress-strain relationships and equations for the engi- In section 6.4,it was shown that the invariants could be useful in the isotropic laminates such as [60/0/-60]or [90/45/0/-45]. matrices,as can be shown by calculating the stiffness matrices for quasi- In general,such simplifications are not possible for the [B]and [D] 子 (An-A2)/2 mation relationships for the quasi-isotropic laminate are given by Q66=(Qu-Q12)/2,and Q=Q26=0.Similarly,the extensional force-defor- Principles of Composite Material Mechanics behavior only. FIGURE 7.17 Analysis of Laminates ::5957cpa ED话:/0.624288 B]= and ants.By substituting the Q from example 7.3 in equation(2.44),we find that The engineering constants for the laminate may be found by using the invari- 15/-45],[30/-30/-90],or [0/-60/-1201),the Ay remain unchanged but the Band Dy do not.Thus,the laminate is isotropic with respect to in-plane tions while maintaining equal angles between adjacent laminae (e.g.,[75/ equations(7.43).It can also be shown that by changing the lamina orienta- It is seen that the matrices [B]and [D]do not have the isotropic form of 0.8560.824 and the bending stiffnesses from equations(7.40)are -196-5060 It is easily shown that this matrix is of the isotropic form shown in equations (7.43).The laminate-coupling stiffnesses from equations (7.39)are Exploded view of[+60/0/-60]quasi-isotropic laminate. 2.002 GPa-mm -5.06GPa-mm2